Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<strong>Answers</strong> to <strong>Exercises</strong> 173<br />
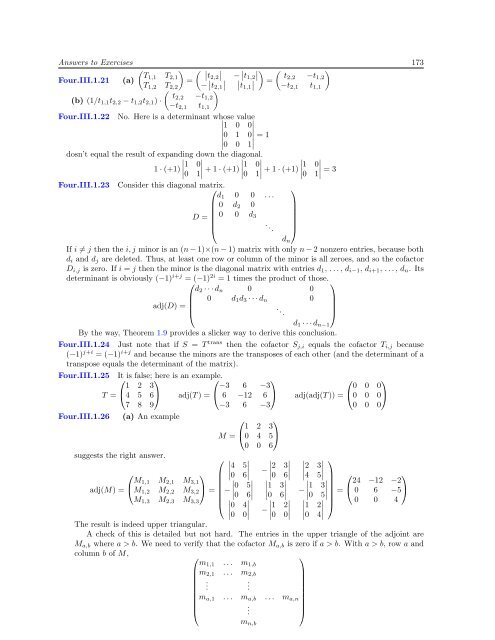
( ) ( ∣ ∣<br />
T1,1 T<br />
Four.III.1.21 (a)<br />
2,1<br />
t<br />
= 2,2 − ∣ ∣)<br />
( )<br />
∣t 1,2 T 1,2 T 2,2 − ∣ ∣ ∣ ∣ t2,2 −t<br />
∣t 2,1 t 1,1 =<br />
1,2<br />
−t 2,1 t<br />
( )<br />
1,1<br />
t2,2 −t<br />
(b) (1/t 1,1 t 2,2 − t 1,2 t 2,1 ) ·<br />
1,2<br />
−t 2,1 t 1,1<br />
Four.III.1.22 No. Here is a determinant whose value<br />
1 0 0<br />
0 1 0<br />
∣0 0 1∣ = 1<br />
dosn’t equal the result of expanding down the diagonal.<br />
1 · (+1)<br />
∣ 1 0<br />
∣ ∣ ∣∣∣ 0 1∣ + 1 · (+1) 1 0<br />
∣∣∣ 0 1∣ + 1 · (+1) 1 0<br />
0 1∣ = 3<br />
Four.III.1.23 Consider this diagonal matrix.<br />
⎛<br />
⎞<br />
d 1 0 0 . . .<br />
0 d 2 0<br />
D =<br />
0 0 d 3 ⎜<br />
⎝<br />
. ..<br />
⎟<br />
⎠<br />
d n<br />
If i ≠ j then the i, j minor is an (n − 1)×(n − 1) matrix with only n − 2 nonzero entries, because both<br />
d i and d j are deleted. Thus, at least one row or column of the minor is all zeroes, and so the cofactor<br />
D i,j is zero. If i = j then the minor is the diagonal matrix with entries d 1 , . . . , d i−1 , d i+1 , . . . , d n . Its<br />
determinant is obviously (−1) i+j = (−1) 2i = 1 times the product of those.<br />
⎛<br />
⎞<br />
d 2 · · · d n 0 0<br />
0 d 1 d 3 · · · d n 0<br />
adj(D) = ⎜<br />
⎝<br />
. ..<br />
⎟<br />
⎠<br />
d 1 · · · d n−1<br />
By the way, Theorem 1.9 provides a slicker way to derive this conclusion.<br />
Four.III.1.24 Just note that if S = T trans then the cofactor S j,i equals the cofactor T i,j because<br />
(−1) j+i = (−1) i+j and because the minors are the transposes of each other (and the determinant of a<br />
transpose equals the determinant of the matrix).<br />
Four.III.1.25 It<br />
⎛<br />
is false; here is an example.<br />
T = ⎝ 1 2 3<br />
⎞<br />
⎛<br />
⎞<br />
⎛<br />
−3 6 −3<br />
4 5 6⎠ adj(T ) = ⎝ 6 −12 6 ⎠ adj(adj(T )) = ⎝ 0 0 0<br />
⎞<br />
0 0 0⎠<br />
7 8 9<br />
−3 6 −3<br />
0 0 0<br />
Four.III.1.26 (a) An example<br />
⎛<br />
M = ⎝ 1 2 3<br />
⎞<br />
0 4 5⎠<br />
0 0 6<br />
suggests the right answer.<br />
⎛<br />
⎛<br />
adj(M) = ⎝ M ⎞<br />
∣ 4 5<br />
1,1 M 2,1 M 3,1<br />
0 6∣<br />
−<br />
∣ 2 3<br />
0 6∣<br />
∣ 2 3<br />
⎞<br />
4 5∣<br />
⎛<br />
⎞<br />
M 1,2 M 2,2 M 3,2<br />
⎠ =<br />
−<br />
∣ 0 5<br />
M 1,3 M 2,3 M 3,3<br />
⎜ 0 6∣<br />
∣ 1 3<br />
0 6∣<br />
−<br />
∣ 1 3<br />
24 −12 −2<br />
0 5∣<br />
= ⎝ 0 6 −5⎠<br />
⎝<br />
∣ 0 4<br />
0 0∣<br />
−<br />
∣ 1 2<br />
0 0∣<br />
∣ 1 2<br />
⎟<br />
⎠ 0 0 4<br />
0 4∣<br />
The result is indeed upper triangular.<br />
A check of this is detailed but not hard. The entries in the upper triangle of the adjoint are<br />
M a,b where a > b. We need to verify that the cofactor M a,b is zero if a > b. With a > b, row a and<br />
column b of M,<br />
⎛<br />
⎞<br />
m 1,1 . . . m 1,b<br />
m 2,1 . . . m 2,b<br />
.<br />
.<br />
m a,1 . . . m a,b . . . m a,n<br />
⎜<br />
.<br />
⎟<br />
⎝<br />
.<br />
⎠<br />
m n,b