Linear Algebra Exercises-n-Answers.pdf

170 Linear Algebra, by Hefferon
Four.II.1.24 Any permutation matrix has the property that the transpose of the matrix is its inverse.
For the implication, we know that |A trans | = |A|. Then 1 = |A · A −1 | = |A · A trans | = |A| · |A trans | =
|A| 2 .
The converse does not hold; here is an example.
( ) 3 1
2 1
Four.II.1.25
of volume.
Four.II.1.26
Where the sides of the box are c times longer, the box has c 3 times as many cubic units
If H = P −1 GP then |H| = |P −1 ||G||P | = |P −1 ||P ||G| = |P −1 P ||G| = |G|.
Four.II.1.27 ( ) ((a) ) The new ( basis ( is the old basis rotated by π/4.
−1 0 0 1
(b) 〈 , 〉, 〈 , 〉
0 −1 −1)
0)
(c) In each case the determinant is +1 (these bases are said to have positive orientation).
(d) Because only one sign can change at a time, the only other cycle possible is
( ( ( (
+ + − −
· · · −→ −→ −→ −→ −→ · · · .
+)
−)
−)
+)
Here each associated determinant is −1 (such bases are said to have a negative orientation).
(e) There is one positively oriented basis 〈(1)〉 and one negatively oriented basis 〈(−1)〉.
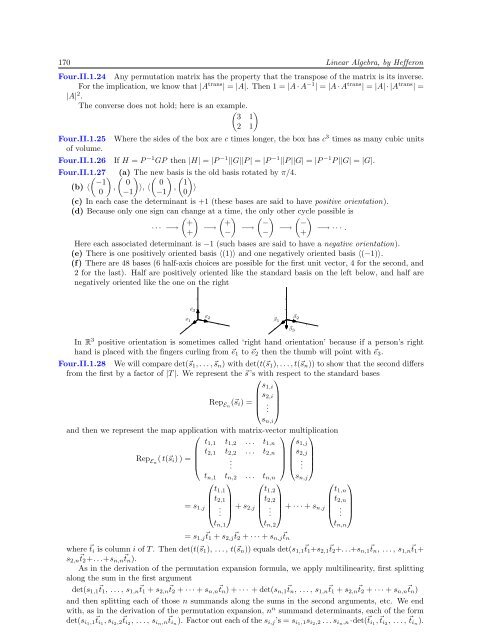
(f) There are 48 bases (6 half-axis choices are possible for the first unit vector, 4 for the second, and
2 for the last). Half are positively oriented like the standard basis on the left below, and half are
negatively oriented like the one on the right
⃗e 3
⃗e
⃗e 2
⃗ β2 ⃗
1 β1
In R 3 positive orientation is sometimes called ‘right hand orientation’ because if a person’s right
hand is placed with the fingers curling from ⃗e 1 to ⃗e 2 then the thumb will point with ⃗e 3 .
Four.II.1.28 We will compare det(⃗s 1 , . . . , ⃗s n ) with det(t(⃗s 1 ), . . . , t(⃗s n )) to show that the second differs
from the first by a factor of |T |. We represent the ⃗s ’s with respect to the standard bases
⎛ ⎞
s 1,i
s 2,i
Rep En
(⃗s i ) = ⎜
⎝
⎟
. ⎠
s n,i
and then we represent the map application with matrix-vector multiplication
⎛
⎞ ⎛ ⎞
t 1,1 t 1,2 . . . t 1,n s 1,j
t 2,1 t 2,2 . . . t 2,n
s 2,j
Rep En
( t(⃗s i ) ) = ⎜
⎟ ⎜ ⎟
⎝ .
⎠ ⎝ . ⎠
t n,1 t n,2 . . . t n,n s n,j
⎛ ⎞ ⎛ ⎞
⎛ ⎞
t 1,1 t 1,2
t 1,n
t 2,1
= s 1,j ⎜ ⎟
⎝ . ⎠ + s t 2,2
2,j ⎜ ⎟
⎝ . ⎠ + · · · + s t 2,n
n,j ⎜ ⎟
⎝ . ⎠
t n,1 t n,2 t n,n
= s 1,j
⃗t 1 + s 2,j
⃗t 2 + · · · + s n,j
⃗t n
where ⃗t i is column i of T . Then det(t(⃗s 1 ), . . . , t(⃗s n )) equals det(s 1,1
⃗t 1 +s 2,1
⃗t 2 +. . .+s n,1
⃗t n , . . . , s 1,n
⃗t 1 +
s 2,n
⃗t 2 +. . .+s n,n
⃗t n ).
As in the derivation of the permutation expansion formula, we apply multilinearity, first splitting
along the sum in the first argument
det(s 1,1
⃗t 1 , . . . , s 1,n
⃗t 1 + s 2,n
⃗t 2 + · · · + s n,n
⃗t n ) + · · · + det(s n,1
⃗t n , . . . , s 1,n
⃗t 1 + s 2,n
⃗t 2 + · · · + s n,n
⃗t n )
and then splitting each of those n summands along the sums in the second arguments, etc. We end
with, as in the derivation of the permutation expansion, n n summand determinants, each of the form
det(s i1 ,1 ⃗ t i1 , s i2 ,2 ⃗ t i2 , . . . , s in ,n ⃗ t in ). Factor out each of the s i,j ’s = s i1 ,1s i2 ,2 . . . s in ,n ·det(⃗t i1 ,⃗t i2 , . . . , ⃗t in ).
⃗β 3