Linear Algebra Exercises-n-Answers.pdf

Answers to Exercises 169
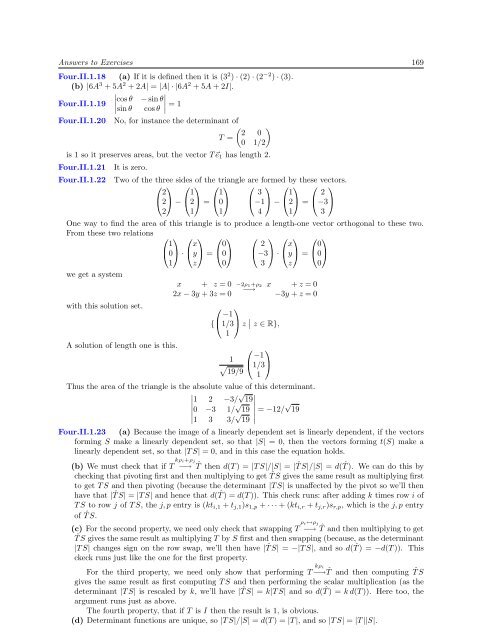
Four.II.1.18 (a) If it is defined then it is (3 2 ) · (2) · (2 −2 ) · (3).
(b) |6A 3 + 5A 2 + 2A| = |A| · |6A 2 + 5A + 2I|.
Four.II.1.19
∣ cos θ − sin θ
sin θ cos θ ∣ = 1
Four.II.1.20
No, for instance the determinant of
( )
2 0
T =
0 1/2
is 1 so it preserves areas, but the vector T⃗e 1 has length 2.
Four.II.1.21
Four.II.1.22
It is zero.
Two of the three sides of the triangle are formed by these vectors.
⎛ ⎞ ⎛ ⎞ ⎛ ⎞ ⎛
⎠ −
⎠ ⎝ 3
⎞ ⎛ ⎞ ⎛ ⎞
⎠ − ⎠ = ⎠
⎝ 2 2
2
⎝ 1 2⎠ =
1
⎝ 1 0
1
One way to find the area of this triangle is to produce a length-one vector orthogonal to these two.
From these two relations
⎛ ⎞ ⎛ ⎞ ⎛ ⎞ ⎛ ⎞ ⎛ ⎞ ⎛ ⎞
we get a system
with this solution set.
⎝ 1 0
1
A solution of length one is this.
⎠ ·
⎝ x y⎠ =
z
⎝ 0 0
0
⎠
x + z = 0
2x − 3y + 3z = 0
−1
4
⎝ 2 −3
3
⎠ ·
⎝ 1 2
1
⎝ x y⎠ =
z
⎝ 2 −3
3
⎝ 0 0
0
−2ρ 1 +ρ 2
−→
x + z = 0
−3y + z = 0
⎛
{ ⎝ −1
⎞
1/3⎠ z ∣ z ∈ R},
1
⎛
1
√ ⎝ −1
⎞
1/3⎠
19/9 1
Thus the area of the triangle is the absolute value of this determinant.
1 2 −3/ √ 19
0 −3 1/ √ 19
∣1 3 3/ √ 19 ∣ = −12/√ 19
Four.II.1.23 (a) Because the image of a linearly dependent set is linearly dependent, if the vectors
forming S make a linearly dependent set, so that |S| = 0, then the vectors forming t(S) make a
linearly dependent set, so that |T S| = 0, and in this case the equation holds.
(b) We must check that if T kρ i+ρ j
−→ ˆT then d(T ) = |T S|/|S| = | ˆT S|/|S| = d( ˆT ). We can do this by
checking that pivoting first and then multiplying to get ˆT S gives the same result as multiplying first
to get T S and then pivoting (because the determinant |T S| is unaffected by the pivot so we’ll then
have that | ˆT S| = |T S| and hence that d( ˆT ) = d(T )). This check runs: after adding k times row i of
T S to row j of T S, the j, p entry is (kt i,1 + t j,1 )s 1,p + · · · + (kt i,r + t j,r )s r,p , which is the j, p entry
of ˆT S.
(c) For the second property, we need only check that swapping T ρ i↔ρ j
−→ ˆT and then multiplying to get
ˆT S gives the same result as multiplying T by S first and then swapping (because, as the determinant
|T S| changes sign on the row swap, we’ll then have | ˆT S| = −|T S|, and so d( ˆT ) = −d(T )). This
ckeck runs just like the one for the first property.
For the third property, we need only show that performing T kρi
−→ ˆT and then computing ˆT S
gives the same result as first computing T S and then performing the scalar multiplication (as the
determinant |T S| is rescaled by k, we’ll have | ˆT S| = k|T S| and so d( ˆT ) = k d(T )). Here too, the
argument runs just as above.
The fourth property, that if T is I then the result is 1, is obvious.
(d) Determinant functions are unique, so |T S|/|S| = d(T ) = |T |, and so |T S| = |T ||S|.
⎠