Linear Algebra Exercises-n-Answers.pdf

138 Linear Algebra, by Hefferon
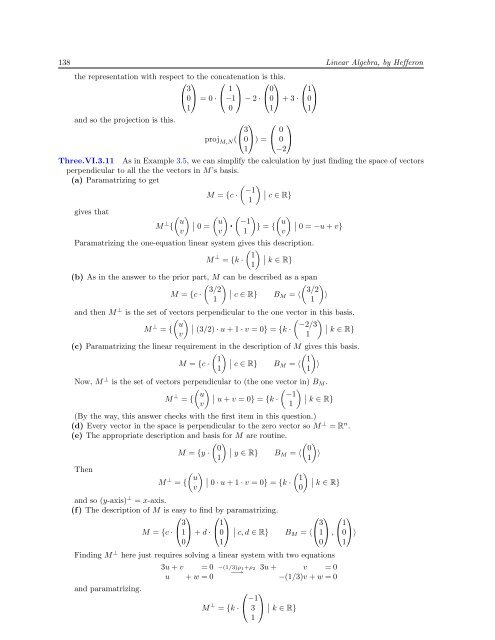
the representation with respect to the concatenation is this.
⎛ ⎞ ⎛ ⎞ ⎛ ⎞
and so the projection is this.
⎝ 3 0⎠ = 0 · ⎝ 1 −1⎠ − 2 · ⎝ 0 0⎠ + 3 · ⎝ 1 0⎠
1 0 1 1
⎛
⎞
proj M,N ( ⎝ 3 0⎠) =
1
⎛
⎝ 0 0
−2
Three.VI.3.11 As in Example 3.5, we can simplify the calculation by just finding the space of vectors
perpendicular to all the the vectors in M’s basis.
(a) Paramatrizing to get
( )
−1 ∣∣
M = {c · c ∈ R}
1
gives that
( ) ( ) ( ) ( )
M ⊥ u ∣∣ u −1 u ∣∣
{ 0 =
} = { 0 = −u + v}
v v 1 v
Paramatrizing the one-equation linear system gives this description.
( )
M ⊥ 1 ∣∣
= {k · k ∈ R}
1
(b) As in the answer to the prior part, M can be described as a span
( ) ( )
3/2 ∣∣ 3/2
M = {c · c ∈ R} B
1
M = 〈 〉
1
and then M ⊥ is the set of vectors perpendicular to the one vector in this basis.
( ) ( )
M ⊥ u ∣∣ −2/3 ∣∣
= { (3/2) · u + 1 · v = 0} = {k · k ∈ R}
v
1
(c) Paramatrizing the linear requirement in the description of M gives this basis.
( ) (
1 ∣∣ 1
M = {c · c ∈ R} B
1
M = 〈 〉
1)
Now, M ⊥ is the set of vectors perpendicular to (the one vector in) B M .
( ) ( )
M ⊥ u ∣∣ −1 ∣∣
= { u + v = 0} = {k · k ∈ R}
v
1
(By the way, this answer checks with the first item in this question.)
(d) Every vector in the space is perpendicular to the zero vector so M ⊥ = R n .
(e) The appropriate description and basis for M are routine.
( ) ( 0 ∣∣ 0
M = {y · y ∈ R} B
1
M = 〈 〉
1)
⎞
⎠
⎛
⎞
Then
( ) ( )
M ⊥ u ∣∣ 1 ∣∣
= { 0 · u + 1 · v = 0} = {k · k ∈ R}
v
0
and so (y-axis) ⊥ = x-axis.
(f) The description of M is easy to find by paramatrizing.
⎛ ⎞ ⎛ ⎞
⎛ ⎞ ⎛ ⎞
3 1
M = {c · ⎝1⎠ + d · ⎝0⎠ ∣ 3 1
c, d ∈ R} B M = 〈 ⎝1⎠ , ⎝0⎠〉
0 1
0 1
Finding M ⊥ here just requires solving a linear system with two equations
and paramatrizing.
3u + v = 0
u + w = 0
−(1/3)ρ 1+ρ 2
−→
3u + v = 0
−(1/3)v + w = 0
⎛
M ⊥ = {k · ⎝ −1
⎞
3 ⎠ ∣ k ∈ R}
1