Linear Algebra Exercises-n-Answers.pdf

and that (⃗v
− (c1 · ⃗κ 1 + · · · + c k · ⃗κ k ) ) ( (c 1 · ⃗κ 1 + · · · + c k · ⃗κ k ) − (d 1 · ⃗κ 1 + · · · + d k · ⃗κ k ) ) = 0
134 Linear Algebra, by Hefferon
This first thing is not so bad because the zero vector is by definition orthogonal to every other vector, so
we could accept this situation as yielding an orthogonal set (although it of course can’t be normalized),
or we just could modify the Gram-Schmidt procedure to throw out any zero vectors. The second thing
to worry about if we drop the phrase ‘linearly independent’ from the question is that the set might be
infinite. Of course, any subspace of the finite-dimensional R n must also be finite-dimensional so only
finitely many of its members are linearly independent, but nonetheless, a “process” that examines the
vectors in an infinite set one at a time would at least require some more elaboration in this question.
A linearly independent subset of R n is automatically finite — in fact, of size n or less — so the ‘linearly
independent’ phrase obviates these concerns.
Three.VI.2.14
The process leaves the basis unchanged.
Three.VI.2.15 (a) The argument is as in the i = 3 case of the proof of Theorem 2.7. The dot
product
)
⃗κ i
(⃗v − proj [⃗κ1 ](⃗v ) − · · · − proj [⃗vk ](⃗v )
can be written as the sum of terms of the form −⃗κ i proj [⃗κj ](⃗v ) with j ≠ i, and the term ⃗κ i (⃗v −
proj [⃗κi ](⃗v )). The first kind of term equals zero because the ⃗κ’s are mutually orthogonal. The other
term is zero because this projection is orthogonal (that is, the projection definition makes it zero:
⃗κ i (⃗v − proj [⃗κi](⃗v )) = ⃗κ i ⃗v − ⃗κ i ((⃗v ⃗κ i )/(⃗κ i ⃗κ i )) · ⃗κ i equals, after all of the cancellation is done,
zero).
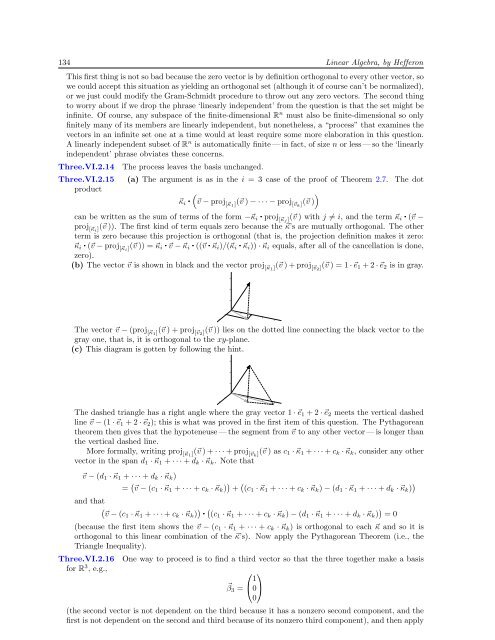
(b) The vector ⃗v is shown in black and the vector proj [⃗κ1 ](⃗v ) + proj [⃗v2 ](⃗v ) = 1 · ⃗e 1 + 2 · ⃗e 2 is in gray.
The vector ⃗v − (proj [⃗κ1 ](⃗v ) + proj [⃗v2 ](⃗v )) lies on the dotted line connecting the black vector to the
gray one, that is, it is orthogonal to the xy-plane.
(c) This diagram is gotten by following the hint.
The dashed triangle has a right angle where the gray vector 1 · ⃗e 1 + 2 · ⃗e 2 meets the vertical dashed
line ⃗v − (1 · ⃗e 1 + 2 · ⃗e 2 ); this is what was proved in the first item of this question. The Pythagorean
theorem then gives that the hypoteneuse — the segment from ⃗v to any other vector — is longer than
the vertical dashed line.
More formally, writing proj [⃗κ1 ](⃗v ) + · · · + proj [⃗vk ](⃗v ) as c 1 · ⃗κ 1 + · · · + c k · ⃗κ k , consider any other
vector in the span d 1 · ⃗κ 1 + · · · + d k · ⃗κ k . Note that
⃗v − (d 1 · ⃗κ 1 + · · · + d k · ⃗κ k )
= ( ⃗v − (c 1 · ⃗κ 1 + · · · + c k · ⃗κ k ) ) + ( (c 1 · ⃗κ 1 + · · · + c k · ⃗κ k ) − (d 1 · ⃗κ 1 + · · · + d k · ⃗κ k ) )
(because the first item shows the ⃗v − (c 1 · ⃗κ 1 + · · · + c k · ⃗κ k ) is orthogonal to each ⃗κ and so it is
orthogonal to this linear combination of the ⃗κ’s). Now apply the Pythagorean Theorem (i.e., the
Triangle Inequality).
Three.VI.2.16
for R 3 , e.g.,
One way to proceed is to find a third vector so that the three together make a basis
⎛ ⎞
1
⃗β 3 = ⎝0⎠
0
(the second vector is not dependent on the third because it has a nonzero second component, and the
first is not dependent on the second and third because of its nonzero third component), and then apply