Linear Algebra Exercises-n-Answers.pdf

Answers to Exercises 133
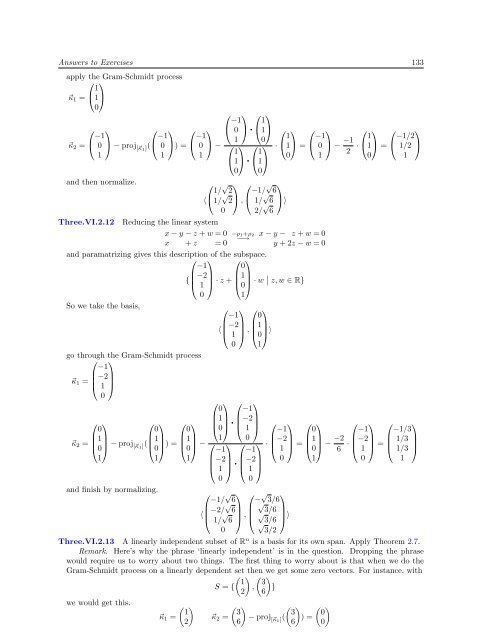
apply the Gram-Schmidt process
⎛ ⎞
⃗κ 1 = ⎝ 1 1⎠
0
⎛ ⎞ ⎛ ⎞
⎛
⃗κ 2 = ⎝ −1
⎞ ⎛
0 ⎠ − proj [⃗κ1]( ⎝ −1
⎞ ⎛
0 ⎠) = ⎝ −1
⎞ ⎝ −1
0 ⎠ ⎝ 1 1⎠
⎛
1 0
0 ⎠ − ⎛
1
1 1
⎝ 1 ⎞ ⎛
1⎠
⎝ 1 ⎞ · ⎝ 1 ⎞ ⎛
1⎠ = ⎝ −1
⎞ ⎛
0 ⎠ − −1
2 · ⎝ 1 ⎞ ⎛
1⎠ = ⎝ −1/2
⎞
1/2 ⎠
0 1
0 1
1⎠
0 0
and then normalize.
⎛
〈 ⎝ 1/√ ⎞ ⎛
2
1/ √ −1/ √ ⎞
6
2⎠ , ⎝ 1/ √ 6
0 2/ √ ⎠〉
6
Three.VI.2.12
Reducing the linear system
x − y − z + w = 0
x + z = 0
−ρ 1 +ρ 2
−→
x − y − z + w = 0
y + 2z − w = 0
and paramatrizing gives this description of the subspace.
⎛ ⎞ ⎛ ⎞
−1 0
{ ⎜−2
⎟
⎝ 1 ⎠ · z + ⎜1
⎟
⎝0⎠ · w ∣ z, w ∈ R}
0 1
So we take the basis,
⎛ ⎞ ⎛ ⎞
−1 0
〈 ⎜−2
⎟
⎝ 1 ⎠ , ⎜1
⎟
⎝0⎠ 〉
0 1
go through the Gram-Schmidt process
⎛ ⎞
−1
⃗κ 1 = ⎜−2
⎟
⎝ 1 ⎠
0
⎛ ⎞ ⎛ ⎞
0 −1
⎛ ⎞ ⎛ ⎞ ⎛ ⎞ ⎜1
⎟ ⎜−2
⎟
0
0 0 ⎝0⎠
⎝ 1 ⎠
⎛ ⎞ ⎛ ⎞ ⎛ ⎞ ⎛ ⎞
−1 0
−1 −1/3
⃗κ 2 = ⎜1
⎟
⎝0⎠ − proj [⃗κ 1 ]( ⎜1
⎟
⎝0⎠ ) = ⎜1
⎟
⎝0⎠ − 1 0
⎛ ⎞ ⎛ ⎞ · ⎜−2
⎟
−1 −1 ⎝ 1 ⎠ = ⎜1
⎟
⎝0⎠ − −2
6 ·
⎜−2
⎟
⎝ 1 ⎠ = ⎜ 1/3
⎟
⎝ 1/3 ⎠
1
1 1
⎜−2
⎟ ⎜−2
0 1
0 1
⎟
⎝ 1 ⎠ ⎝ 1 ⎠
0 0
and finish by normalizing.
⎛
−1/ √ ⎞ ⎛
6
〈 ⎜−2/ √ − √ ⎞
√
3/6
6
⎝ 1/ √ ⎟
6 ⎠ , ⎜ 3/6
√ ⎟
⎝
√ 3/6 ⎠ 〉
0 3/2
Three.VI.2.13 A linearly independent subset of R n is a basis for its own span. Apply Theorem 2.7.
Remark. Here’s why the phrase ‘linearly independent’ is in the question. Dropping the phrase
would require us to worry about two things. The first thing to worry about is that when we do the
Gram-Schmidt process on a linearly dependent set then we get some zero vectors. For instance, with
( ( 1 3
S = { , }
2)
6)
we would get this.
(
1
⃗κ 1 =
2)
⃗κ 2 =
( ( (
3 3 0
− proj
6)
[⃗κ1]( ) =
6)
0)