Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Linear Algebra Exercises-n-Answers.pdf
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<strong>Answers</strong> to <strong>Exercises</strong> 123<br />
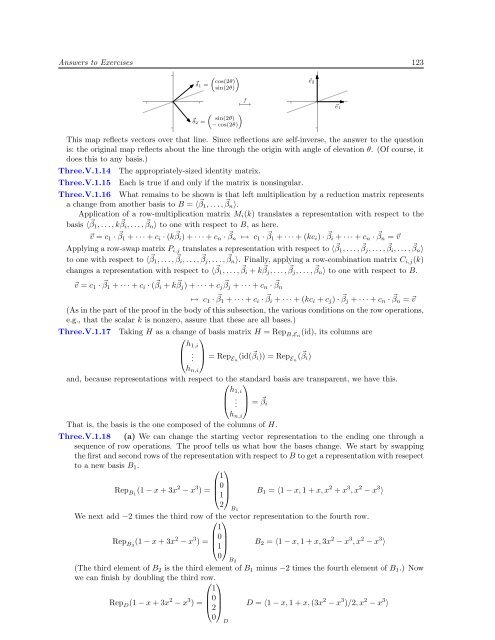
( )<br />
⃗ cos(2θ)<br />
δ1 =<br />
sin(2θ)<br />
⃗e 2<br />
↦−→<br />
( )<br />
⃗ sin(2θ)<br />
δ2 =<br />
− cos(2θ)<br />
f<br />
⃗e 1<br />
This map reflects vectors over that line. Since reflections are self-inverse, the answer to the question<br />
is: the original map reflects about the line through the origin with angle of elevation θ. (Of course, it<br />
does this to any basis.)<br />
Three.V.1.14<br />
Three.V.1.15<br />
The appropriately-sized identity matrix.<br />
Each is true if and only if the matrix is nonsingular.<br />
Three.V.1.16 What remains to be shown is that left multiplication by a reduction matrix represents<br />
a change from another basis to B = 〈 β ⃗ 1 , . . . , β ⃗ n 〉.<br />
Application of a row-multiplication matrix M i (k) translates a representation with respect to the<br />
basis 〈 β ⃗ 1 , . . . , kβ ⃗ i , . . . , β ⃗ n 〉 to one with respect to B, as here.<br />
⃗v = c 1 · ⃗β 1 + · · · + c i · (kβ ⃗ i ) + · · · + c n · ⃗β n ↦→ c 1 · ⃗β 1 + · · · + (kc i ) · ⃗β i + · · · + c n · ⃗β n = ⃗v<br />
Applying a row-swap matrix P i,j translates a representation with respect to 〈 β ⃗ 1 , . . . , β ⃗ j , . . . , β ⃗ i , . . . , β ⃗ n 〉<br />
to one with respect to 〈 β ⃗ 1 , . . . , β ⃗ i , . . . , β ⃗ j , . . . , β ⃗ n 〉. Finally, applying a row-combination matrix C i,j (k)<br />
changes a representation with respect to 〈 β ⃗ 1 , . . . , β ⃗ i + kβ ⃗ j , . . . , β ⃗ j , . . . , β ⃗ n 〉 to one with respect to B.<br />
⃗v = c 1 · ⃗β 1 + · · · + c i · ( ⃗ β i + k ⃗ β j ) + · · · + c j<br />
⃗ βj + · · · + c n · ⃗β n<br />
↦→ c 1 · ⃗β 1 + · · · + c i · ⃗β i + · · · + (kc i + c j ) · ⃗β j + · · · + c n · ⃗β n = ⃗v<br />
(As in the part of the proof in the body of this subsection, the various conditions on the row operations,<br />
e.g., that the scalar k is nonzero, assure that these are all bases.)<br />
Three.V.1.17 Taking H as a change of basis matrix H = Rep B,En (id), its columns are<br />
⎛ ⎞<br />
h 1,i<br />
⎜ . ⎟<br />
⎝ . ⎠ = Rep En<br />
(id( β ⃗ i )) = Rep En<br />
( β ⃗ i )<br />
h n,i<br />
and, because representations with respect to the standard basis are transparent, we have this.<br />
⎛ ⎞<br />
h 1,i<br />
⎜<br />
⎝<br />
⎟<br />
. ⎠ = β ⃗ i<br />
h n,i<br />
That is, the basis is the one composed of the columns of H.<br />
Three.V.1.18 (a) We can change the starting vector representation to the ending one through a<br />
sequence of row operations. The proof tells us what how the bases change. We start by swapping<br />
the first and second rows of the representation with respect to B to get a representation with resepect<br />
to a new basis B 1 .<br />
⎛ ⎞<br />
1<br />
Rep B1<br />
(1 − x + 3x 2 − x 3 ) = ⎜0<br />
⎟<br />
⎝ B<br />
1<br />
1 = 〈1 − x, 1 + x, x 2 + x 3 , x 2 − x 3 〉<br />
2<br />
⎠B 1<br />
We next add −2 times the third row of<br />
⎛<br />
the<br />
⎞<br />
vector representation to the fourth row.<br />
1<br />
Rep B3<br />
(1 − x + 3x 2 − x 3 ) = ⎜0<br />
⎟<br />
⎝ B<br />
1<br />
2 = 〈1 − x, 1 + x, 3x 2 − x 3 , x 2 − x 3 〉<br />
0<br />
⎠B 2<br />
(The third element of B 2 is the third element of B 1 minus −2 times the fourth element of B 1 .) Now<br />
we can finish by doubling the third row.<br />
⎛ ⎞<br />
1<br />
Rep D (1 − x + 3x 2 − x 3 ) = ⎜0<br />
⎟<br />
⎝2⎠<br />
D = 〈1 − x, 1 + x, (3x 2 − x 3 )/2, x 2 − x 3 〉<br />
0<br />
D