Linear Algebra Exercises-n-Answers.pdf

112 Linear Algebra, by Hefferon
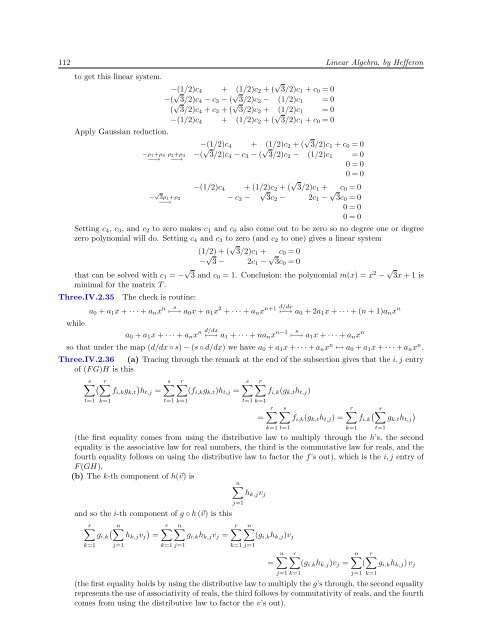
to get this linear system.
Apply Gaussian reduction.
−(1/2)c 4 + (1/2)c 2 + ( √ 3/2)c 1 + c 0 = 0
−( √ 3/2)c 4 − c 3 − ( √ 3/2)c 2 − (1/2)c 1 = 0
( √ 3/2)c 4 + c 3 + ( √ 3/2)c 2 + (1/2)c 1 = 0
−(1/2)c 4 + (1/2)c 2 + ( √ 3/2)c 1 + c 0 = 0
−ρ 1+ρ 4
−→
ρ 2+ρ 3
− √ 3ρ 1+ρ 2
−(1/2)c 4 + (1/2)c 2 + ( √ 3/2)c 1 + c 0 = 0
−( √ 3/2)c −→ 4 − c 3 − ( √ 3/2)c 2 − (1/2)c 1 = 0
0 = 0
0 = 0
−(1/2)c 4 + (1/2)c 2 + ( √ 3/2)c 1 + c 0 = 0
− c −→ 3 − √ 3c 2 − 2c 1 − √ 3c 0 = 0
0 = 0
0 = 0
Setting c 4 , c 3 , and c 2 to zero makes c 1 and c 0 also come out to be zero so no degree one or degree
zero polynomial will do. Setting c 4 and c 3 to zero (and c 2 to one) gives a linear system
(1/2) + ( √ 3/2)c 1 + c 0 = 0
− √ 3 − 2c 1 − √ 3c 0 = 0
that can be solved with c 1 = − √ 3 and c 0 = 1. Conclusion: the polynomial m(x) = x 2 − √ 3x + 1 is
minimal for the matrix T .
Three.IV.2.35
while
The check is routine:
a 0 + a 1 x + · · · + a n x n
s
↦−→ a 0 x + a 1 x 2 n+1 d/dx
+ · · · + a n x ↦−→ a 0 + 2a 1 x + · · · + (n + 1)a n x n
a 0 + a 1 x + · · · + a n x n d/dx
↦−→ a 1 + · · · + na n x n−1 s
↦−→ a 1 x + · · · + a n x n
so that under the map (d/dx ◦ s) − (s ◦ d/dx) we have a 0 + a 1 x + · · · + a n x n ↦→ a 0 + a 1 x + · · · + a n x n .
Three.IV.2.36 (a) Tracing through the remark at the end of the subsection gives that the i, j entry
of (F G)H is this
s∑ ( ∑
r )
s∑ r∑
s∑ r∑
f i,k g k,t ht,j = (f i,k g k,t )h t,j = f i,k (g k,t h t,j )
t=1
k=1
t=1 k=1
t=1 k=1
=
r∑
k=1 t=1
s∑
f i,k (g k,t h t,j ) =
r∑ ( ∑
s )
f i,k g k,t h t,j
(the first equality comes from using the distributive law to multiply through the h’s, the second
equality is the associative law for real numbers, the third is the commutative law for reals, and the
fourth equality follows on using the distributive law to factor the f’s out), which is the i, j entry of
F (GH).
(b) The k-th component of h(⃗v) is
n∑
h k,j v j
and so the i-th component of g ◦ h (⃗v) is this
r∑ ( ∑
n )
r∑ n∑
r∑ n∑
g i,k h k,j v j = g i,k h k,j v j = (g i,k h k,j )v j
k=1
j=1
k=1 j=1
j=1
k=1 j=1
=
n∑
j=1 k=1
r∑
(g i,k h k,j )v j =
k=1
j=1 k=1
t=1
n∑ r∑
( g i,k h k,j ) v j
(the first equality holds by using the distributive law to multiply the g’s through, the second equality
represents the use of associativity of reals, the third follows by commutativity of reals, and the fourth
comes from using the distributive law to factor the v’s out).