Linear Algebra Exercises-n-Answers.pdf

Answers to Exercises 105
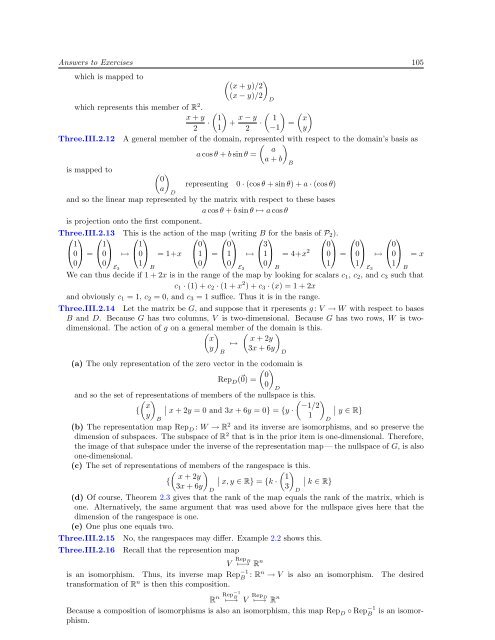
which is mapped to
which represents this member of R 2 .
x + y
2
Three.III.2.12
is mapped to ( 0
a)
( ) (x + y)/2
(x − y)/2
D
(
1
· +
1)
x − y
2
( (
1 x
· =
−1)
y)
A general member of the domain, represented
( )
with respect to the domain’s basis as
a
a cos θ + b sin θ =
a + b
D
representing 0 · (cos θ + sin θ) + a · (cos θ)
and so the linear map represented by the matrix with respect to these bases
a cos θ + b sin θ ↦→ a cos θ
is projection onto the first component.
Three.III.2.13 This is the action of the map (writing B for the basis of P 2 ).
⎛
⎝ 1 ⎞ ⎛
0⎠ = ⎝ 1 ⎞ ⎛
0 ↦→ ⎝ 1 ⎞
⎛
0⎠
= 1+x ⎝ 0 ⎞ ⎛
1⎠ = ⎝ 0 ⎞ ⎛
1 ↦→ ⎝ 3 ⎞
⎛
1⎠
= 4+x
0 0 1
0 0 0
⎠E 3
⎠E 2 ⎝ 0 ⎞ ⎛
0⎠ = ⎝ 0 ⎞
0
1 1
3
B
B
B
⎠E 3
↦→
⎛
⎞
⎝ 0 0⎠
1
We can thus decide if 1 + 2x is in the range of the map by looking for scalars c 1 , c 2 , and c 3 such that
c 1 · (1) + c 2 · (1 + x 2 ) + c 3 · (x) = 1 + 2x
and obviously c 1 = 1, c 2 = 0, and c 3 = 1 suffice. Thus it is in the range.
Three.III.2.14 Let the matrix be G, and suppose that it rperesents g : V → W with respect to bases
B and D. Because G has two columns, V is two-dimensional. Because G has two rows, W is twodimensional.
The action of g on a general
(
member
(
of the domain
)
is this.
x x + 2y
↦→
y)
3x + 6y
(a) The only representation of the zero vector in the codomain is
( 0
Rep D (⃗0) =
0)
and so the set of representations
(
of members of the nullspace is
(
this.
)
x { ∣ −1/2
x + 2y = 0 and 3x + 6y = 0} = {y ·
y)
1
B
B
D
D
D
∣ y ∈ R}
(b) The representation map Rep D : W → R 2 and its inverse are isomorphisms, and so preserve the
dimension of subspaces. The subspace of R 2 that is in the prior item is one-dimensional. Therefore,
the image of that subspace under the inverse of the representation map — the nullspace of G, is also
one-dimensional.
(c) The set of representations of members of the rangespace is this.
( )
x + 2y
{
3x + 6y
D
∣ x, y ∈ R} = {k ·
(
1
3)
D
∣ k ∈ R}
(d) Of course, Theorem 2.3 gives that the rank of the map equals the rank of the matrix, which is
one. Alternatively, the same argument that was used above for the nullspace gives here that the
dimension of the rangespace is one.
(e) One plus one equals two.
Three.III.2.15
Three.III.2.16
No, the rangespaces may differ. Example 2.2 shows this.
Recall that the represention map
V Rep B
↦−→ R n
is an isomorphism. Thus, its inverse map Rep −1
B : Rn → V is also an isomorphism. The desired
transformation of R n is then this composition.
is an isomor-
↦−→ V Rep D
↦−→ R n
Because a composition of isomorphisms is also an isomorphism, this map Rep D ◦ Rep −1
B
phism.
R n Rep−1 B
B
= x