Chapter 3: THE FRIEDMANN MODELS
Chapter 3: THE FRIEDMANN MODELS
Chapter 3: THE FRIEDMANN MODELS
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
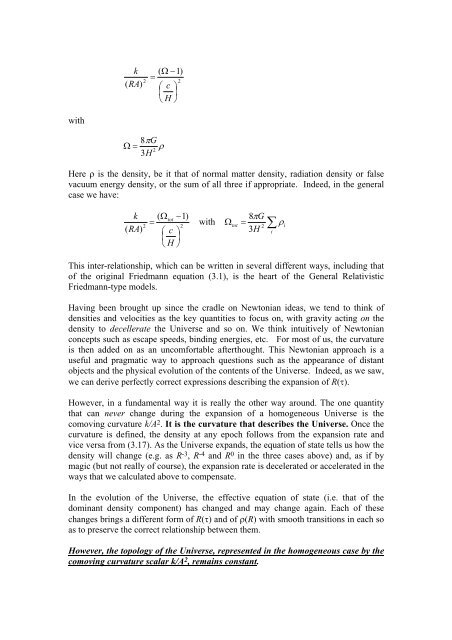
k<br />
( RA)<br />
2<br />
( Ω −1)<br />
=<br />
2<br />
⎛ c ⎞<br />
⎜ ⎟<br />
⎝ H ⎠<br />
with<br />
Ω= 8 πG<br />
ρ<br />
3H<br />
2<br />
Here ρ is the density, be it that of normal matter density, radiation density or false<br />
vacuum energy density, or the sum of all three if appropriate. Indeed, in the general<br />
case we have:<br />
k<br />
( RA)<br />
2<br />
( Ωtot<br />
=<br />
⎛ c<br />
⎜<br />
⎝ H<br />
−1)<br />
2<br />
⎞<br />
⎟<br />
⎠<br />
with<br />
Ω<br />
tot<br />
8πG<br />
=<br />
2<br />
3H<br />
∑<br />
i<br />
ρ<br />
i<br />
This inter-relationship, which can be written in several different ways, including that<br />
of the original Friedmann equation (3.1), is the heart of the General Relativistic<br />
Friedmann-type models.<br />
Having been brought up since the cradle on Newtonian ideas, we tend to think of<br />
densities and velocities as the key quantities to focus on, with gravity acting on the<br />
density to decellerate the Universe and so on. We think intuitively of Newtonian<br />
concepts such as escape speeds, binding energies, etc. For most of us, the curvature<br />
is then added on as an uncomfortable afterthought. This Newtonian approach is a<br />
useful and pragmatic way to approach questions such as the appearance of distant<br />
objects and the physical evolution of the contents of the Universe. Indeed, as we saw,<br />
we can derive perfectly correct expressions describing the expansion of R(τ).<br />
However, in a fundamental way it is really the other way around. The one quantity<br />
that can never change during the expansion of a homogeneous Universe is the<br />
comoving curvature k/A 2 . It is the curvature that describes the Universe. Once the<br />
curvature is defined, the density at any epoch follows from the expansion rate and<br />
vice versa from (3.17). As the Universe expands, the equation of state tells us how the<br />
density will change (e.g. as R -3 , R -4 and R 0 in the three cases above) and, as if by<br />
magic (but not really of course), the expansion rate is decelerated or accelerated in the<br />
ways that we calculated above to compensate.<br />
In the evolution of the Universe, the effective equation of state (i.e. that of the<br />
dominant density component) has changed and may change again. Each of these<br />
changes brings a different form of R(τ) and of ρ(R) with smooth transitions in each so<br />
as to preserve the correct relationship between them.<br />
However, the topology of the Universe, represented in the homogeneous case by the<br />
comoving curvature scalar k/A 2 , remains constant.