Lecture 18 Subgradients

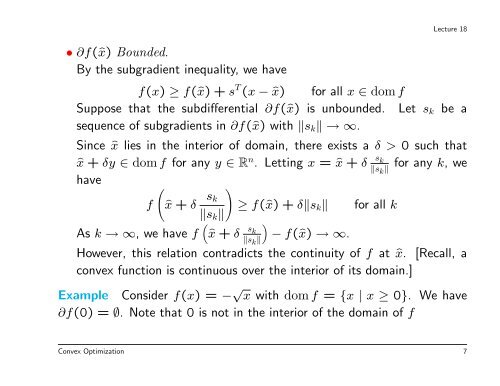
• ∂f(ˆx) Bounded.
By the subgradient inequality, we have
Lecture 18
f(x) ≥ f(ˆx) + s T (x − ˆx) for all x ∈ dom f
Suppose that the subdifferential ∂f(ˆx) is unbounded. Let s k be a
sequence of subgradients in ∂f(ˆx) with ‖s k ‖ → ∞.
Since ˆx lies in the interior of domain, there exists a δ > 0 such that
ˆx + δy ∈ dom f for any y ∈ R n . Letting x = ˆx + δ s k
for any k, we
‖s k ‖
have
(
f ˆx + δ
s )
k
≥ f(ˆx) + δ‖s k ‖ for all k
‖s k ‖
As k → ∞, we have f (ˆx + δ s )
k
‖s k ‖ − f(ˆx) → ∞.
However, this relation contradicts the continuity of f at ˆx. [Recall, a
convex function is continuous over the interior of its domain.]
Example Consider f(x) = − √ x with dom f = {x | x ≥ 0}. We have
∂f(0) = ∅. Note that 0 is not in the interior of the domain of f
Convex Optimization 7