Equivariant Embeddings of Algebraic Groups

Theorem 6 ([26], Weyl’s Character Formula). For any character λ ∈ X ∗ (T ),
χ(λ) = J(λ + ρ)/J(ρ),
where J(µ) = ∑ w∈W (−1)l(w) e w·µ and ρ = 1 2
∑
α∈Φ + (T,G) α.
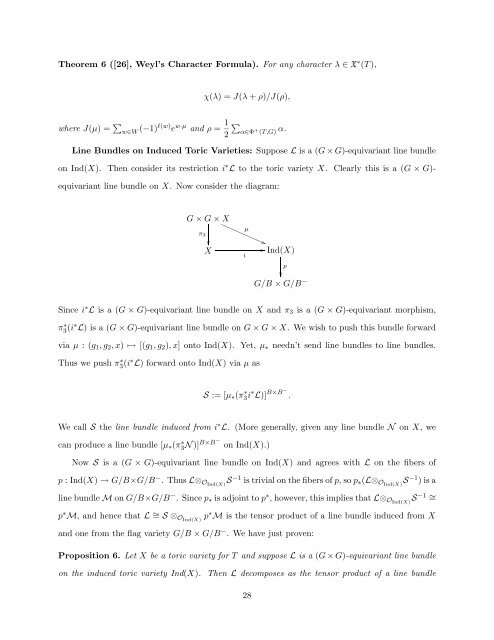
Line Bundles on Induced Toric Varieties: Suppose L is a (G × G)-equivariant line bundle
on Ind(X). Then consider its restriction i ∗ L to the toric variety X. Clearly this is a (G × G)-
equivariant line bundle on X. Now consider the diagram:
G × G × X
µ
π 3
X
i
Ind(X)
p
G/B × G/B −
Since i ∗ L is a (G × G)-equivariant line bundle on X and π 3 is a (G × G)-equivariant morphism,
π3 ∗(i∗ L) is a (G × G)-equivariant line bundle on G × G × X. We wish to push this bundle forward
via µ : (g 1 , g 2 , x) ↦→ [(g 1 , g 2 ), x] onto Ind(X). Yet, µ ∗ needn’t send line bundles to line bundles.
Thus we push π3 ∗(i∗ L) forward onto Ind(X) via µ as
S := [µ ∗ (π ∗ 3i ∗ L)] B×B− .
We call S the line bundle induced from i ∗ L. (More generally, given any line bundle N on X, we
can produce a line bundle [µ ∗ (π ∗ 3 N )]B×B−
on Ind(X).)
Now S is a (G × G)-equivariant line bundle on Ind(X) and agrees with L on the fibers of
p : Ind(X) → G/B×G/B − . Thus L⊗ OInd(X) S −1 is trivial on the fibers of p, so p ∗ (L⊗ OInd(X) S −1 ) is a
line bundle M on G/B×G/B − . Since p ∗ is adjoint to p ∗ , however, this implies that L⊗ OInd(X) S −1 ∼ =
p ∗ M, and hence that L ∼ = S ⊗ OInd(X) p ∗ M is the tensor product of a line bundle induced from X
and one from the flag variety G/B × G/B − . We have just proven:
Proposition 6. Let X be a toric variety for T and suppose L is a (G × G)-equivariant line bundle
on the induced toric variety Ind(X). Then L decomposes as the tensor product of a line bundle
28