Plane Waves

Chapter 3
Plane Waves
This chapter describes the behavior of plane waves at dielectric interfaces, as well as the reflection of plane
waves at multiple layers.
3.1 Plane waves at dielectric interfaces
An optical fiber is shown in fig. 3.1. It consists of a fiber core with the refraction index n 1 and a core
cladding with the refraction index n 2 < n 1 . In such an optical fiber the wave is guided by the constant total
reflection. For typical dimensions of the optical fiber ( e.g. fiber diameter 125 µm) there is a reflection e.g.
every millimeter as shown in fig. 3.1. Hence, there are about 10 6 reflexions per kilometer. With assumed
additional losses of less than 0.1 dB/km , the reflectivity per reflection has to be greater than 99, 999998% ,
so that ’total’ reflection is really required. What should the waveguide properties be in order to ensure this
amount of total internal reflection?
6 J = H A B A N E
E ? D J I J H = D
) K I > H A E J K C I H E ? D J K C
Figure 3.1: Principle of a waveguide with n 2 < n 1
To investigate the total reflection, the reflection of a plane wave at a dielectric boundary, in accordance with
fig. 3.2, should be considered in detail. The vectors ⃗ k 1 , ⃗ k 1 ′ and ⃗ k 2 , shown in fig. 3.2, describe the wave
vector of the incident, reflected and transmitted wave. In this example, ⃗ E is assumed perpendicular to the
1

0
-
0
-
0
O
G
-
N
Introduction to fiber optic communications ONT/ 2
plane of incidence. Therefore the wave vectors are equal to:
( ) 2 ( ) 2
⃗k1 = ⃗k1
′ = k
2
0 n 2 1 (3.1)
(
⃗k2
) 2
= k
2
0 n 2 2 (3.2)
For example: The vector ⃗ k 1 has an x- and a z-component. Thus:
⎛ ⎞
k x1
⃗ ⎜ ⎟
k1 = ⎝ 0 ⎠ (3.3)
k z with ∣ ⃗ ∣ ∣∣
2
k 1 = k
2
x1 + kz 2 = k0 2n2 1 . Furthermore it is:
cos(θ 1 ) = k z
∣ ⃗ ∣ ∣∣
=
k 1
k z
k 0 n 1
(3.4)
G
The components of the incident wave yield
Figure 3.2: A plane wave incident on a boundary surface
E y = E y1 exp (−j k x1 x − j k z z) (3.5)
H z = E y
sin (θ 1 )
Z F 1
(3.6)
H x = −E y
cos(θ 1 )
Z F 1
(3.7)

Introduction to fiber optic communications ONT/ 3
The characteristic wave impedances on both sides of the interface are
Z F 1 = 1 n 1
√
µ0
ε 0
, Z F 2 = 1 n 2
√
µ0
ε 0
(3.8)
At the interface ( x = 0 ) the tangential field components must be continuous. ⇒ The z-dependent components
of the incident, reflected and transmitted waves have to be equal.
⇒ Hence, the z-components of ⃗ k 1 , ⃗ k 1 ′ and ⃗ k 2 are equal (k z ).
k
For the transmitted wave there is z
| ⃗ = cos(θ k 2|
2) = k z
k 0 n 2
and therefore yields
This is Snell’s law:
k z = k 0 n 1 cos(θ 1 ) = k 0 n 2 cos(θ 2 ) (3.9)
n 1
= cos(θ 2)
n 2 cos(θ 1 ) = sin(φ 2)
sin(φ 1 )
(3.10)
If cos(θ 1 ) > n 2
n 1
or sin(φ 1 ) > n 2
n 1
, there won’t be a real solution in eq. (3.9) for a real angle θ 2 . The wave no
longer penetrates into medium 2. Total reflection occurs. The critical angle for total reflection is:
As long as it is θ 1 < θ 1g and hence φ 1 > φ 1g , total reflection takes place.
sin(φ 1g ) = cos(θ 1g ) = n 2
n 1
(3.11)
3.1.1 Determination of the reflection coefficient for plane waves at dielectric interfaces
The reflection coefficient r E is defined as r E = E y1 ′
E
. The index E of the reflection coefficient r
y1
E
specifies in this case that the E-field is polarized perpendicular to the plane of incidence. The demand for
the continuity of the tangential field components at the interface yields the following boundary conditions
E y1 + E y1 ′ = E y2 (3.12)
H z1 + H z1 ′ = H z2 (3.13)
The indexing 1, 1 ’and 2 refer to the incident, the reflected and the transmitted waves. In both media, wave
impedances are now introduced:
Z 1E = E y1
H z1
= − E y1 ′
H z1 ′
Z 2E = E y2
H z2
=
1
n 2 sin(θ 2 )
1
=
n 1 sin(θ 1 )
√
µ0
ε 0
= k 0
k x1
√
µ0
ε 0
(3.14)
√
µ0
ε 0
= k 0
k x2
√
µ0
ε 0
(3.15)
Now the components of the H-field in eq. (3.13) can be expressed by the components of the E-field. For
that eq. (3.14) and eq. (3.15) have to be inserted into eq. (3.13):
Z 2E
Z 2E
E y1 − E
Z y1 ′ = E
1E Z y2 (3.16)
1E
Inserting this expression into eq. (3.12) and transforming it, the reflection factor r E results in
r E = Z 2E − Z 1E
= n 1 sin(θ 1 ) − n 2 sin(θ 2 )
Z 2E + Z 1E n 1 sin(θ 1 ) + n 2 sin(θ 2 ) = n 1 cos(φ 1 ) − n 2 cos(φ 2 )
n 1 cos(φ 1 ) + n 2 cos(φ 2 )
(3.17)

Introduction to fiber optic communications ONT/ 4
Making use of Snell’s Law (eq. (3.10)) with n 1 = n 2 sin(φ 2 )/ sin(φ 1 ) , eq. (3.17) can be transformed to:
r E = sin(φ 2) cos(φ 1 ) − cos(φ 2 ) sin(φ 1 )
sin(φ 2 ) cos(φ 1 ) + cos(φ 2 ) sin(φ 1 ) = sin(φ 2 − φ 1 )
sin(φ 2 + φ 1 )
(3.18)
In Analogy for a wave with the H-field perpendicular to the incident plane the reflection factor r H can be
determined. Firstly, this result leads to the following field components of the incident wave:
with the characteristic wave impedances
H y = H y1 exp (−j k x1 x − j k z z) (3.19)
E z = −H y · sin (θ 1 ) · Z F 1 (3.20)
E x = H y · cos(θ 1 ) · Z F 1 (3.21)
Z 1H = − E z1
H y1
= E z1 ′
H y1 ′
= sin(θ 1)
n 1
√
µ0
ε 0
=
Z 2H = − E z2
H y2
= sin(θ 2)
n 2
√
µ0
ε 0
=
k x2
n 2 2 · k 0
k x1
√
µ0
ε 0
(3.22)
n 2 1 · k 0
√
µ0
(3.23)
ε 0
With r H = E z1 ′
E z1
= − H y1 ′
H y1
it results:
r H = Z 2H − Z 1H
= n 1 sin(θ 2 ) − n 2 sin(θ 1 )
Z 2H + Z 1H n 1 sin(θ 2 ) + n 2 sin(θ 1 ) = n 1 cos(φ 2 ) − n 2 cos(φ 1 )
n 1 cos(φ 2 ) + n 2 cos(φ 1 )
(3.24)
With Snell’s Law (3.10) eq. (3.24) is redefined to:
r H = cos(φ 2 + φ 1 ) · sin(φ 2 − φ 1 )
cos(φ 2 − φ 1 ) · sin(φ 2 + φ 1 )
(3.25)
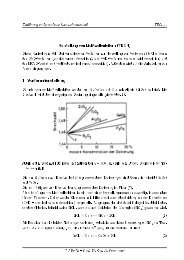
For the given refraction numbers n 1 and n 2 the reflection factors can now be determined as a function of the
incident angle. Fig. 3.3 shows two examples.
In fig. 3.3 the so called Brewster-angle φ 1B is represented, wherein r H = 0 results for the reflexion factor
φ 1 = φ 1B . Corresponding to eq. (3.25), φ 1B results when φ 1 + φ 2 = π/2 . Hence, in this case, the
reflected and transmitted beams are perpendicular to each other. Thus:
The Brewster angle is utilized e.g. for anechoic laser resonators (Fig. 3.4).
For n 1 = 1 (air) it is simply tg(φ 1B ) = n 2 .
tg(φ 1B ) = n 2
n 1
(3.26)
Example: For n 2 = 1.5 the Brewster angle results in φ 1B = 56.3 ◦ . Since only the polarization with ⃗ H
perpendicular to the incident plane penetrates into the gas discharge chamber losslessly, the emitted laser
beam is linearly polarized.
For φ 1 > φ 1g the wave number k x2 = k 0 ·
√
n 2 2 − n2 1 sin2 (φ 1 ) , as well as Z 2H becomes imaginary.
⇒ |r E | = |r H | = 1 (3.27)

Introduction to fiber optic communications ONT/ 5
Figure 3.3: Magnitude of the amplitude reflection coefficients at an interface of glass/air, shown as a function
of the incident angle φ. (a) the incident wave proceeds in air, (b) in glass.
/ = I A J = @ K C I H = K
5 F E A C A
*
Figure 3.4: Schematic of the laser resonator

N
Introduction to fiber optic communications ONT/ 6
Assuming a total reflection, the field in region 2 (refraction index n 2 ) decreases exponentially with the
penetration depth
d x = 1
j · k x2
=
λ
√
2π n 2 1 sin2 (φ 1 ) − n 2 2
For √ n 2 1 − n2 2 = 0.2 and φ 1 = 90 ◦ , the result is for example d x = 0.8 · λ .
(3.28)
3.2 Reflection on multi layers
The reflection of a plane wave at multiple layers in the x-direction is examined, where each layer has a
different refractive index. m layers each with a refraction number n i ( i = 1...m ) are assumed (fig. 3.5).
!
E
@
E
Figure 3.5: m-Layers with the refraction numbers n i and the thicknesses d i
If the incident angle φ 1 is given, the x-component of the wave number in the i-th layer k xi , based on the
constant wave component in z-direction k z = k 0 n 1 sin(φ 1 ) , will be determined as:
k xi = k 0
√n 2 i − n2 1 sin2 (φ 1 ) (3.29)
and the characteristic wave impedances Z iE and Z iH , as shown in eq. (3.14), eq. (3.15), eq. (3.22) and eq.
(3.23), are
Z iE = k 0
k xi
√
µ0
Z iH = k xi
n 2 i k 0
(3.30)
ε 0
√
µ0
. (3.31)
ε 0
Since the wave resistance of the layers can be calculated directly, it is possible to develop an equivalent
electronic circuit (fig. 3.6).
In order to calculate the reflection coefficient at the first layer, the terminating resistor has to be transformed
to the input (e.g. with the Smith chart, see lecture Hochfrequenztechnik I for further details). Hence the
problem is solved in general. A particularly simple and special case is λ 4 -layers.
Accordingly it applies for λ 4 -layers: k xi · d i = π 2
. In this case it is possible to simplify to following
expression:
Z ai =
Z2 iE
Z a(i+1)
(3.32)

Introduction to fiber optic communications ONT/ 7
-
-
N
E -
N E
-
N
-
=
@
E
* A H A ? D K C @ A I 4 A B A N E I B = J H I
E @ E A I A H - > A A
Figure 3.6: equivalent electronic circuit for ⃗ E perpendicular to the incident plane
@
E
F
N E
= E
Figure 3.7: equivalent resistance for λ/4-layers
to transform step-by-step the resistance Z a(i+1) a layer forward to Z ai (fig. 3.7). With multiple λ/4 -layers
this transformation can be applied recursively, leading to Z a . Then the reflection factor can be calculated by
r E = Z a − Z 1E
Z a + Z 1E
. (3.33)
As an example, a dielectric layer can be determined, so that the transition from medium 1 to medium 3 is
completely non-reflective, i.e. the wave couples without reflection into the dielectric with n 3 (fig. 3.8). For
simplicity, a perpendicular wave incidence (φ 1 = 0) is assumed. In this case, the wave impedances are
given as:
Z iE = Z iH = Z F i = 1 n i
√
µ0
ε 0
(3.34)
Z F 2 and Z F 3 are merged to the equivalent impedance Z a .
Z a = Z2 F 2
= n √
3 µ0
Z F 3 n 2 (3.35)
2
ε 0
Hence it results Z a = Z F 1 for r E = r H = 0. And furthermore
Z F 2 = √ Z F 1 · Z F 3 ⇒ n 2 = √ n 1 · n 3 (3.36)
Also the thickness of the layer can be determined, since following relation applies:
d 2 · k x2 = d 2 · k 0 · n 2 = d 2 · n 2
2π
λ
!
= π 2
(3.37)

Introduction to fiber optic communications ONT/ 8
I A H A ? D J A E B = A @ A
A > A A 9 A A
!
@ "
.
.
. !
=
.
. !
* A H A ? D K C @ A I 4 A B A N E I B = J H I
E @ E A I A H - > A A
Figure 3.8: e.g.: λ/4-layer for anti-reflection
Hence the thickness of the layer can be evaluated as
d 2 =
λ
4 · n 2
(3.38)