Etudes et évaluation de processus océaniques par des hiérarchies ...

138 CHAPITRE 4. ETUDES DE PROCESSUS OCÉANOGRAPHIQUES
558 Ocean Dynamics (2009) 59:551–563
tel-00545911, version 1 - 13 Dec 2010
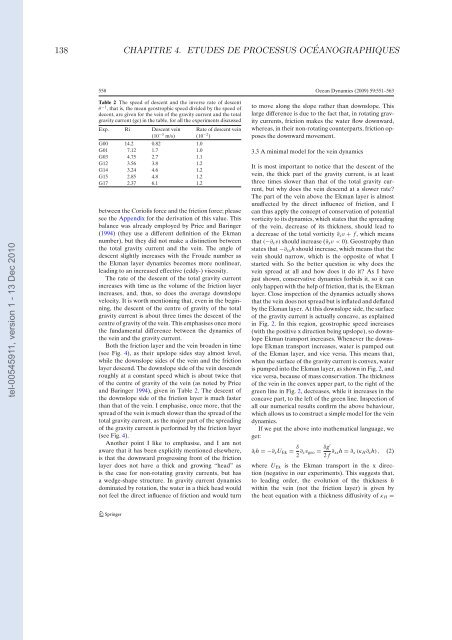
Table 2 The speed of descent and the inverse rate of descent
θ −1 , that is, the mean geostrophic speed divided by the speed of
decent, are given for the vein of the gravity current and the total
gravity current (gc) in the table, for all the experiments discussed
Exp. Ri Descent vein Rate of descent vein
(10 −3 m/s) (10 −2 )
G00 14.2 0.82 1.0
G01 7.12 1.7 1.0
G03 4.75 2.7 1.1
G12 3.56 3.8 1.2
G14 3.24 4.6 1.2
G15 2.85 4.8 1.2
G17 2.37 6.1 1.2
between the Coriolis force and the friction force; please
see the Appendix for the derivation of this value. This
balance was already employed by Price and Baringer
(1994) (they use a different definition of the Ekman
number), but they did not make a distinction between
the total gravity current and the vein. The angle of
descent slightly increases with the Froude number as
the Ekman layer dynamics becomes more nonlinear,
leading to an increased effective (eddy-) viscosity.
The rate of the descent of the total gravity current
increases with time as the volume of the friction layer
increases, and, thus, so does the average downslope
velocity. It is worth mentioning that, even in the beginning,
the descent of the centre of gravity of the total
gravity current is about three times the descent of the
centre of gravity of the vein. This emphasises once more
the fundamental difference between the dynamics of
the vein and the gravity current.
Both the friction layer and the vein broaden in time
(see Fig. 4), as their upslope sides stay almost level,
while the downslope sides of the vein and the friction
layer descend. The downslope side of the vein descends
roughly at a constant speed which is about twice that
of the centre of gravity of the vein (as noted by Price
and Baringer 1994), given in Table 2. The descent of
the downslope side of the friction layer is much faster
than that of the vein. I emphasise, once more, that the
spread of the vein is much slower than the spread of the
total gravity current, as the major part of the spreading
of the gravity current is performed by the friction layer
(see Fig. 4).
Another point I like to emphasise, and I am not
aware that it has been explicitly mentioned elsewhere,
is that the downward progressing front of the friction
layer does not have a thick and growing “head” as
is the case for non-rotating gravity currents, but has
a wedge-shape structure. In gravity current dynamics
dominated by rotation, the water in a thick head would
not feel the direct influence of friction and would turn
to move along the slope rather than downslope. This
large difference is due to the fact that, in rotating gravity
currents, friction makes the water flow downward,
whereas, in their non-rotating counterparts, friction opposes
the downward movement.
3.3 A minimal model for the vein dynamics
It is most important to notice that the descent of the
vein, the thick part of the gravity current, is at least
three times slower than that of the total gravity current,
but why does the vein descend at a slower rate?
The part of the vein above the Ekman layer is almost
unaffected by the direct influence of friction, and I
can thus apply the concept of conservation of potential
vorticity to its dynamics, which states that the spreading
of the vein, decrease of its thickness, should lead to
a decrease of the total vorticity ∂ x v + f , which means
that (−∂ x v) should increase (∂ x v < 0). Geostrophy than
states that −∂ xx h should increase, which means that the
vein should narrow, which is the opposite of what I
started with. So the better question is: why does the
vein spread at all and how does it do it? As I have
just shown, conservative dynamics forbids it, so it can
only happen with the help of friction, that is, the Ekman
layer. Close inspection of the dynamics actually shows
that the vein does not spread but is inflated and deflated
by the Ekman layer. At this downslope side, the surface
of the gravity current is actually concave, as explained
in Fig. 2. In this region, geostrophic speed increases
(with the positive x direction being upslope), so downslope
Ekman transport increases. Whenever the downslope
Ekman transport increases, water is pumped out
of the Ekman layer, and vice versa. This means that,
when the surface of the gravity current is convex, water
is pumped into the Ekman layer, as shown in Fig. 2, and
vice versa, because of mass conservation. The thickness
of the vein in the convex upper part, to the right of the
green line in Fig. 2, decreases, while it increases in the
concave part, to the left of the green line. Inspection of
all our numerical results confirm the above behaviour,
which allows us to construct a simple model for the vein
dynamics.
If we put the above into mathematical language, we
get:
∂ t h = −∂ x U Ek = δ 2 ∂ xv geo = δg′
2 f ∂ xxh = ∂ x (κ H ∂ x h), (2)
where U Ek is the Ekman transport in the x direction
(negative in our experiments). This suggests that,
to leading order, the evolution of the thickness h
within the vein (not the friction layer) is given by
the heat equation with a thickness diffusivity of κ H =