A Brief Review of Elasticity and Viscoelasticity for Solids 1 Introduction

More documents

Recommendations

Info

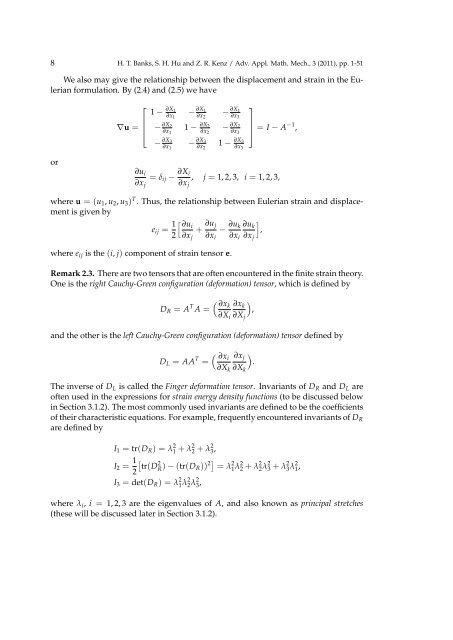
8 H. T. Banks, S. H. Hu and Z. R. Kenz / Adv. Appl. Math. Mech., 3 (2011), pp. 1-51 We also may give the relationship between the displacement and strain in the Eulerian formulation. By (2.4) and (2.5) we have ∇u = ⎡ ⎢ ⎣ 1 − ∂X 1 ∂x 1 − ∂X 1 ∂x 2 − ∂X 1 ∂x 3 − ∂X 2 ∂x 1 1 − ∂X 2 ∂x 2 − ∂X 2 ∂x 3 − ∂X 3 ∂x 1 − ∂X 3 ∂x 2 1 − ∂X 3 ∂x 3 ⎤ ⎥ ⎦ = I − A−1 , or ∂u i ∂x j = δ ij − ∂X i ∂x j , j = 1, 2, 3, i = 1, 2, 3, where u = (u 1 , u 2 , u 3 ) T . Thus, the relationship between Eulerian strain and displacement is given by e ij = 1 [ ∂ui + ∂u j − ∂u k ∂u ] k , 2 ∂x j ∂x i ∂x i ∂x j where e ij is the (i, j) component of strain tensor e. Remark 2.3. There are two tensors that are often encountered in the finite strain theory. One is the right Cauchy-Green configuration (deformation) tensor, which is defined by D R = A T A = ( ∂xk ∂x ) k , ∂X i ∂X j and the other is the left Cauchy-Green configuration (deformation) tensor defined by D L = AA T = ( ∂xi ∂X k ∂x j ∂X k ) . The inverse of D L is called the Finger deformation tensor. Invariants of D R and D L are often used in the expressions for strain energy density functions (to be discussed below in Section 3.1.2). The most commonly used invariants are defined to be the coefficients of their characteristic equations. For example, frequently encountered invariants of D R are defined by I 1 = tr(D R ) = λ 2 1 + λ2 2 + λ 2 3 , I 2 = 1 2[ tr(D 2 R ) − (tr(D R )) 2] = λ 2 1 λ2 2 + λ 2 2 λ2 3 + λ 2 3 λ2 1 , I 3 = det(D R ) = λ 2 1 λ2 2 λ2 3 , where λ i , i = 1, 2, 3 are the eigenvalues of A, and also known as principal stretches (these will be discussed later in Section 3.1.2).
H. T. Banks, S. H. Hu and Z. R. Kenz / Adv. Appl. Math. Mech., 3 (2011), pp. 1-51 9 Infinitesimal strain theory Infinitesimal strain theory is also called small deformation theory, small displacement theory or small displacement-gradient theory. In infinitesimal strain theory, it is assumed that the components of displacement u i are such that their first derivatives are so small that higher order terms such as the squares and the products of the partial derivatives of u i are negligible compared with the first-order terms. In this case e ij reduces to Cauchy’s infinitesimal strain tensor ε ij = 1 [ ∂ui + ∂u ] j , (2.8) 2 ∂x j ∂x i (hence the infinitesimal strain tensor is also symmetric). Thus, ε ij = ε ji . We note that in this case the distinction between the Lagrangian and Eulerian strain tensors disappears (i.e., E ij ≈ e ij ≈ ε ij ), since it is immaterial whether the derivatives of the displacement are calculated at the position of a point before or after deformation. Hence, the necessity of specifying whether the strains are measured with respect to the initial configuration (Lagrangian description) or with respect to the deformed configuration (Eulerian description) is characteristic of a finite strain analysis and the two different formulations are typically not encountered in the infinitesimal theory. 2.1.3 Stress Stress is a measure of the average amount of force exerted per unit area (in units N/m 2 or Pa), and it is a reaction to external forces on a surface of a body. Stress was introduced into the theory of elasticity by Cauchy almost two hundred years ago. Definition 2.4. The stress vector (traction) is defined by T (n) = dF dΓ = lim ∆F ∆Γ→0 ∆Γ , where the superscript (n) is introduced to denote the direction of the normal vector n of the surface Γ and F is the force on the surface. To further elaborate on this concept, consider a small cube in the body as depicted in Fig. 2 (left). Let the surface of the cube normal (perpendicular) to the axis z be donated by ∇Γ z . Let the stress vector that acts on the surface ∇Γ z be T (e 3) , where e 3 = (0, 0, 1) T . Resolve T (e 3) into three components in the direction of the coordinate axes and denote them by σ zx , σ zy and σ zz . Similarly we may consider surface ∇Γ x and ∇Γ y perpendicular to x and y, the stress vectors acting on them, and their components in the x, y and z directions. The components σ xx , σ yy and σ zz are called normal stresses, and σ xy , σ xz , σ yx , σ yz , σ zx and σ zy are called shear stresses. A stress component is positive if it acts in the positive direction of the coordinate axes. We remark that the notation σ face, direction is consistently used in elasticity theory.
Page 1 and 2: Advances in Applied Mathematics and
Page 3 and 4: H. T. Banks, S. H. Hu and Z. R. Ken
Page 7: H. T. Banks, S. H. Hu and Z. R. Ken
Page 51: H. T. Banks, S. H. Hu and Z. R. Ken

A Brief Review of Elasticity and Viscoelasticity for Solids 1 Introduction

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?