The GNSS integer ambiguities: estimation and validation

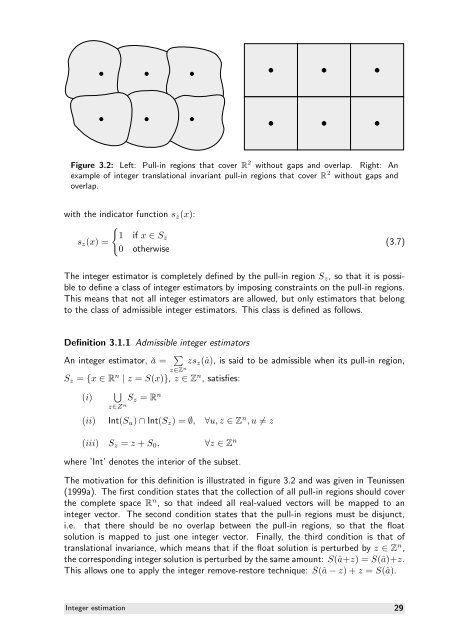
Figure 3.2: Left: Pull-in regions that cover R 2 without gaps and overlap. Right: An
example of integer translational invariant pull-in regions that cover R 2 without gaps and
overlap.
with the indicator function sz(x):
1 if x ∈ Sz
sz(x) =
0 otherwise
(3.7)
The integer estimator is completely defined by the pull-in region Sz, so that it is possible
to define a class of integer estimators by imposing constraints on the pull-in regions.
This means that not all integer estimators are allowed, but only estimators that belong
to the class of admissible integer estimators. This class is defined as follows.
Definition 3.1.1 Admissible integer estimators
An integer estimator, ǎ =
zsz(â), is said to be admissible when its pull-in region,
z∈Z n
Sz = {x ∈ Rn | z = S(x)}, z ∈ Zn , satisfies:
(i)
Sz = Rn z∈Z n
(ii) Int(Su) ∩ Int(Sz) = ∅, ∀u, z ∈ Z n , u = z
(iii) Sz = z + S0, ∀z ∈ Z n
where ’Int’ denotes the interior of the subset.
The motivation for this definition is illustrated in figure 3.2 and was given in Teunissen
(1999a). The first condition states that the collection of all pull-in regions should cover
the complete space R n , so that indeed all real-valued vectors will be mapped to an
integer vector. The second condition states that the pull-in regions must be disjunct,
i.e. that there should be no overlap between the pull-in regions, so that the float
solution is mapped to just one integer vector. Finally, the third condition is that of
translational invariance, which means that if the float solution is perturbed by z ∈ Z n ,
the corresponding integer solution is perturbed by the same amount: S(â+z) = S(â)+z.
This allows one to apply the integer remove-restore technique: S(â − z) + z = S(â).
Integer estimation 29