The GNSS integer ambiguities: estimation and validation

1.5
1
0.5
0
−0.5
−1
−1.5
−1.5 −1 −0.5 0 0.5 1 1.5
1.5
1
0.5
0
−0.5
−1
−1.5
−1.5 −1 −0.5 0 0.5 1 1.5
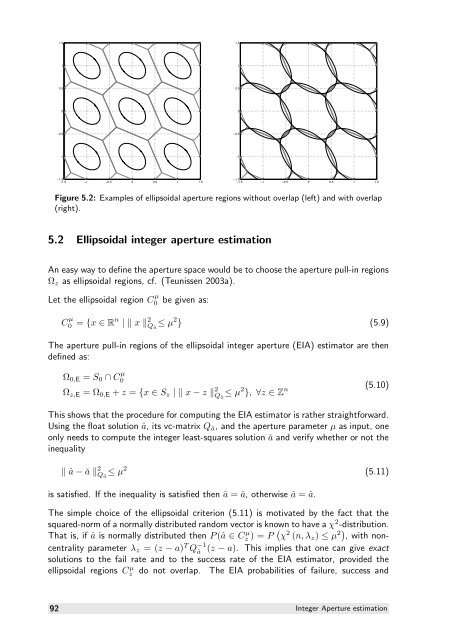
Figure 5.2: Examples of ellipsoidal aperture regions without overlap (left) and with overlap
(right).
5.2 Ellipsoidal integer aperture estimation
An easy way to define the aperture space would be to choose the aperture pull-in regions
Ωz as ellipsoidal regions, cf. (Teunissen 2003a).
Let the ellipsoidal region C µ
0
be given as:
C µ
0 = {x ∈ Rn | x 2 Qâ ≤ µ2 } (5.9)
The aperture pull-in regions of the ellipsoidal integer aperture (EIA) estimator are then
defined as:
Ω0,E = S0 ∩ C µ
0
Ωz,E = Ω0,E + z = {x ∈ Sz | x − z 2 Qâ ≤ µ2 }, ∀z ∈ Z n
(5.10)
This shows that the procedure for computing the EIA estimator is rather straightforward.
Using the float solution â, its vc-matrix Qâ, and the aperture parameter µ as input, one
only needs to compute the integer least-squares solution ǎ and verify whether or not the
inequality
â − ǎ 2 Qâ≤ µ2
is satisfied. If the inequality is satisfied then ā = ǎ, otherwise ā = â.
(5.11)
The simple choice of the ellipsoidal criterion (5.11) is motivated by the fact that the
squared-norm of a normally distributed random vector is known to have a χ2-distribution. That is, if â is normally distributed then P (â ∈ C µ z ) = P χ2 (n, λz) ≤ µ 2 , with non-
centrality parameter λz = (z − a) T Q −1
(z − a). This implies that one can give exact
â
solutions to the fail rate and to the success rate of the EIA estimator, provided the
ellipsoidal regions C µ z do not overlap. The EIA probabilities of failure, success and
92 Integer Aperture estimation