Residual Strength and Fatigue Lifetime of ... - Solid Mechanics

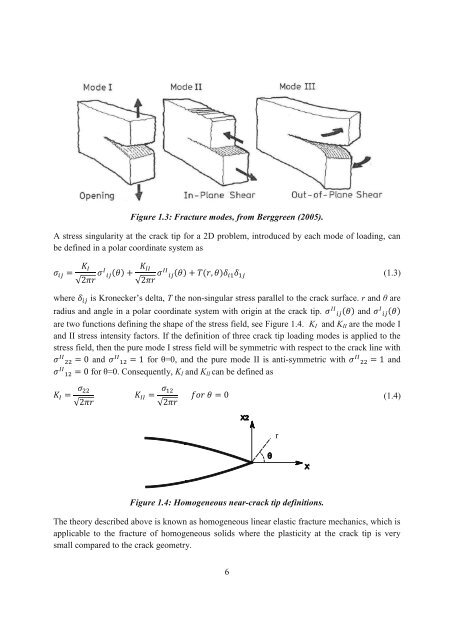
Figure 1.3: Fracture modes, from Berggreen (2005).
A stress singularity at the crack tip for a 2D problem, introduced by each mode of loading, can
be defined in a polar coordinate system as
where is Kronecker’s delta, T the non-singular stress parallel to the crack surface. r and are
radius and angle in a polar coordinate system with origin at the crack tip. and
are two functions defining the shape of the stress field, see Figure 1.4. KI and KII are the mode I
and II stress intensity factors. If the definition of three crack tip loading modes is applied to the
stress field, then the pure mode I stress field will be symmetric with respect to the crack line with
and for =0, and the pure mode II is anti-symmetric with and
for =0. Consequently, KI and KII can be defined as
(1.4)
Figure 1.4: Homogeneous near-crack tip definitions.
The theory described above is known as homogeneous linear elastic fracture mechanics, which is
applicable to the fracture of homogeneous solids where the plasticity at the crack tip is very
small compared to the crack geometry.
6
r
(1.3)