Rasmus ÿstergaard forside 100%.indd - Solid Mechanics

306 R.C. Østergaard, B.F. Sørensen
where
sin γ = χ √
UV.
I2
In Eq. (13) the only remaining unknown parameter is
the phase angel ω. The load-independent phase angle
ω depends on the material parameters ( and β) and
the geometry (η) but not on the load parameters P and
M.
In the general, case ω must be determined by numerical
means. In the present work, ω is determined using
a novel method called Crack Surface Displacement
Extrapolation (CSDE) method. The method calculates
mode mixity based on crack opening components found
with the Finite Element Method (FEM). The CSDE
method, which is described in detail in Appendix B,
has also been implemented in a parallel study (Berggren
et al. 2007).
Once ω(,β,η) is determined, the singularity at
the crack tip is characterized in terms of K . However,
from an experimental point of view, a more practical
measure of the singularity is comprised by the energy
release rate, G, together with the mode mixity, ψ, that
is related to K via (7). Combining (5), (6), (7) and (16),
while setting l = h, the mode mixity is expressed as
ψ = tan −1
ψ = ω, for M = 0,
λ sin ω − cos(ω + γ)
, for M = 0,
λ cos ω + sin(ω + γ)
(17)
where λ is a non-dimensional load parameter
V Ph
λ =
U M .
4 Results
4.1 Checking the CSDE method
The accuracy of the CSDE method is checked against
two problems for which mode mixity solutions exist.
A problem that has an analytical solution is a homogeneous
DCB specimen loaded by moments on the
one beam and on the uncracked end of the specimen
(Fig. 4a). The actual combination of moments gives the
exact mode mixity value ψ = tan −1 ( √ 3/4) ≈ 40.89 ◦
(Hutchinson and Suo 1992). Applying the CSDE method
to a FEM solution, we obtain ψ = 40.85 ◦ .
123
(a) (b)
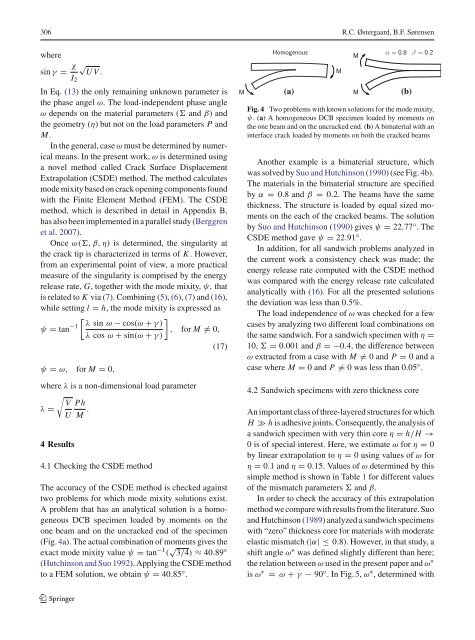
Fig. 4 Two problems with known solutions for the mode mixity,
ψ. (a) A homogeneous DCB specimen loaded by moments on
the one beam and on the uncracked end. (b) A bimaterial with an
interface crack loaded by moments on both the cracked beams
Another example is a bimaterial structure, which
was solved by Suo and Hutchinson (1990)(seeFig.4b).
The materials in the bimaterial structure are specified
by α = 0.8 and β = 0.2. The beams have the same
thickness. The structure is loaded by equal sized moments
on the each of the cracked beams. The solution
by Suo and Hutchinson (1990)givesψ = 22.77 ◦ .The
CSDE method gave ψ = 22.91 ◦ .
In addition, for all sandwich problems analyzed in
the current work a consistency check was made; the
energy release rate computed with the CSDE method
was compared with the energy release rate calculated
analytically with (16). For all the presented solutions
the deviation was less than 0.5%.
The load independence of ω was checked for a few
cases by analyzing two different load combinations on
the same sandwich. For a sandwich specimen with η =
10, = 0.001 and β =−0.4, the difference between
ω extracted from a case with M = 0 and P = 0 and a
case where M = 0 and P = 0 was less than 0.05 ◦ .
4.2 Sandwich specimens with zero thickness core
An important class of three-layered structures for which
H ≫ h is adhesive joints. Consequently, the analysis of
a sandwich specimen with very thin core η = h/H →
0 is of special interest. Here, we estimate ω for η = 0
by linear extrapolation to η = 0 using values of ω for
η = 0.1 and η = 0.15. Values of ω determined by this
simple method is shown in Table 1 for different values
of the mismatch parameters and β.
In order to check the accuracy of this extrapolation
method we compare with results from the literature. Suo
and Hutchinson (1989) analyzed a sandwich specimens
with “zero” thickness core for materials with moderate
elastic mismatch (|α| ≤0.8). However, in that study, a
shift angle ω ∗ was defined slightly different than here;
the relation between ω used in the present paper and ω ∗
is ω ∗ = ω + γ − 90 ◦ .InFig.5, ω ∗ , determined with