Rasmus ÿstergaard forside 100%.indd - Solid Mechanics

314 R.C. Østergaard, B.F. Sørensen
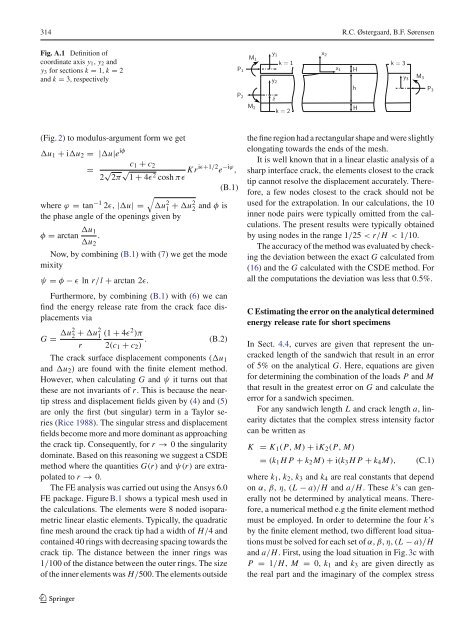
Fig. A.1 Definition of
coordinate axis y1, y2 and
y3 for sections k = 1, k = 2
and k = 3, respectively
(Fig. 2) to modulus-argument form we get
u1 + iu2 = |u|e iφ
c1 + c2
=
2 √ 2π √ 1 + 4ɛ2 cosh πɛ Kriɛ+1/2e −iϕ ,
(B.1)
where ϕ = tan−1
2ɛ, |u| = u2 1 + u2 2 and φ is
the phase angle of the openings given by
φ = arctan u1
.
u2
Now, by combining (B.1) with (7) we get the mode
mixity
ψ = φ − ɛ ln r/l + arctan 2ɛ.
Furthermore, by combining (B.1) with (6) we can
find the energy release rate from the crack face displacements
via
G = u2 2 + u2 1
r
(1 + 4ɛ2 )π
. (B.2)
2(c1 + c2)
The crack surface displacement components (u1
and u2) are found with the finite element method.
However, when calculating G and ψ it turns out that
these are not invariants of r. This is because the neartip
stress and displacement fields given by (4) and (5)
are only the first (but singular) term in a Taylor series
(Rice 1988). The singular stress and displacement
fields become more and more dominant as approaching
the crack tip. Consequently, for r → 0 the singularity
dominate. Based on this reasoning we suggest a CSDE
method where the quantities G(r) and ψ(r) are extrapolated
to r → 0.
The FE analysis was carried out using the Ansys 6.0
FE package. Figure B.1 shows a typical mesh used in
the calculations. The elements were 8 noded isoparametric
linear elastic elements. Typically, the quadratic
fine mesh around the crack tip had a width of H/4 and
contained 40 rings with decreasing spacing towards the
crack tip. The distance between the inner rings was
1/100 of the distance between the outer rings. The size
of the inner elements was H/500. The elements outside
123
P1
P2
M1
M2
y1
y2
δ
k = 1
k = 2
x2
x1
H
h
H
k = 3
the fine region had a rectangular shape and were slightly
elongating towards the ends of the mesh.
It is well known that in a linear elastic analysis of a
sharp interface crack, the elements closest to the crack
tip cannot resolve the displacement accurately. Therefore,
a few nodes closest to the crack should not be
used for the extrapolation. In our calculations, the 10
inner node pairs were typically omitted from the calculations.
The present results were typically obtained
by using nodes in the range 1/25 < r/H < 1/10.
The accuracy of the method was evaluated by checking
the deviation between the exact G calculated from
(16) and the G calculated with the CSDE method. For
all the computations the deviation was less that 0.5%.
C Estimating the error on the analytical determined
energy release rate for short specimens
In Sect. 4.4, curves are given that represent the uncracked
length of the sandwich that result in an error
of 5% on the analytical G. Here, equations are given
for determining the combination of the loads P and M
that result in the greatest error on G and calculate the
error for a sandwich specimen.
For any sandwich length L and crack length a, linearity
dictates that the complex stress intensity factor
can be written as
K = K1(P, M) + iK2(P, M)
= (k1HP+ k2M) + i(k3HP+ k4M), (C.1)
where k1, k2, k3 and k4 are real constants that depend
on α, β, η, (L − a)/H and a/H. These k’s can generally
not be determined by analytical means. Therefore,
a numerical method e.g the finite element method
must be employed. In order to determine the four k’s
by the finite element method, two different load situations
must be solved for each set of α, β, η, (L − a)/H
and a/H. First, using the load situation in Fig. 3c with
P = 1/H, M = 0, k1 and k3 are given directly as
the real part and the imaginary of the complex stress
y3
M3
P3