Entropy Coherent and Entropy Convex Measures of Risk - Eurandom
Entropy Coherent and Entropy Convex Measures of Risk - Eurandom
Entropy Coherent and Entropy Convex Measures of Risk - Eurandom
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
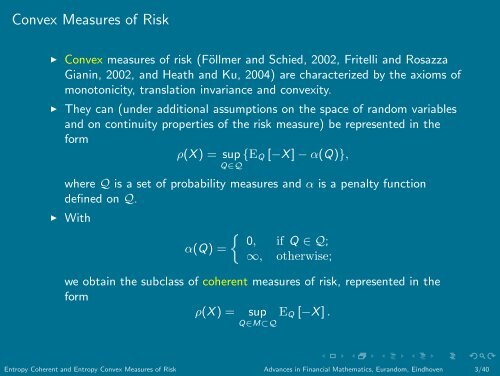
<strong>Convex</strong> <strong>Measures</strong> <strong>of</strong> <strong>Risk</strong><br />
◮ <strong>Convex</strong> measures <strong>of</strong> risk (Föllmer <strong>and</strong> Schied, 2002, Fritelli <strong>and</strong> Rosazza<br />
Gianin, 2002, <strong>and</strong> Heath <strong>and</strong> Ku, 2004) are characterized by the axioms <strong>of</strong><br />
monotonicity, translation invariance <strong>and</strong> convexity.<br />
◮ They can (under additional assumptions on the space <strong>of</strong> r<strong>and</strong>om variables<br />
<strong>and</strong> on continuity properties <strong>of</strong> the risk measure) be represented in the<br />
form<br />
ρ(X) = sup {EQ [−X] − α(Q)},<br />
Q∈Q<br />
where Q is a set <strong>of</strong> probability measures <strong>and</strong> α is a penalty function<br />
defined on Q.<br />
◮ With<br />
α(Q) =0, if Q ∈ Q;<br />
∞, otherwise;<br />
we obtain the subclass <strong>of</strong> coherent measures <strong>of</strong> risk, represented in the<br />
form<br />
ρ(X) = sup<br />
Q∈M⊂Q<br />
EQ [−X] .<br />
<strong>Entropy</strong> <strong>Coherent</strong> <strong>and</strong> <strong>Entropy</strong> <strong>Convex</strong> <strong>Measures</strong> <strong>of</strong> <strong>Risk</strong> Advances in Financial Mathematics, Eur<strong>and</strong>om, Eindhoven 3/40





![The Contraction Method on C([0,1]) and Donsker's ... - Eurandom](https://img.yumpu.com/19554492/1/190x143/the-contraction-method-on-c01-and-donskers-eurandom.jpg?quality=85)








