Mathematical Modelling of Granulation: Static and Dynamic Liquid ...

P. Rynhart et al., Mathematical Modelling of Granulation 205
Neglecting inertial terms, that is assuming Re ≪ 1, the momentum equations from [11] reduce to
0=− ∂P
∂r + µ ∂2vr 1 ∂vr
+
∂r2 r ∂r + ∂2vr ∂z
0=− ∂P
∂z − ρg + µ ∂2vz 1
+
∂r2 r
r 2
2 − vr
∂vz
∂r + ∂2vz ∂z2 where P = P (r, z) is the pressure difference between the inside and outside of the liquid bridge, defined
to be positive when the pressure is higher internally. To make progress on this problem, an approximation
that vz ≪ vr is introduced. Physically this means that the bridges must have a small volume, and that a
small gap distance h must separate the particles when compared to the volume and radius of a fundamental
particle with radius R. Since vz ≪ vr, and because gravity is not considered in this approximation, only
equation (15) is applicable to the solution.
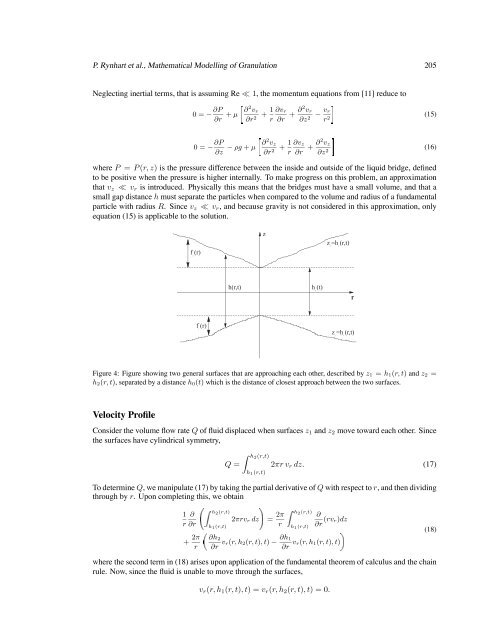
Figure 4: Figure showing two general surfaces that are approaching each other, described by z1 = h1(r, t) and z2 =
h2(r, t), separated by a distance h0(t) which is the distance of closest approach between the two surfaces.
Velocity Profile
Consider the volume flow rate Q of fluid displaced when surfaces z1 and z2 move toward each other. Since
the surfaces have cylindrical symmetry,
Q =
h2(r,t)
h1(r,t)
(15)
(16)
2πr vr dz. (17)
To determine Q,wemanipulate (17) by taking the partial derivative of Q with respect to r,andthen dividing
through by r. Upon completing this, we obtain
1
r
∂
∂r
+ 2π
r
Z h2(r,t)
h1(r,t)
∂h2
∂r
! 2π
2πrvr dz =
r
Z h2(r,t)
h1(r,t)
∂
∂r (rvr)dz
∂h1
vr(r, h2(r, t),t) − vr(r, h1(r, t),t)
∂r
where the second term in (18) arises upon application of the fundamental theorem of calculus and the chain
rule. Now, since the fluid is unable to move through the surfaces,
vr(r, h1(r, t),t)=vr(r, h2(r, t),t)=0.
(18)