Notes with answers - Summit Schools

2.1 

Your Notes 

Functions and Their Graphs 

Goal p Identify and graph functions. 

Vocabulary 

Relation A mapping, or pairing, of input values 

with output values 

Domain The set of input values of a relation 

Range The set of output values of a relation 

Function A relation for which each input has 

exactly one output 

Equation in two variables An equation that contains 

two variables 

Independent variable The input variable in an 

equation in two variables 

Dependent variable The output variable in an 

equation in two variables 

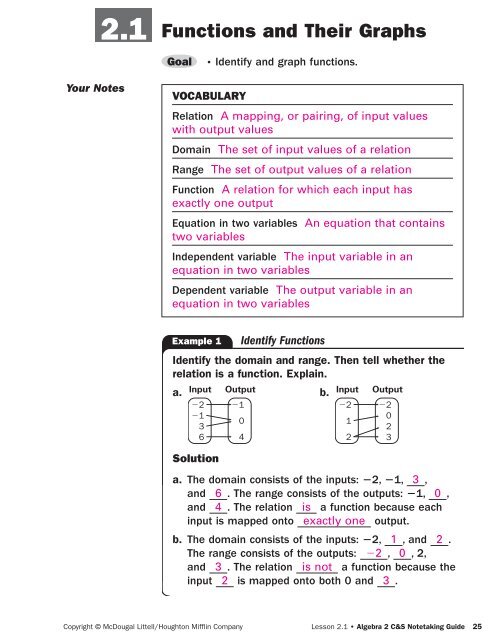
Example 1 Identify Functions 

Identify the domain and range. Then tell whether the 

relation is a function. Explain. 

a. 

Input Output 

22 21 

21 

3 

0 

6 4 

Solution 

b. 

Input Output 

22 22 

0 

1 

2 

2 3 

a. The domain consists of the inputs: 22, 21, 3 , 

and 6 . The range consists of the outputs: 21, 0 , 

and 4 . The relation is a function because each 

input is mapped onto exactly one output. 

b. The domain consists of the inputs: 22, 1 , and 2 . 

The range consists of the outputs: 22 , 0 , 2, 

and 3 . The relation is not a function because the 

input 2 is mapped onto both 0 and 3 . 

Copyright © McDougal Littell/Houghton Mifflin Company Lesson 2.1 • Algebra 2 C&S Notetaking Guide 25


VErTical linE TEST For FuncTionS 

A relation is a function if and only if no vertical line 

intersects the graph at more than one point . 

Function Not a function 

1 

O 

y 

1 

x 

Example 2 Apply the Vertical Line Test 

Graph the relations from Example 1. Use the vertical 

line test to tell whether the relations are functions. 

a. First, write the relation 

as a set of ordered 

pairs. 

(–2, –1), (–1, 0) , 

(3, 0), (6, 4) 

Then plot the points. 

1 

O 

y 

1 

No vertical line 

contains more 

than one point so the 

relation is a function . 

x 

1 

O 

y 

1 

b. First, write the relation 

as a set of ordered 

pairs. 

(–2, –2) , (1, 0), 

(2, 2) , (2, 3) 

Then plot the points. 

1 

O 

y 

1 

The vertical line at 

x 5 2 contains two 

different points in the 

relation. So, the relation 

is not a function. 

26 Lesson 2.1 • Algebra 2 C&S Notetaking Guide Copyright © McDougal Littell/Houghton Mifflin Company 

x 

x


Homework 

GraphinG EquaTionS in Two VariablES 

Step 1 Make a table of values. Write the ordered pairs . 

Step 2 Plot enough solutions to recognize a pattern . 

Step 3 Connect the points with a line or a curve . 

Example 3 Graph an Equation 

Graph y 5 22x 2 2. 

Solution 

1. Make a table of values . Write the ordered pairs . 

Choose x 22 21 0 1 2 

Evaluate y 2 0 22 24 26 

(x, y): (22, 2 ), (21, 0 ), (0, 22 ), (1, 24 ), (2, 26 ) 

2. Plot the points. Notice that all 

the points lie on a line . 

3. Connect the points with a 

line . Observe that the 

graph represents a function . 

Checkpoint Complete the following exercises. 

1. Graph y 5 x 1 2. 2. Graph y 5 3x 2 1. 

1 

O 

y 

1 

Copyright © McDougal Littell/Houghton Mifflin Company Lesson 2.1 • Algebra 2 C&S Notetaking Guide 27 

x 

1 

O 

y 

1 

1 

O 

y 

1 

x 

x

2.2 


linear Functions and Function 

notation 

Goal p Identify, evaluate, and graph linear functions. 

Vocabulary 

Linear function A function that can be written in the 

form y 5 mx 1 b where m and b are constants 

Function notation Using f(x) to represent the 

dependent variable of a function 

Example 1 Identify a Linear Function 

Tell whether the function is linear. Explain. 

a. f(x) 5 2x3 1 6 2 x b. g(x) 5 9 1 4x 

a. f is not a linear function because it has an 

x 3-term . 

b. g is a linear function because it can be rewritten as 

g(x) 5 4x 1 9 , so m 5 4 and b 5 9 . 

Checkpoint Tell whether the function is linear. Explain. 

1. f(x) 5 6x 1 10 

Yes; it is of the form 

y 5 mx 1 b. 

Example 2 Evaluate a Function 

Evaluate the function when x 5 3. 

2. g(x) 5 2x2 1 4x 2 1 

No; it is not of the 

form y 5 mx 1 b. 

a. f(x) 5 22x 1 5 b. g(x) 5 x2 2 x 2 1 

a. f (x) 5 22x 1 5 Write original function. 

f ( 3 ) 5 22( 3 ) 1 5 Substitute 3 for x. 

5 26 1 5 Multiply. 

5 21 Simplify. 

28 Lesson 2.2 • Algebra 2 C&S Notetaking Guide Copyright © McDougal Littell/Houghton Mifflin Company


Homework 

b. g (x) 5 x 2 2 x 2 1 Write original function. 

g( 3 ) 5 ( 3 ) 2 2 3 2 1 Substitute 3 for x. 

5 9 2 3 2 1 Multiply. 

5 5 Simplify. 

Checkpoint Evaluate the function when x 5 24. 

3. f(x) 5 2x 2 3 4. g(x) 5 2x 2 1 3x 

211 20 

Example 3 Graph a Linear Function 

Graph f(x) 5 2x 1 2. 

Solution 

Rewrite the function as y 5 2x 1 2. Find a point on 

the graph by substituting a convenient value for x . 

y 5 2x 1 2 Write equation. 

y 

y 5 2( 0 ) 1 2 Substitute 0 for x. 

y 5 2 Simplify. 

1 

O 1 

One point is (0, 2) . Find a second 

point. 

y 5 2(1) 1 2 Substitute 1 for x . 


A second point is (1, 1) . Draw a line through the 

two points. 

Checkpoint Complete the following exercise. 

5. Graph h(x) 5 22x 1 1. 


1 

O 

y 

1 

x 

x

2.3 


When calculating 

the slope, be sure 

to subtract the 

x‑coordinates and 

the y‑coordinates 

in the correct order. 

A vertical line has 

“undefined slope” 

because for any 

two points, the 

slope formula’s 

denominator 

becomes 0, and 

division by 0 is 

undefined. 

Slope and rate of change 

Goal p Find and use the slope of a line. 

Vocabulary 

Slope For a nonvertical line, the ratio of the line’s 

vertical change to its horizontal change 

ThE SlopE oF a linE 

The slope m of the nonvertical line passing through 

the points (x1 , y1 ) and (x2 , y2 ) is given by the formula 

m 5 y2 2 y1 } . 

x2 2 x1 Example 1 Find the Slope of a Line 

Find the slope of the line through (1, 3) and (6, 7). 

Solution 

Let (x 1 , y 1 ) 5 (1, 3) and (x 2 , y 2 ) 5 (6, 7) . 

m 5 y2 2 y1 } 5 

x2 2 x1 7 2 3 

} 

6 2 1 

The slope of the line is 

4 

5 } 

5 

4 

} . 

5 

claSSiFyinG linES by SlopE 

Positive Negative Zero Undefined 

O 

y 

x 

O 

y 

Line rises from Line falls from Line is Line is 

left to right. left to right . horizontal . vertical . 


x 

O 

y 

x 

O 

y 

x


Example 2 Classify Lines Using Slope 

Without graphing, tell whether the line through the 

given points rises, falls, is horizontal, or is vertical. 

a. (26, 22), (1, 3) b. (2, 21), (2, 2) 

Solution 

a. m 5 

b. m 5 

3 2 (22) 

} 

1 2 (26) 

2 2 (21) 

} 

2 2 2 

5 5 

} 

7 Because m > 0, the line rises . 

5 3 

} 

0 Because m is undefined , the 

line is vertical . 


1. Find the slope of the line through (4, 2) and (7, 9). 

7 

} 

3 

2. Without graphing, tell whether the line through the 

points (23, 2) and (1, 4) rises, falls, is horizontal, or 

is vertical. 

rises 

Example 3 Compare Steepness of Lines 

Tell which line is steeper. 

Line 1: through (2, 3) and (21, 4) 


Solution 

Slope of line 1: m 5 

Slope of line 2: m 5 

4 2 3 

} 

21 2 2 

25 2 (22) 

} 

24 2 (27) 

5 1 

} 

23 

or 2 1 

} 

3 

5 23 

} 

3 

5 21 

Both lines have negative slope. 

Because |m1 | > |m2 |, line 1 is steeper than 

line 2 . 



Homework 

Checkpoint Tell which line is steeper. 

3. Line 1: through (3, 5) and (6, 8) 


line 1 

Example 4 Slope as a Rate of Change 

census The population of Smalltown was 10,000 in 

2001 and 12,500 in 2006. Find the average rate of 

change in the population. 

Solution 

Let (x1 , y1 ) 5 (2001, 10,000 ) and 

(x2 , y2 ) 5 ( 2006 , 12,500 ). 

Change in population 

Average rate of change 5 }} 

Change in time 

12,500 2 10,000 

5 }} 

2006 2 2001 

5 2500 

} 

5 

5 500 

The average rate of change is 500 people per year . 


4. Census Suppose the population of Smalltown will be 

20,000 in 2011. Use the information in Example 4 

to find the average rate of change in the population 

from 2001 to 2011. 

1000 people per year 



2.4 quick Graphs of linear 

Equations 

Goal p Use slope-intercept form and standard form to 

graph equations. 

Vocabulary 

y-intercept The y-coordinate of a point where a 

graph intersects the y-axis 

Slope-intercept form A linear equation written in the 

form y 5 mx 1 b where m is the slope and b is the 

y-intercept of the equation’s graph 

x-intercept The x-coordinate of a point where a 

graph intersects the x-axis 

Standard form of a linear equation A linear equation 

written in the form Ax 1 By 5 C where A and B are 

not both zero 

GraphinG EquaTionS in SlopE-inTErcEpT Form 

Step 1 Write the equation in slope-intercept form by 

solving for y . 

Step 2 Find the y-intercept and plot the 

corresponding point. 

Step 3 Find the slope and use it to plot a second 

point on the line. 

Step 4 Draw a line through the two points . 



Example 1 Use Slope-Intercept Form to Graph a Line 

Graph y 1 3 } x 5 1. 

2 

solution 

1. Solve the equation for y by subtracting 3 

} x from each 

2 

side: 

y 5 1 2 3 

} x or y 5 2 

2 3 

} x 1 1 

2 

2. Find the y-intercept. Comparing y 5 2 3 

} x 1 1 to 

2 

y 5 mx 1 b, you can see that b 5 1 . Plot a point 

at ( 0 , 1 ). 

3. Find the slope. The slope is m 5 2 3 

} , so 

2 rise 

} 

run 

From (0, 1), move down 3 units and right 

2 units. Plot a second point at ( 2 , 22 ). 

4. Draw a line through the two points. 

1 

O 

y 

1 

x 

GraphinG Equations in standard Form 

Step 1 Write the equation in standard form . 

Step 2 Find the x-intercept by letting y 5 0 and 

solving for x . Plot the corresponding point. 

Step 3 Find the y-intercept by letting x 5 0 and 

solving for y . Plot the corresponding point. 

Step 4 Draw a line through the two points . 

5 23 

} 

2 . 



Example 2 Draw Quick Graphs 

Graph 4x 1 5y 5 20. 

1. The equation is already in standard form . 

2. Find the x-intercept. 4x 1 5( 0 ) 5 20 Let y 5 0. 

SlopES oF parallEl and pErpEndicular linES 

Consider two different 

nonvertical lines l 1 and l 2 

with slopes m 1 and m 2 . 

Two lines are parallel if and only 

if they have the same slope . 

m 1 5 m 2 

Two lines are perpendicular if and 

only if their slopes are negative 

reciprocals of each other. 

m 1 5 2 1 

} 

m 2 or m 1 • m 2 5 –1 

x 5 5 Solve for x. 

The x-intercept is 5 , so plot the point (5, 0) . 

3. Find the y-intercept. 4( 0 ) 1 5y 5 20 Let x 5 0 . 

y 5 4 Solve for y. 

The y-intercept is 4 , so plot the point (0, 4) . 

4. Draw a line through 

the intercepts. 


l 1 

l 1 

1 

O 

l 2 

y 

1 

O 

1 

1 

O 

y 

y 

1 

1 

l 2 

x 

x 

x


Homework 

Example 3 Graph Parallel and Perpendicular Lines 

a. Draw the graph y 5 2x 2 3. 

b. Graph the line that passes through (0, 2) and is 

parallel to the graph of y 5 2x 2 3. 

c. Graph the line that passes through (0, 2) and is 

perpendicular to the graph of y 5 2x 2 3. 

Solution 

a. Draw the graph y 5 2x 2 3 

using the y-intercept 23 and 

slope 2 . 

b. Graph the point (0, 2). Any 

line parallel to the graph of 

y 5 2x 2 3 has the same 

slope, 2 . From (0, 2), move 

up 2 units and right 

1 unit. Plot a second point at (1, 4) . Draw a line 

through the two points. 

c. The slope of any line perpendicular to the 

graph of y 5 2x 2 3 is the negative reciprocal 

of 2, or 2 1 

} . So, from (0, 2), move down 1 unit 

2 

and right 2 units. Plot a second point at 

(2, 1) . Draw the line. 

Checkpoint On the same grid, draw each graph. 

1. y 5 2 1 } 

3 x 1 1 

2. The line parallel to 

y 5 2 1 } 

3 x 1 1 through 

the point (0, 4) 

3. The line perpendicular 

to y 5 2 1 } 

3 x 1 1 through 

the point (0, 4) 


c 

1 

O 

y 

1 

1 

O 

Ex. 3 

y 

1 

b 

Ex. 1 

Ex. 2 

x 

a 

x


2.5 writing Equations of lines 

Goal p Write linear equations. 

Vocabulary 

Point-slope form An equation of a line written in 

the form y 2 y 1 5 m(x 2 x 1 ) where the line passes 

through the point (x 1 , y 1 ) and has a slope of m 

wriTinG an EquaTion oF a linE 

Slope-Intercept Form Given the slope m and the 

y-intercept b , use slope-intercept form: y 5 mx 1 b . 

Point-Slope Form Given the slope m and a point 

(x1 , y1 ) , use point-slope form: y 2 y1 5 m(x 2 x1 ) . 

Example 1 Write an Equation Given Slope and y-Intercept 

Write an equation of the 

line shown. 

y 

Solution 

1 

From the graph, you can see 

that the slope is m 5 2 

O 2 

3 

} 

2 

. 

Because the line intersects the 

y-axis at ( 0, 1 ), the y-intercept 

is b 5 1 . 

Use the slope-intercept form to write an equation of 

the line. 

y 5 mx 1 b Use slope-intercept form. 

y 5 2 3 

} 

2 

x 1 1 Substitute 2 3 

} 

2 

for m and 1 for b. 


x


Example 2 Write an Equation Given Slope and a Point 

Write an equation of the line that passes through (2, 1) 

and has a slope of 2. 

Solution 

Because you know the slope and a point on the line, 

use the point-slope form with (x1 , y1 ) 5 (2, 1) and 

m 5 2 . 

y 2 y1 5 m(x 2 x1 ) Use point-slope form. 

y 2 1 5 2 (x 2 2 ) Substitute for m, x1 , and y1 . 

Rewrite the equation in slope-intercept form. 

y 2 1 5 2x 2 4 Distributive property 

y 5 2x 2 3 Slope-intercept form 

Checkpoint Write an equation of the line. 

1. y 

2. The line through (1, 5) 

1 

with a slope of 22 

O 

1 

x 

y 5 2 

} 

3 x 2 3 y 5 22x 1 7 

Example 3 Write Equations of Parallel Lines 

Write an equation of the line that passes through 

(21, 1) and is parallel to the line y 5 22x 1 3. 

Solution 

The given line has a slope of 22 . Any line parallel 

to this line must also have a slope of 22 . Use 

point-slope form with (x1 , y1 ) 5 (21, 1) and 

m 5 22 to write the equation of the line. 



Homework 

y 2 y 1 5 m(x 2 x 1 ) Use point-slope form. 

y 2 1 5 22 [x 2 (21) ] Substitute 1 for y 1 , 22 

for m, and 21 for x 1 . 

y 2 1 5 22x 2 2 Distributive property 

y 5 22x 2 1 Slope-intercept form 

Example 4 Write Equations of Perpendicular Lines 

Write an equation of the line that passes through 

(23, 5) and is perpendicular to the line y 5 4x 2 1. 

solution 

The given line has a slope of 4 . The slope of any 

line perpendicular to this line will be the negative 

reciprocal of 4 , which is 2 1 

} . 

4 

Use point-slope form with (x1 , y1 ) 5 (23, 5) and 

m 5 2 1 

} to write the equation of the line. 

4 

y 2 y 1 5 m(x 2 x 1 ) Use point-slope form. 

y 2 5 5 2 1 

} [x 2 (23) ] 

4 

Substitute 5 for y1 , 2 1 

} 

4 

for m, and 23 for x1 . 

1 

y 2 5 5 2 } x 2 

4 3 

} 

4 

Distributive property 

1 

y 5 2 } x 1 

4 17 

} 

4 

Checkpoint Write an equation of the line. 

3. The line through 

(22, 3) parallel to 

y 5 4x 2 6 

y 5 4x 1 11 

Slope-intercept form 

4. The line through 

(22, 3) perpendicular 

to y 5 4x 2 6 

y 5 2 1 

} x 1 

4 5 

} 

2 



2.6 direct Variation 

Goal p Write and graph direct variation equations. 

Vocabulary 

Direct variation Two variables x and y show direct 

variation provided that y 5 kx where k is a nonzero 

constant. 

Constant of variation The nonzero constant k in a 

direct variation equation y 5 kx 

Example 1 Graph a Direct Variation Equation 

Graph y 5 5 

} x. 

3 

Solution 

Plot a point at the origin . 

Find a second point by substituting 

a convenient value for x . 

y 5 5 } x 

3 

Write equation. 

y 5 5 } ( 3 ) 

3 

Substitute 3 for x. 


A second point is (3, 5) . 

Plot the second point. Then draw a line through the 

two points. 

Checkpoint Graph the equation. 

1. y 5 23x 

1 

O 

y 

1 


x 

1 

O 

y 

1 

x


The value of k is 

called the spring 

constant. This 

constant is unique 

for each spring. 

Example 2 Write a Direct Variation Equation 

physics According to Hooke’s law, the amount that a 

spring is stretched varies directly with the force that is 

applied to the spring. If 64 pounds of force F stretches 

a spring a distance d of 8 inches, write an equation 

so that F varies directly with d. How many pounds of 

force are needed to stretch the spring to a distance 

of 14 inches? 

Solution 

Find the constant of variation. 

F 5 kd Write a direct variation equation. 

64 5 k( 8 ) Substitute 64 for F and 8 for d. 

8 5 k Simplify. 

An equation is F 5 8d . To find how many pounds 

of force is needed to stretch the spring 14 inches, 

substitute 14 for d. 

F 5 8 ( 14 ) 5 112 

A force of 112 pounds will stretch the spring 14 inches. 


2. Suppose that a force of 92 pounds is applied to 

the spring discussed in Example 2. How far will 

the spring be stretched? 

11.5 inches 



Homework 

Example 3 Identify Direct Variation 

The table gives sample cell phone bills, showing the 

total monthly cost and the number of minutes used 

per month. Tell whether total cost and the number of 

minutes show direct variation. If so, write an equation 

relating c and m. 

Total cost, c (dollars) 35 45 80 15 28 

Minutes used, m 100 129 229 43 80 

solution 

Find the ratio of c to m for each data pair. 

35 

} 

100 

15 

} 

43 

5 0.35 45 

} 

129 

28 

< 0.35 } 5 0.35 

30 


3. Tell whether the data (21, 22), (1, 2), (3, 6), 

(5, 10), (7, 14) show direct variation. If so, write an 

expression relating x and y. 

direct variation; y 5 2x 

80 

< 0.35 } < 0.35 

229 

The ratio of c to m in each pair is nearly the same , so 

the data show direct variation . Substituting 0.35 

for k in c 5 km gives the direct variation equation 

c 5 0.35 m, which relates the total cost to number of 

minutes used. 


2.7 


Scatter plots and correlation 

Goal p See correlation in a scatter plot and find a 

best-fitting line. 

Vocabulary 

Scatter plot A graph of a set of data pairs (x, y) 

used to determine whether there is a relationship 

between the variables x and y 

Positive correlation A set of data pairs (x, y) for 

which as x increases, y tends to increase 

Negative correlation A set of data pairs (x, y) for 

which as x increases, y tends to decrease 

Relatively no correlation A set of data pairs (x, y) for 

which there is no obvious pattern between x and y 

Best-fitting line The line that most closely models 

the data shown in a scatter plot 

Example 1 Identify Correlation 

Describe the correlation shown by each plot. 

a. y 

b. 

1 

O 

1 

x 

Solution 

a. The scatter plot shows a negative correlation: as 

x increases, y decreases . 

b. The scatter plot shows a positive correlation: as 

x increases , y also increases . 


1 

O 

y 

1 

x


Be sure that about 

the same number 

of points lie above 

your best-fitting 

line as lie below it. 

approximatinG a BEst-FittinG LinE 

Step 1 Draw a scatter plot of the data . 

Step 2 Sketch a line that follows the trend of the 

data points. 

Step 3 Choose two points that appear to lie on the 

line, and estimate their coordinates . 

Step 4 Write an equation of the line that passes 

through the two points from Step 3. This 

equation gives a model for the data. 

Example 2 Find a Best-Fitting Line 

Football attendance The table gives the number of 

people y who attended each of the first seven football 

games x of the season. Approximate the best-fitting 

line for the data. 

x 1 2 3 4 5 6 7 

y 722 763 772 826 815 857 897 

solution 

1. Draw a scatter plot. 

950 

y 

2. Sketch the line that 

900 

best fits the data. 

850 

800 

3. Choose two points on the 

line. For this scatter plot you 

750 

700 

650 

might choose (1, 725) and 

0 

0 1 2 3 4 5 6 7 x 

( 2 , 750). 

Football game 

4. Write an equation of the line. First find the slope using 

the two points: 

750 2 725 25 

m 5 } 5 } 5 25 

2 2 1 1 

Now use point-slope form. Choose (x1 , y1 ) 5 (1, 725). 

y 2 y 1 5 m(x 2 x 1 ) Point-slope form 

y 2 725 5 25 (x 2 1 ) Substitute for y 1 , m, and x 1 . 

Number of people 

y 5 25x 1 700 Solve for y. 



Homework 

Example 3 Use a Best-Fitting Line 

Use the equation of the best-fitting line from Example 2 

to predict the number of people that will attend the 

tenth football game. 

Solution 

Substitute 10 for x in the equation from Example 2. 

y 5 25( 10 ) 1 700 5 250 1 700 5 950 

You can predict that 950 people will attend the game. 


For each scatter plot, tell whether the data show a 

positive correlation, negative correlation, or relatively no 

correlation. 

1. y 

2. y 

1 

O 

1 

positive correlation 

x 

1 

O 

1 

relatively no correlation 

3. School The table gives the average class score y on 

each test for the first six chapters x of the textbook. 

x 1 2 3 4 5 6 

y 84 83 86 88 87 90 

a. Approximate the best-fitting 

line for the data. 

y 5 2x 1 80 

b. Use your equation to predict 

the test score for the 9th test 

that the class will take. 

98 

Average class score 

90 

88 

86 

84 

82 

y 

0 

0 1 2 3 4 5 

Test 


x 

6 

x

words to review 

use your own words and/or an example to explain 

the vocabulary word. 

Relation 

A mapping, or pairing, 

of input values with 

output values 

Range 

The set of output 

values in a relation 

Equation in two variables 

y 5 2x 2 5 

Dependent variable 

The dependent variable 

in the equation 

y 5 2x 2 5 is y. 

Function notation 

f(x) 5 2x 1 4 

y-intercept 

The y-intercept of 

y 5 2x 1 4 is 4. 

x-intercept 

The x-intercept of 

y 5 2x 1 4 is 22. 

Domain 

The set of input values 

in a relation 

Function 

A relation for which 

each input has exactly 

one output 

Independent variable 

The independent 

variable in the equation 

y 5 2x 2 5 is x. 

Linear function 

A function that can be 

written in the form 

y 5 mx 1 b where m 

and b are constants 

Slope 

The slope of the line 

passing through (2, 6) 

and (5, 9) is 

m 5 y 2 2 y 1 

} 

x 2 2 x 1 

5 

9 2 6 

} 

5 2 2 

5 3 

} 

3 5 1. 

Slope-intercept form 

y 5 2x 1 4 

Standard form of a linear 

equation 

2x 1 4y 5 7 

46 Words to Review • Algebra 2 C&S Notetaking Guide Copyright © McDougal Littell/Houghton Mifflin Company

Point-slope form 

y 2 1 5 2(x 2 5) 

Constant of variation 

The constant of 

variation in the direct 

variation equation 

y 5 6x is 6. 

Positive correlation 

This scatter plot shows 

a positive correlation. 

y 

Relatively no correlation 

This scatter plot 

shows relatively no 

correlation. 

y 

x 

x 

Direct variation 

y 5 6x 

Scatter plot 

A graph of a set of 

data pairs (x, y) used 

to determine whether 

there is a relationship 

between the variables 

x and y 

Negative correlation 

This scatter plot shows 

a negative correlation. 

y 

Best-fitting line 

The line that most 

closely models the data 

shown in a scatter plot 

review your notes and chapter 2 by using the 

chapter review on pages 115–118 of your textbook. 

Copyright © McDougal Littell/Houghton Mifflin Company Words to Review • Algebra 2 C&S Notetaking Guide 47 

x

Notes with answers - Summit Schools

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?