Hyperbolic Functions
Hyperbolic Functions
Hyperbolic Functions
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<strong>Hyperbolic</strong> <strong>Functions</strong><br />
The trigonometric functions cos α and cos α are defined using the unit circle x2 +y 2 = 1<br />
by measuring the distance α in the counter-clockwise direction along the circumference<br />
of the circle. The area of the sector so determined is α<br />
, so we can equivalently say that<br />
2<br />
cos α and cos α are derived from the unit circle x2 + y 2 = 1 by measuring off a sector<br />
(shaded red )of area α<br />
. The other four trigonometric functions can then be defined in<br />
2<br />
terms of cos and sin.<br />
Similarly, we may define hyperbolic functions cosh α and sinh α from the “unit hyperbola”<br />
x2 −y 2 = 1 by measuring off a sector (shaded red )of area α<br />
to obtain a point P whose<br />
2<br />
x- and y- coordinates are defined to be cosh α and sinh α.<br />
y<br />
x<br />
y<br />
(cosh α, sinh α)<br />
Since at this point we do not yet know how to compute the areas of most curved regions,<br />
we must take it on faith that the six hyperbolic functions may be expressed simply in<br />
terms of the exponential function:<br />
sinh α = eα − e−α cosh α =<br />
2<br />
eα + e−α 2<br />
tanh α =<br />
sech α =<br />
sinh α<br />
cosh α = eα − e−α eα + e−α 1<br />
cosh α =<br />
2<br />
e α + e −α<br />
cotanh α =<br />
cosech α =<br />
cosh α<br />
sinh α = eα + e−α eα − e−α 1<br />
sinh α =<br />
2<br />
e α − e −α<br />
Note that the domains of sinh, cosh, tanh, and sech are (−∞, ∞) and the domains of<br />
cotanh and cosech are (−∞, 0) ∪ (0, ∞).<br />
1<br />
x<br />
University of<br />
DEO PAT-<br />
ET RIE<br />
Saskatchewan
α −α e + e<br />
We can check that the point<br />
,<br />
2<br />
eα − e−α <br />
lies on the unit hyperbola:<br />
2<br />
α −α 2 α −α 2 e + e e − e<br />
−<br />
=<br />
2<br />
2<br />
e2α + 2 + e−2α −<br />
4<br />
e2α − 2 + e−2α =<br />
4<br />
4<br />
= 1<br />
4<br />
“Pythagorean” Identities<br />
This gives us the first important hyperbolic function identity:<br />
cosh 2 α − sinh 2 α ≡ 1<br />
This may be used to derive two other identities relating the two other pairs of hyperbolic<br />
functions:<br />
1 − tanh 2 α = sech 2 α and cotanh 2 α − 1 = cosech 2 α<br />
Odd and Even Identities<br />
It is clear that sinh, tanh, cotanh xand cosech are odd functions, while cosh, cotanh ,<br />
and sech are even, so we have the corresponding identities:<br />
sinh(−x) =−sinh x, tanh(−x) =−tanh x,<br />
cotanh (−x) =−cotanh x, cosech (−x) =−cosech x<br />
cosh(−x) = cosh x, sech (−x) = sech x.<br />
Sum and Difference Identities<br />
We can use the above formulas for the hyperbolic functions in terms of e x to derive<br />
analogs of the identities for the trigonometric functions:<br />
sinh α cosh β = eα − e −α<br />
e α+β + e α−β − e −α+β − e −α−β<br />
4<br />
2<br />
sinh β cosh α = eβ − e −β<br />
2<br />
e β + e −β<br />
2<br />
e α + e −α<br />
2<br />
= (eα − e −α )(e β + e −β )<br />
4<br />
= (eβ − e −β )(e α + e −α )<br />
4<br />
2<br />
=<br />
=
e β+α + e β−α − e −β+α − e −β−α<br />
4<br />
Adding these two products gives:<br />
sinh α cosh β + sinh β cosh α =<br />
e α+β + e α−β − e −α+β − e −α−β<br />
4<br />
2e α+β − 2e −α−β<br />
4<br />
= eα+β − e −α−β<br />
2<br />
+ eβ+α + e β−α + e −β+α − e −β−α<br />
4<br />
= e(α+β) − e −(α+β)<br />
2<br />
and subtracting these two products gives:<br />
sinh α cosh β − sinh β cosh α =<br />
e α+β + e α−β − e −α+β − e −α−β<br />
4<br />
2e α−β − 2e −(α−β)<br />
4<br />
Similarly,<br />
cosh α cosh β = eα + e −α<br />
= eα−β − e −(α−β)<br />
2<br />
e α+β + e α−β + e β−α + e −α−β<br />
4<br />
2<br />
sinh α sinh β = eα − e −α<br />
e α+β − e α−β − e β−α + e −α−β<br />
4<br />
2<br />
=<br />
= sinh(α + β)<br />
− eβ+α + e β−α + e −β+α − e −β−α<br />
4<br />
e β + e −β<br />
2<br />
e β − e −β<br />
Adding these two products gives<br />
cosh α cosh β + sinh α sinh β =<br />
e α+β + e α−β + e β−α + e −α−β<br />
4<br />
2<br />
= sinh(α − β)<br />
= (eα + e −α )(e β + e −β )<br />
4<br />
= (eα − e −α )(e β − e −β )<br />
4<br />
+ eα+β − e α−β − e β−α + e −α−β<br />
4<br />
3<br />
=<br />
=<br />
=<br />
=
2e α+β + 2e −α−β<br />
4<br />
= eα+β + e −(α+β)<br />
2<br />
and subtracting them gives:<br />
cosh α cosh β − sinh α sinh β =<br />
e α+β + e α−β + e β−α + e −α−β<br />
4<br />
2e α−β + 2e −α+β<br />
4<br />
= eα−β + e −(α−β)<br />
2<br />
Summarizing, we have four identities:<br />
= cosh(α + β)<br />
− eα+β − e α−β − e β−α + e −α−β<br />
4<br />
= cosh(α − β)<br />
sinh(α + β) ≡ sinh α cosh β + sinh β cosh α<br />
sinh(α − β) ≡ sinh α cosh β − sinh β cosh α<br />
cosh(α + β) ≡ cosh α cosh β + sinh α sinh β<br />
cosh(α − β) ≡ cosh α cosh β − sinh α sinh β<br />
which are almost exactly parallel to those for the trigonometric functions and may be<br />
used to derive sum and difference formulas for the other four hyperbolic functions.<br />
Letting β = α, we get:<br />
sinh 2α ≡ 2 sinh α cosh α,<br />
Double and Half-“Angle” Identities<br />
cosh 2α ≡ cosh 2 α + sinh 2 α ≡ 1 + 2 sinh 2 α ≡ 2 cosh 2 α − 1, so<br />
cosh 2 cosh 2α + 1<br />
α = and sinh<br />
2<br />
2 cosh 2α − 1<br />
α = , and thus:<br />
2<br />
<br />
<br />
cosh α =<br />
cosh 2α + 1<br />
and sinh α =<br />
2<br />
cosh 2α − 1<br />
2<br />
4<br />
=
cosh α<br />
2 =<br />
<br />
cosh α + 1<br />
2<br />
d<br />
d<br />
(sinh x) =<br />
dx dx<br />
d<br />
d<br />
(cosh x) =<br />
dx dx<br />
and sinh α<br />
2 =<br />
<br />
cosh α − 1<br />
2<br />
Derivatives<br />
x −x <br />
e − e<br />
2<br />
x −x <br />
e + e<br />
<br />
d<br />
d sinh x<br />
(tanh x) = =<br />
dx dx cosh x<br />
cosh x(sinh x) ′ − sinh x(cosh x) ′<br />
cosh 2 x<br />
cosh 2 x − sinh 2 x<br />
cosh 2 x<br />
=<br />
d<br />
d<br />
(cotanh x) =<br />
dx dx<br />
2<br />
= ex − (−e −x )<br />
2<br />
= ex + (−e −x )<br />
2<br />
= cosh x cosh x − sinh x sinh x<br />
1<br />
cosh 2 x = sech 2 x<br />
<br />
cosh x<br />
=<br />
sinh x<br />
sinh x(cosh x) ′ − cosh x(sinh x) ′<br />
sinh 2 x<br />
sinh 2 x − cosh 2 x<br />
sinh 2 x<br />
= sinh x sinh x − cosh x cosh x<br />
= −1<br />
sinh 2 x =−cosech 2 x<br />
d<br />
d<br />
(sech x) =<br />
dx dx (cosh x)−1 =<br />
= ex + e −x<br />
2<br />
= ex − e −x<br />
2<br />
= cosh x<br />
= sinh x<br />
cosh 2 x<br />
sinh 2 x<br />
(−1) (cosh x) −2 (cosh x) ′ = (−1) (cosh x) −2 sinh x =−sech x tanh x<br />
d<br />
d<br />
(cosech x) =<br />
dx dx (sinh x)−1 =<br />
5<br />
=<br />
=
(−1) (sinh x) −2 (sinh x) ′ = (−1) (sinh x) −2 cosh x =−cosech xcotanh x<br />
d<br />
(sinh x) = cosh x<br />
dx<br />
d<br />
dx (tanh x) = sech 2 x<br />
d<br />
(sech x) =−sech x tanh x<br />
dx<br />
Summary:<br />
d<br />
(cosh x) = sinh x<br />
dx<br />
d<br />
dx (cotanh x) =−cosech 2 x<br />
d<br />
(cosech x) =−cosech xcotanh x<br />
dx<br />
Graphs ofthe <strong>Hyperbolic</strong> <strong>Functions</strong><br />
y<br />
y = sinh x<br />
y = cosech x<br />
x<br />
y = cosh x<br />
y = sech x<br />
The domains and ranges are summarized in the next table:<br />
6<br />
y<br />
x<br />
y<br />
y = tanh x<br />
y = cotanh x<br />
x
function domain Range<br />
sinh (−∞, ∞) (−∞, ∞)<br />
cosh (−∞, ∞) [1, ∞)<br />
tanh (−∞, ∞) (−1, 1)<br />
cotanh (−∞, 0) ∪ (0, ∞) (−∞, −1) ∪ (1, ∞)<br />
sech (−∞, ∞) (0, 1])<br />
cosech (−∞, 0) ∪ (0, ∞) (−∞, 0) ∪ (0, ∞)<br />
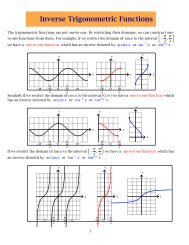
Inverse <strong>Hyperbolic</strong> <strong>Functions</strong><br />
sinh, tanh, cotanh and cosech are one-to-one, but cosh and sech are not. For the<br />
purpose of defining the inverse of cosh and sech we will restrict their domains to<br />
[0, ∞).<br />
We will denote the inverse hyperbolic functions by<br />
sinh −1 , cosh −1 , tanh −1 , cotanh −1 , sech −1 , and cosech −1<br />
or:<br />
sinh inv , cosh inv , tanh inv , cotanh inv , sech inv , and cosech inv<br />
or even:<br />
arcsinh , arccosch , arctanh , arccothh , arcsech , and arccosech .<br />
The usual Cancellation Laws hold in the appropriate domains:<br />
sinh(sinh −1 x) ≡ x sinh −1 (sinh x) ≡ x<br />
cosh(cosh −1 x) ≡ x cosh −1 (cosh x) ≡ x<br />
tanh(tanh −1 x) ≡ x tanh −1 (tanh x) ≡ x<br />
cotanh (cotanh −1 x) ≡ x cotanh −1 (cotanh x) ≡ x<br />
sech (sech −1 x) ≡ x sech −1 (sech x) ≡ x<br />
cosech (cosech −1 x) ≡ x cosech −1 (cosech x) ≡ x<br />
The derivatives of the inverse hyperbolic functions may be found the same way the<br />
derivatives of the inverse trigonometric functions were found: by differentiating the<br />
left-hand Cancellation Laws above:<br />
Example:<br />
Differentiating sinh(sinh −1 x) ≡ x we get<br />
7
cosh(sinh −1 <br />
x) sinh −1 ′<br />
x = 1, so<br />
<br />
sinh −1 ′ 1<br />
x =<br />
cosh(sinh −1 x) .<br />
Using the identity cosh 2 x − sinh 2 x ≡ 1weget<br />
cosh 2 x ≡ 1 + sinh 2 x,so<br />
<br />
cosh x ≡ 1 + sinh 2 x and therefore<br />
cosh(sinh −1 x) =<br />
=<br />
<br />
1 + x 2<br />
Thus we have<br />
<br />
1 + sinh 2 (sinh −1 <br />
<br />
x) = 1 + sinh(sinh −1 2 x)<br />
<br />
sinh −1 ′<br />
x =<br />
1<br />
√ 1 + x 2<br />
One may similarly derive the derivatives of the other hyperbolic functions:<br />
<br />
cosh −1 ′<br />
x =<br />
1<br />
√ x − 1 2<br />
<br />
tanh −1 ′ <br />
x = cotanh −1 ′<br />
x =<br />
<br />
sech −1 ′<br />
x<br />
<br />
cosech −1 ′<br />
x =<br />
1<br />
=−<br />
x √ 1 − x2 −1<br />
|x| √ 1 + x 2<br />
1<br />
1 − x 2<br />
Explicit Computation ofInverse <strong>Hyperbolic</strong>s<br />
The inverse hyperbolic functions have the unusual property that they can be explicity<br />
computed:<br />
Example: Solve the equation sinh y = x for y in terms of x.<br />
(The solution will be sinh −1 x!)<br />
We have sinh y = ey − e−y = x, so<br />
2<br />
ey −e−y = 2x or ey −2x −e−y = 0. Multiplying both sides of this equation by e y we get:<br />
8
(e y ) 2 − 2xe y − 1 = 0, a quadratic equation in e y which has solution<br />
e y = −(−2x) ± (−2x) 2 − 4(1)(−1)<br />
2<br />
= 2x ± √ 4x 2 + 4<br />
2<br />
Since x − √ x2 + 1 < 0 and we must have ey > 0, we get<br />
e y <br />
= x + x2 + 1.<br />
Taking logarithms of both sides of this equation, we get<br />
y = ln(x + √ x2 + 1), so we have<br />
sinh −1 <br />
x = ln(x + x2 + 1)<br />
<br />
= x ± x2 + 1<br />
Similarly,<br />
cosh −1 <br />
x = ln(x + x2 − 1) and tanh −1 x = 1<br />
2 ln<br />
<br />
1 + x<br />
1 − x<br />
We then have<br />
cosech −1 x = sinh −1 1<br />
x<br />
ln<br />
1<br />
x +<br />
√ <br />
1 + x2 |x|<br />
= ln<br />
Similarly<br />
cotanh −1 x = 1<br />
2 ln<br />
<br />
x + 1<br />
x − 1<br />
⎛<br />
⎝ 1<br />
x +<br />
<br />
2 1<br />
x<br />
⎞ ⎛<br />
+ 1⎠<br />
= ln ⎝ 1<br />
x +<br />
<br />
1 + x2 x2 ⎞<br />
⎠ =<br />
and sech −1 √ <br />
1 + 1 − x2 x = ln<br />
x<br />
9