On 1/18

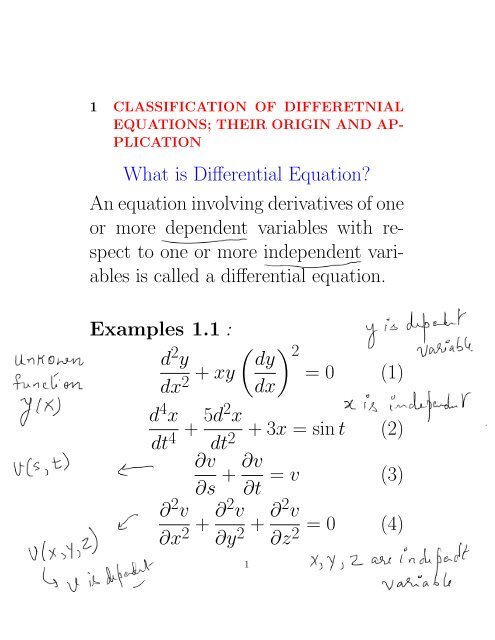
1 CLASSIFICATION OF DIFFERETNIAL
EQUATIONS; THEIR ORIGIN AND AP-
PLICATION
What is Differential Equation?
An equation involving derivatives of one
or more dependent variables with respect
to one or more independent variables
is called a differential equation.
Examples 1.1 :
d2 2 y dy
+ xy = 0
dx2 dx
(1)
d4x dt4 + 5d2x + 3x = sin t
dt2 ∂v ∂v
+ = v
∂s ∂t
∂
(2)
(3)
2v ∂x2 + ∂2v ∂y2 + ∂2v = 0
∂z2 (4)
1

What are the independent variable and
dependent variable in equation 1, 2, 3,
4?
DEFINITION:
A differential equation involving ordinary
derivatives of one or more dependent
variables with respect to a single
independent variable is called an ordinary
differential equation, O.D.E.
2

So which of the above equation in Example
1.1 are O.D.E?
DEFINITION:
A differential equation involving partial
derivatives of one or more dependent
variables with respect to more than
one independent variable is called a partial
differential equation, P.D.E.
So, which equations from Example 1.1
are P.D.E?
3

EMCF01
(1) Classify the independent and dependent
variables in the follwing differential
equation ∂4 u
∂x 3 ∂y
+ 5x∂u
∂x
+ u = 0 :
(a) u is independent function and x and
y are dependent variable
(b) x and y are independent variable and
u is a dependent function
(c) u, x and y are independent functions.
(e) None of the above.
4

What is the order of differential equation?
order of differential equation is the order
of the highest ordered derivatives involved
in its expression.
Examples 1.2
Order is
Order is
Order is
d2y + xy
dx2
dy
dx
d2 2 y dy
+ x
dx2 dx
= 0 (5)
= 0 (6)
d4x dt4 + d2x + x = 0 (7)
dt2 5

What is the degree of differential equation?
The degree of differential equation is the
degree that its highest ordered derivative
would have if the equation were rationalized
and cleared of fraction with
regard to all derivatives involved in it.
Examples 1.3
d2y + xy
dx2 Degree is
Degree is
dy
dx
+ x
dy
dx
2 dy
dx
= 0 (8)
= 0 (9)
(y ′ ) 2 + 1
(y ′ = 0 (10)
) 2
6

EMCF01
(2) Classify the following differential equation
d3y + 5xdy + y sin x = 0 :
dx3 dx
(a) A third order linear ordinary differential
equation
(b) A second order linear partial differential
equation
(c) A second order linear ordinary differential
equation
(d) A third order linear partial differential
equation
(e) None of the above.
7

What do we mean by solution of differential
equation?
A function y = g(x) which solves the
differential equation,
F (x, y(x), y ′ (x), y ′′ (x)....) = 0. Here,
when we plug y (i.e g(x)) and its derivatives
w.r.t x in the differential equation
then equation gets identically satisfies.
Examples 1.4
y = g(x) = 2 sin x + 3 cos x (11)
is the explicit solution of y ′′ + y = 0.
x 2 + y 2 − 25 = 0 (12)
is the implicit solution of x+yy ′ = 0.
8

EMCF01
(3) Which of the following function solves
(a) y(x) = x 2
(b) y 2 + x 2 = 16
(c) y(x) = x
(d) y(x) + x 2 = 2
yy ′ + x = 0
(e) None of the above.
9

Examples 1.5 Given
Show that y(x) solves
y(x) = x 2 + x (13)
y ′′ + y ′ − 2y = 3 − 2x 2
10
(14)

Examples 1.6 Whether
log y + x
= c (15)
y
solves
(y − x)y ′ + y = 0? (16)
11

Examples 1.7 Show that every function
f defined by
f(x) = (x 3 + c)e −3x
(17)
Where c is arbitrary constant, is a
solution of the differential equation
dy
dx + 3y = 3x2e −3x
(18)
12

Examples 1.8 Show that every function
f defined by
f(x) = ce 3x
(19)
Where c is arbitrary constant, is a
solution of the differential equation
y ′ = 3y (20)
13

EMCF01
(4) How many solutions are possible for
the following differential equation
(a) Only one
(b) No solution
y ′ = y
(c) Infinitely many solutions
(e) None of the above.
14

Moral from example 1.7 and 1.8 is .....
Differential equation has infinitely many
solution we need to give some extra information
in order to get the specific
(unique solution) and Thus, we have following
questions
1) What is the information which we
need to get unique solution?
2) Is there any connection of that information
with the order of differential
equation?
15

Let us view example 1.8 geometrically
and guess what do we need to get unique
solution?
16

Initial Value Problem
F (x, y(x), y ′ (x), y ′′ (x)....y (n) (a)) = 0
y(a) = b
y ′ (a) = b
.
.
.
y (n−1) (a) = b
17

EMCF01
(5) Which of the following is an Initial
valued problem?
(a) y ′′ + 2y = 0; y(0) = 0, y(1) = 0
(b) y ′′ + 2y ′ = 0; y(0) = 0
(c) y ′′ + 2y ′ = 2; y(0) = 0, y ′ (0) = 0
(d) None of the above.
18

Examples 1.9 Given that every function
f defined by
f(x) = c1e 4x + c2e −3x
(21)
is a solution of the differential equation
d2y dy
− − 12y = 0 (22)
dx2 dx
for some arbitrary choice of c1, c2.
Solve I.V.P
d2y dy
−
dx2 dx
− 12y = 0
y(0) = 5, y ′ (0) = 6
19

Seperable Differential Equation
y ′ = f(x)g(y)
is said to be seperable differential equation.
Examples 1.10
(x − 4)y 4 dx − x 3 (y 2 − 3)dy = 0 (23)
Examples 1.11
4xydx + (x 2 + 1)dy = 0 (24)
20

Solution method
dy
= f(x)g(y)
dx
Step 1: Seperate x’s and y’s on different
sides.
dy
= f(x)dx
g(y)
Step 2: Integrate both the sides.
dy
g(y) =
f(x)dx + C
Step 3: Express y in terms of x where
possible.
Step 4: Check that constant solutions
y = C, where g(C) = 0 are not missed.
22