Survey 1979: Equational Logic - Department of Mathematics ...

WALTER TAYLOR 33
(All theories intended as extensions of 23 = {x(yz) = (xy)z} .)
13.5. Forcommutativesemigroups, see [349], [179], [325],[388],and [78].
Perkins proved that every commutative semigroup theory is finitely based (cf. 9.3
above), and hence this lattice is countable. And so it cannot contain IIoo, but it does
contain every IIm (Burris-Nelson [78]) and hence obeys no special lattice laws;
Schwabauer had earlier proved [388] that the lattice of commutative semigroup
theories is nonmodular. For semigroups with zero, consult [324], [83], and with unit
[179]. For related work see [344], [350], [351].
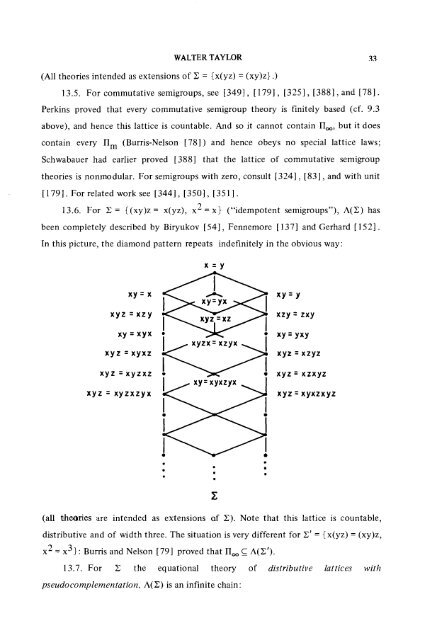
13.6. For 23 = ((xy)z = x(yz), x 2= x} ("idempotent semigroups"), A(23) has
been completely described by Biryukov [54], Fennemore [137] and Gerhard [152].
In this picture, the diamond pattern repeats indefinitely in the obvious way:
xy=x
xyz = xz y
xy = xyx
xyz = xyxz
xyz = xyzxz
xyz = xyzxzyx
xy=y
xzy = zxy
xy = yxy
xyz = xzyz
xyz = xzxyz
xyz = xyxzxyz
(all [hexries are intended as extensions of 23). Note that this lattice is countable,
distributive and of width three. The situation is very different for 23' = (x(yz) = (xy)z,
x 2 = x3} ß Burris and Nelson [79] proved that Iloo C_
13.7. For 23 the equational theory of distributive lattices with
pseudocomplementation, A(23) is an infinite chain: