Nonlinear Mechanics - Physics at Oregon State University
Nonlinear Mechanics - Physics at Oregon State University
Nonlinear Mechanics - Physics at Oregon State University
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
3.1. NOTATION 35<br />
Those of you who have studied special rel<strong>at</strong>ivity should find (??) congenial.<br />
Remember the definition of a Lorentz transform<strong>at</strong>ion: any 4×4 m<strong>at</strong>rix<br />
Λ th<strong>at</strong> s<strong>at</strong>isfies<br />
g = Λ · g · Λ T<br />
(3.11)<br />
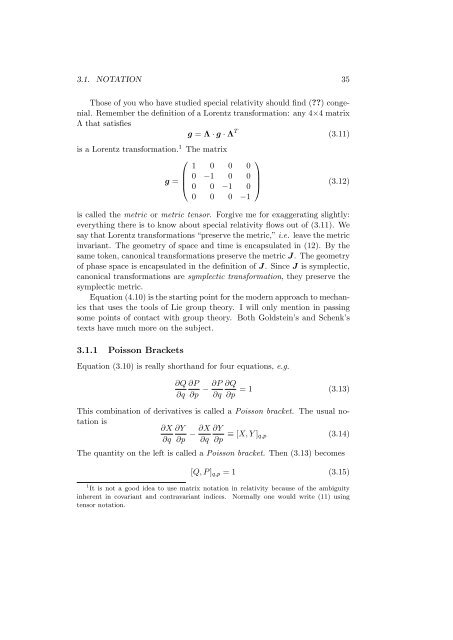
is a Lorentz transform<strong>at</strong>ion. 1 The m<strong>at</strong>rix<br />
⎛<br />
1 0 0<br />
⎞<br />
0<br />
⎜<br />
g = ⎜ 0<br />
⎝ 0<br />
−1<br />
0<br />
0<br />
−1<br />
0 ⎟<br />
0 ⎠<br />
0 0 0 −1<br />
(3.12)<br />
is called the metric or metric tensor. Forgive me for exagger<strong>at</strong>ing slightly:<br />
everything there is to know about special rel<strong>at</strong>ivity flows out of (3.11). We<br />
say th<strong>at</strong> Lorentz transform<strong>at</strong>ions “preserve the metric,” i.e. leave the metric<br />
invariant. The geometry of space and time is encapsul<strong>at</strong>ed in (12). By the<br />
same token, canonical transform<strong>at</strong>ions preserve the metric J. The geometry<br />
of phase space is encapsul<strong>at</strong>ed in the definition of J. Since J is symplectic,<br />
canonical transform<strong>at</strong>ions are symplectic transform<strong>at</strong>ion, they preserve the<br />
symplectic metric.<br />
Equ<strong>at</strong>ion (4.10) is the starting point for the modern approach to mechanics<br />
th<strong>at</strong> uses the tools of Lie group theory. I will only mention in passing<br />
some points of contact with group theory. Both Goldstein’s and Schenk’s<br />
texts have much more on the subject.<br />
3.1.1 Poisson Brackets<br />
Equ<strong>at</strong>ion (3.10) is really shorthand for four equ<strong>at</strong>ions, e.g.<br />
∂Q ∂P<br />
∂q ∂p<br />
∂P ∂Q<br />
− = 1 (3.13)<br />
∂q ∂p<br />
This combin<strong>at</strong>ion of deriv<strong>at</strong>ives is called a Poisson bracket. The usual not<strong>at</strong>ion<br />
is<br />
∂X ∂Y ∂X ∂Y<br />
− ≡ [X, Y ]q,p<br />
∂q ∂p ∂q ∂p<br />
(3.14)<br />
The quantity on the left is called a Poisson bracket. Then (3.13) becomes<br />
[Q, P ]q,p = 1 (3.15)<br />
1 It is not a good idea to use m<strong>at</strong>rix not<strong>at</strong>ion in rel<strong>at</strong>ivity because of the ambiguity<br />
inherent in covariant and contravariant indices. Normally one would write (11) using<br />
tensor not<strong>at</strong>ion.