1. (a) - Physics at Oregon State University

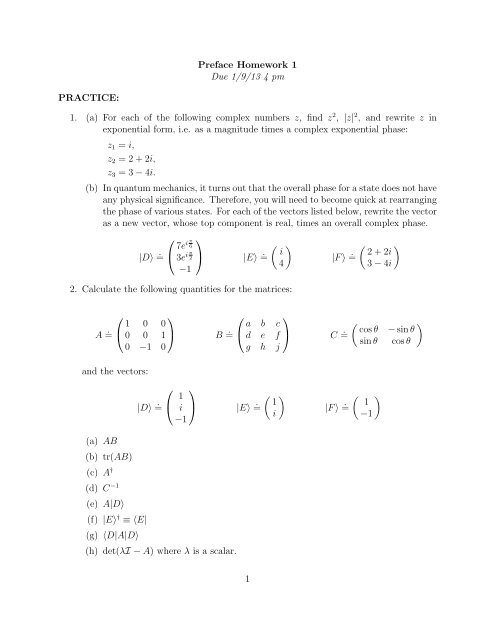
PRACTICE:
Preface Homework 1
Due 1/9/13 4 pm
1. (a) For each of the following complex numbers z, find z 2 , |z| 2 , and rewrite z in
exponential form, i.e. as a magnitude times a complex exponential phase:
z1 = i,
z2 = 2 + 2i,
z3 = 3 − 4i.
(b) In quantum mechanics, it turns out that the overall phase for a state does not have
any physical significance. Therefore, you will need to become quick at rearranging
the phase of various states. For each of the vectors listed below, rewrite the vector
as a new vector, whose top component is real, times an overall complex phase.
⎛
7ei π
6
⎞
|D〉 . π
= ⎝ i 3e 2 ⎠ |E〉
−1
. =
i
4
2. Calculate the following quantities for the matrices:
|F 〉 . =
A . ⎛
1
= ⎝ 0
0
0
⎞
0
1 ⎠ B
0 −1 0
. ⎛
a
= ⎝ d
b
e
⎞
c
f ⎠ C
g h j
. =
and the vectors:
(a) AB
(b) tr(AB)
(c) A †
(d) C −1
(e) A|D〉
(f) |E〉 † ≡ 〈E|
(g) 〈D|A|D〉
|D〉 . =
⎛
⎝ 1
i
−1
⎞
⎠ |E〉 . =
(h) det(λI − A) where λ is a scalar.
1
1
i
|F 〉 . =
2 + 2i
3 − 4i
cos θ
− sin θ
sin θ cos θ
1
−1

(i) (A|D〉) †
(j) Using explicit matrix multiplication (without using a theorem) verify that (A|D〉) † =
〈D|A †
3. The Pauli spin matrices σx, σy, and σz are defined by:
σx =
0 1
1 0
σy =
0 −i
i 0
σz =
1 0
0 −1
These matrices are related to angular momentum in quantum mechanics. Prove, and
become familiar with, the identities listed below.
(a) Show that each of the Pauli matrices is hermitian. (A matrix is hermitian if it is
equal to its hermitian adjoint.
(b) Show that the determinant of each of the Pauli matrices is −1.
(c) Show that σ 2 i = I for each of the Pauli matrices, i.e. for i ∈ {x, y, z}.
4. Perform the following matrix multiplications:
REQUIRED:
1 1 1 3 1
√
2 1 −1 1 3
5. The Pauli spin matrices σx, σy, and σz are defined by:
σx =
0 1
1 0
σy =
0 −i
i 0
1 1 1
√
2 1 −1
σz =
1 0
0 −1
These matrices are related to angular momentum in quantum mechanics. Prove, and
become familiar with, the identities listed below.
(a) Show that σxσy = iσz and σyσx = −iσz. (Note: These identities also hold under
a cyclic permutation of {x, y, z}, e.g. x → y, y → z, and z → x).
(b) The commutator of two matrices A and B is defined by [A, B] def
= AB −BA. Show
that [σx, σy] = 2iσz. (Note: This identity also holds under a cyclic permutation
of {x, y, z}, e.g. x → y, y → z, and z → x).
(c) The anti-commutator of two matrices A and B is defined by {A, B} def
= AB +
BA. Show that {σx, σy} = 0. (Note: This identity also holds under a cyclic
permutation of {x, y, z}, e.g. x → y, y → z, and z → x).
2

6. Consider the following matrices:
A =
0 1
1 0
B =
3 1
1 3
C =
1 0
0 −1
(a) Explain what each of the matrices “does” geometrically when thought of as a
linear transformation acting on a vector.
(b) The commutator of two matrices A and B is defined by [A, B] def
= AB − BA. Find
the following commutators: [A, B], [A, C], [B, C].
(c) Two matrices are said to “commute,” if their commutator is zero. Thought of as
linear transformations, two matrices commute if it doesn’t matter in which order
the transformations act. For all pairs of the matrices A, B, and C, show geometrically
that the order of the transformations doesn’t matter when the matrices
commute and does matter when they don’t commute.
7. (a) Carry out the following matrix calculations.
and
( 0 1 0 )
( 0 1 0 )
⎛
⎝ a11 a12 a13
a21 a22 a23
⎛
a31 a32 a33
⎝ a11 a12 a13
a21 a22 a23
a31 a32 a33
⎞ ⎛
⎠ ⎝ 1
⎞
0 ⎠
0
⎞ ⎛
⎠ ⎝ 0
⎞
1 ⎠
0
(b) What matrix multiplication would you do if you wanted the answer to be a13?
(c) The bra-ket language for the calulations in part (a) are
〈2|A|1〉 =? and 〈2|A|2〉 =?
Write the question to part (b) in bra-ket language.
8. Consider the matrix:
A . =
0 −i
i 0
Find the matrix M . = e iAθ where θ is an arbitrary real scalar parameter. To do this
problem, expand the exponential in a power series, without worrying too much about
what A is. Then, you will need to compute all of the powers of A. It’s easier than it
looks!
3