Compatibility of induction methods for mantle ... - MTNet - DIAS

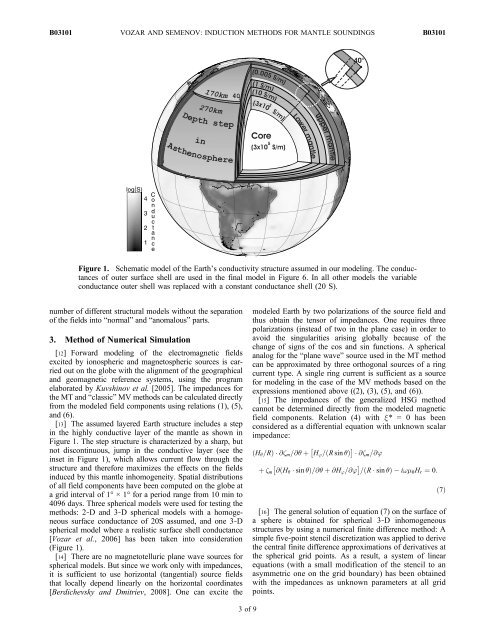
B03101
number of different structural models without the separation
of the fields into “normal” and “anomalous” parts.
3. Method of Numerical Simulation
VOZAR AND SEMENOV: INDUCTION METHODS FOR MANTLE SOUNDINGS B03101
Figure 1. Schematic model of the Earth’s conductivity structure assumed in our modeling. The conductances
of outer surface shell are used in the final model in Figure 6. In all other models the variable
conductance outer shell was replaced with a constant conductance shell (20 S).
[12] Forward modeling of the electromagnetic fields
excited by ionospheric and magnetospheric sources is carried
out on the globe with the alignment of the geographical
and geomagnetic reference systems, using the program
elaborated by Kuvshinov et al. [2005]. The impedances for
the MT and “classic” MV methods can be calculated directly
from the modeled field components using relations (1), (5),
and (6).
[13] The assumed layered Earth structure includes a step
in the highly conductive layer of the mantle as shown in
Figure 1. The step structure is characterized by a sharp, but
not discontinuous, jump in the conductive layer (see the
inset in Figure 1), which allows current flow through the
structure and therefore maximizes the effects on the fields
induced by this mantle inhomogeneity. Spatial distributions
of all field components have been computed on the globe at
a grid interval of 1° × 1° for a period range from 10 min to
4096 days. Three spherical models were used for testing the
methods: 2‐D and 3‐D spherical models with a homogeneous
surface conductance of 20S assumed, and one 3‐D
spherical model where a realistic surface shell conductance
[Vozar et al., 2006] has been taken into consideration
(Figure 1).
[14] There are no magnetotelluric plane wave sources for
spherical models. But since we work only with impedances,
it is sufficient to use horizontal (tangential) source fields
that locally depend linearly on the horizontal coordinates
[Berdichevsky and Dmitriev, 2008]. One can excite the
3of9
modeled Earth by two polarizations of the source field and
thus obtain the tensor of impedances. One requires three
polarizations (instead of two in the plane case) in order to
avoid the singularities arising globally because of the
change of signs of the cos and sin functions. A spherical
analog for the “plane wave” source used in the MT method
can be approximated by three orthogonal sources of a ring
current type. A single ring current is sufficient as a source
for modeling in the case of the MV methods based on the
expressions mentioned above ((2), (3), (5), and (6)).
[15] The impedances of the generalized HSG method
cannot be determined directly from the modeled magnetic
field components. Relation (4) with x* = 0 has been
considered as a differential equation with unknown scalar
impedance:
ðH =RÞ
@ m=@ þ H’= ðRsin Þ @ m=@’
þ m @ðHsin Þ=@ þ @H’=@’ = ðRsin Þ i! 0Hr ¼ 0:
[16] The general solution of equation (7) on the surface of
a sphere is obtained for spherical 3‐D inhomogeneous
structures by using a numerical finite difference method: A
simple five‐point stencil discretization was applied to derive
the central finite difference approximations of derivatives at
the spherical grid points. As a result, a system of linear
equations (with a small modification of the stencil to an
asymmetric one on the grid boundary) has been obtained
with the impedances as unknown parameters at all grid
points.
ð7Þ