P. Schmoldt, PhD - MTNet - DIAS

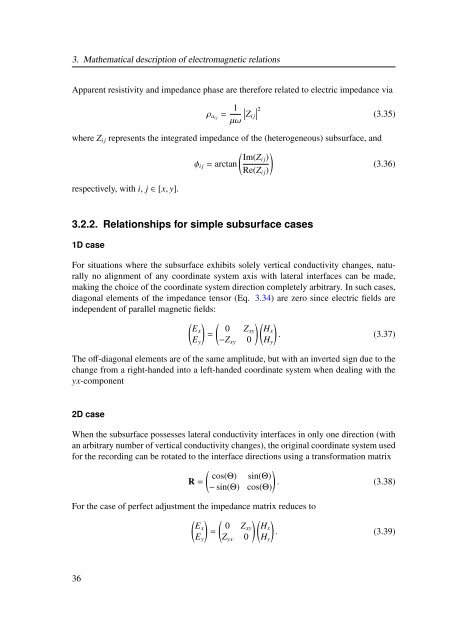
3. Mathematical description of electromagnetic relations
Apparent resistivity and impedance phase are therefore related to electric impedance via
ρai j
1
=
µω
Zi j
2
where Zi j represents the integrated impedance of the (heterogeneous) subsurface, and
respectively, with i, j ∈ [x, y].
φi j = arctan
Im(Zi j)
Re(Zi j)
3.2.2. Relationships for simple subsurface cases
1D case
(3.35)
(3.36)
For situations where the subsurface exhibits solely vertical conductivity changes, naturally
no alignment of any coordinate system axis with lateral interfaces can be made,
making the choice of the coordinate system direction completely arbitrary. In such cases,
diagonal elements of the impedance tensor (Eq. 3.34) are zero since electric fields are
independent of parallel magnetic fields:
Ex
Ey
=
0 Zxy
−Zxy 0
Hx
Hy
. (3.37)
The off-diagonal elements are of the same amplitude, but with an inverted sign due to the
change from a right-handed into a left-handed coordinate system when dealing with the
yx-component
2D case
When the subsurface possesses lateral conductivity interfaces in only one direction (with
an arbitrary number of vertical conductivity changes), the original coordinate system used
for the recording can be rotated to the interface directions using a transformation matrix
cos(Θ) sin(Θ)
R =
. (3.38)
− sin(Θ) cos(Θ)
For the case of perfect adjustment the impedance matrix reduces to
Ex 0
=
Zyx
Zxy Hx
.
0
(3.39)
36
Ey
Hy