WAVES AND VIBRATIONS IN INHOMOGENEOUS STRUCTURES ...

Topological material layout in plates for vibration suppression and wave propagation control
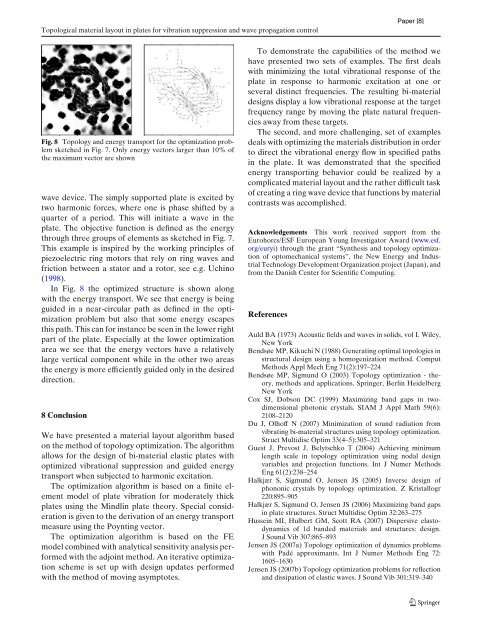
Fig. 8 Topology and energy transport for the optimization problem
sketched in Fig. 7. Only energy vectors larger than 10% of
the maximum vector are shown
wave device. The simply supported plate is excited by
two harmonic forces, where one is phase shifted by a
quarter of a period. This will initiate a wave in the
plate. The objective function is defined as the energy
through three groups of elements as sketched in Fig. 7.
This example is inspired by the working principles of
piezoelectric ring motors that rely on ring waves and
friction between a stator and a rotor, see e.g. Uchino
(1998).
In Fig. 8 the optimized structure is shown along
with the energy transport. We see that energy is being
guided in a near-circular path as defined in the optimization
problem but also that some energy escapes
this path. This can for instance be seen in the lower right
part of the plate. Especially at the lower optimization
area we see that the energy vectors have a relatively
large vertical component while in the other two areas
the energy is more efficiently guided only in the desired
direction.
8 Conclusion
We have presented a material layout algorithm based
on the method of topology optimization. The algorithm
allows for the design of bi-material elastic plates with
optimized vibrational suppression and guided energy
transport when subjected to harmonic excitation.
The optimization algorithm is based on a finite element
model of plate vibration for moderately thick
plates using the Mindlin plate theory. Special consideration
is given to the derivation of an energy transport
measure using the Poynting vector.
The optimization algorithm is based on the FE
model combined with analytical sensitivity analysis performed
with the adjoint method. An iterative optimization
scheme is set up with design updates performed
with the method of moving asymptotes.
To demonstrate the capabilities of the method we
have presented two sets of examples. The first deals
with minimizing the total vibrational response of the
plate in response to harmonic excitation at one or
several distinct frequencies. The resulting bi-material
designs display a low vibrational response at the target
frequency range by moving the plate natural frequencies
away from these targets.
The second, and more challenging, set of examples
deals with optimizing the materials distribution in order
to direct the vibrational energy flow in specified paths
in the plate. It was demonstrated that the specified
energy transporting behavior could be realized by a
complicated material layout and the rather difficult task
of creating a ring wave device that functions by material
contrasts was accomplished.
Acknowledgements This work received support from the
Eurohorcs/ESF European Young Investigator Award (www.esf.
org/euryi) through the grant “Synthesis and topology optimization
of optomechanical systems”, the New Energy and Industrial
Technology Development Organization project (Japan), and
from the Danish Center for Scientific Computing.
References
Auld BA (1973) Acoustic fields and waves in solids, vol I. Wiley,
New York
Bendsøe MP, Kikuchi N (1988) Generating optimal topologies in
structural design using a homogenization method. Comput
Methods Appl Mech Eng 71(2):197–224
Bendsøe MP, Sigmund O (2003) Topology optimization - theory,
methods and applications. Springer, Berlin Heidelberg
New York
Cox SJ, Dobson DC (1999) Maximizing band gaps in twodimensional
photonic crystals. SIAM J Appl Math 59(6):
2108–2120
Du J, Olhoff N (2007) Minimization of sound radiation from
vibrating bi-material structures using topology optimization.
Struct Multidisc Optim 33(4–5):305–321
Guest J, Prevost J, Belytschko T (2004) Achieving minimum
length scale in topology optimization using nodal design
variables and projection functions. Int J Numer Methods
Eng 61(2):238–254
Halkjær S, Sigmund O, Jensen JS (2005) Inverse design of
phononic crystals by topology optimization. Z Kristallogr
220:895–905
Halkjær S, Sigmund O, Jensen JS (2006) Maximizing band gaps
in plate structures. Struct Multidisc Optim 32:263–275
Hussein MI, Hulbert GM, Scott RA (2007) Dispersive elastodynamics
of 1d banded materials and structures: design.
J Sound Vib 307:865–893
Jensen JS (2007a) Topology optimization of dynamics problems
with Padé approximants. Int J Numer Methods Eng 72:
1605–1630
Jensen JS (2007b) Topology optimization problems for reflection
and dissipation of elastic waves. J Sound Vib 301:319–340