Investigating Oblique & Non-linear Asymptotes Activity
Investigating Oblique & Non-linear Asymptotes Activity
Investigating Oblique & Non-linear Asymptotes Activity
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
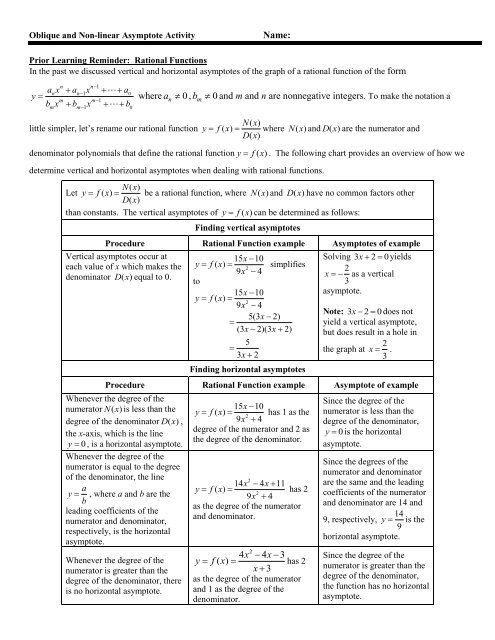
<strong>Oblique</strong> and <strong>Non</strong>-<strong>linear</strong> Asymptote <strong>Activity</strong> Name:<br />
Prior Learning Reminder: Rational Functions<br />
In the past we discussed vertical and horizontal asymptotes of the graph of a rational function of the form<br />
n n1<br />
n n1<br />
m m1<br />
m m1<br />
a x a x a0<br />
y <br />
b x b x b<br />
little simpler, let’s rename our rational function<br />
0<br />
where an 0 , b 0 and m and n are nonnegative integers. To make the notation a<br />
m<br />
Nx ( )<br />
y f ( x)<br />
where Nx ( ) and Dx ( ) are the numerator and<br />
Dx ( )<br />
denominator polynomials that define the rational function y f ( x)<br />
. The following chart provides an overview of how we<br />
determine vertical and horizontal asymptotes when dealing with rational functions.<br />
Nx ( )<br />
Let y f ( x)<br />
be a rational function, where Nx ( ) and Dx ( ) have no common factors other<br />
Dx ( )<br />
than constants. The vertical asymptotes of y f ( x)<br />
can be determined as follows:<br />
Finding vertical asymptotes<br />
Procedure Rational Function example <strong>Asymptotes</strong> of example<br />
Vertical asymptotes occur at<br />
each value of x which makes the<br />
denominator Dx ( ) equal to 0.<br />
15x 10<br />
y f ( x)<br />
2<br />
9x 4<br />
simplifies<br />
to<br />
15x 10<br />
y f ( x)<br />
2<br />
9x4 5(3x 2)<br />
<br />
(3x2)(3x2) 5<br />
<br />
3x2 Finding horizontal asymptotes<br />
Solving 3x 20yields 2<br />
x as a vertical<br />
3<br />
asymptote.<br />
Note: 3x 20does not<br />
yield a vertical asymptote,<br />
but does result in a hole in<br />
the graph at<br />
2<br />
x .<br />
3<br />
Procedure Rational Function example Asymptote of example<br />
Whenever the degree of the<br />
numerator Nx ( ) is less than the<br />
degree of the denominator Dx ( ) ,<br />
the x-axis, which is the line<br />
y 0 , is a horizontal asymptote.<br />
Whenever the degree of the<br />
numerator is equal to the degree<br />
of the denominator, the line<br />
a<br />
y , where a and b are the<br />
b<br />
leading coefficients of the<br />
numerator and denominator,<br />
respectively, is the horizontal<br />
asymptote.<br />
Whenever the degree of the<br />
numerator is greater than the<br />
degree of the denominator, there<br />
is no horizontal asymptote.<br />
15x 10<br />
y f ( x)<br />
has 1 as the<br />
2<br />
9x4 degree of the numerator and 2 as<br />
the degree of the denominator.<br />
y f ( x)<br />
<br />
2<br />
14x 4x11 2<br />
9x4 has 2<br />
as the degree of the numerator<br />
and denominator.<br />
2<br />
4x 4x3 y f ( x)<br />
<br />
has 2<br />
x 3<br />
as the degree of the numerator<br />
and 1 as the degree of the<br />
denominator.<br />
Since the degree of the<br />
numerator is less than the<br />
degree of the denominator,<br />
y 0 is the horizontal<br />
asymptote.<br />
Since the degrees of the<br />
numerator and denominator<br />
are the same and the leading<br />
coefficients of the numerator<br />
and denominator are 14 and<br />
14<br />
9, respectively, y is the<br />
9<br />
horizontal asymptote.<br />
Since the degree of the<br />
numerator is greater than the<br />
degree of the denominator,<br />
the function has no horizontal<br />
asymptote.
<strong>Investigating</strong> Other Types of <strong>Asymptotes</strong> of Rational Functions<br />
Connection to Prior Learning: Rational Numbers<br />
Given 141<br />
141<br />
r<br />
, use long division to find the quotient q and remainder r. Is equal to q , where d is the divisor? (Recall<br />
8 8 d<br />
that the divisor is the denominator. In this case, d = 8). Verify your answer.<br />
Extending to Rational Functions<br />
1. Let’s investigate a rational function where the degree of the numerator is one greater than the degree of the<br />
2<br />
4x 4x3 denominator. For example, we can examine the rational function y <br />
.<br />
x 3<br />
a) Use either long division or synthetic division in the space provided to determine the quotient and remainder. (Hint:<br />
The remainder should be 45. If you don’t get 45, raise your hand.)<br />
Quotient = Qx ()<br />
__________________<br />
Remainder = Rx ( ) 45<br />
Numerator & Denominator of Rational Function Nx ()<br />
________________<br />
Dx ()<br />
_________<br />
Nx ( )<br />
N( x) R( x)<br />
Rational functions of the form y f ( x)<br />
can be written as y f ( x) Q( x)<br />
<br />
Dx ( )<br />
D( x) D( x )<br />
where Qx ( ) and<br />
Rx ( ) are the quotient polynomial and the remainder polynomial, respectively, when we divide Nx ( ) by Dx ( ).<br />
Rewrite<br />
y <br />
2<br />
4x 4x3 x 3<br />
( )<br />
in the form ( ) .<br />
( )<br />
Rx<br />
y Q x<br />
Dx
2<br />
4x 4x3 b) Let’s examine the y values of the rational function y <br />
and the y values of the quotient function<br />
x 3<br />
y4x 16.<br />
To do this put the rational function in and the quotient function in . Next, set up your table by<br />
accessing and set the “Indpnt” variable to “Ask” so that you can input your x values into the table. Finally,<br />
select and enter the x values from the tables below, recording the corresponding y values.<br />
x<br />
What can you say about the values in relative to the values in as x ?<br />
2<br />
4x 4x3 c) Graph the rational function y <br />
x 3<br />
and the quotient function y4x16using your graphing calculator<br />
with the window settings below. Using two different colors, sketch the graphs of both in the box provided.<br />
USE WINDOW<br />
x:[-25,25] scale:5<br />
y:[-150,50] scale:25<br />
– 10<br />
– 20<br />
– 30<br />
x 10 20 30 40 50 100<br />
d) Where do the graphs of the rational function and the quotient function look alike? Where do they look different?<br />
– 40<br />
– 50<br />
-100
2<br />
4x 4x3 e) Notice that as the graph of y <br />
approaches the graph of the quotient function y4x16. x 3<br />
Since the quotient function’s degree is one, its graph is a line. When the graph of a rational function approaches a<br />
non-horizontal line as x , that line is called an oblique asymptote of the rational function. For our rational<br />
2<br />
4x 4x3 function, y = 4x 16 is an equation of the oblique asymptote. Knowing that y <br />
can be expressed as<br />
x 3<br />
2<br />
45<br />
4x 4x3 y4x16 , explain why the graph of y <br />
must approach the line y4x16as .<br />
x 3<br />
x 3<br />
(Hint: Think about what happens to 45<br />
x <br />
x <br />
as x<br />
.)<br />
x 3<br />
f) In part e above you explained why the graphs are close to each other as x . Give one possible reason why the<br />
graphs are not close to each other near 3<br />
<br />
x ?<br />
2<br />
4x 4x3 g) In part e above you learned that the rational function y <br />
has an oblique asymptote that was obtained by<br />
x 3<br />
dividing the numerator of the rational function by the denominator of the rational function. Also, you learned that an<br />
oblique asymptote of a rational function is a non-horizontal line, so it is defined by a degree 1 polynomial. What must<br />
the relationship between the degrees of the numerator and the denominator of a rational function be in order for the<br />
rational function to have an oblique asymptote? Explain.
3 2<br />
2x 7x 13x 18<br />
2. Now let’s consider the rational function y <br />
where the degree of the numerator is 2 greater than<br />
x 1<br />
the degree of the denominator.<br />
a) Use long division or synthetic division to determine the quotient polynomial Qx () and the remainder polynomial<br />
Rx () . Then write the function in the form of<br />
Qx ()<br />
___________________<br />
( )<br />
( ) .<br />
( )<br />
Rx<br />
y Q x<br />
Dx<br />
Rx ()<br />
y Q() x <br />
Dx () ________________________________<br />
Rx ( ) 22<br />
How does the degree of this quotient polynomial differ from the degree of the quotient polynomial in problem 1 a)?<br />
What could be the reason for this difference?<br />
b) As in 1b) examine the y values of the rational function and the quotient function y Q( x ) . Remember to let<br />
represent the rational function and the quotient function.<br />
x<br />
– 2<br />
– 10<br />
– 18<br />
x 2 10 18 26 34 42<br />
What can you say about the values of the rational function relative to the values of the quotient function as x ?<br />
– 26<br />
– 34<br />
– 42
c) Graph the rational function and the quotient function using your graphing calculator with the settings below. Using<br />
two different colors, sketch the graphs of both in the box provided.<br />
USE WINDOW<br />
x:[-10,10] scale:1<br />
y:[-40,100] scale:20<br />
3 2<br />
2x 7x 13x 18<br />
d) Notice that as x the graph of y <br />
x 1<br />
approaches the graph of the quotient function<br />
y Q( x ) . This quotient function is a non-<strong>linear</strong> asymptote of our rational function. This asymptote is non-<strong>linear</strong><br />
because the quotient polynomial’s degree is not 1. Knowing that<br />
y <br />
3 2<br />
22<br />
2x 7x 13x 18<br />
y Q( x)<br />
, explain why the graph of y <br />
x 1<br />
x 1<br />
x<br />
. (Hint: If needed, refer to the hint in 1e.)<br />
3. a) Given the rational function<br />
y <br />
Qx ()<br />
__________________________<br />
5 3<br />
x 5x4x 2<br />
x x12 Rx ()<br />
y Q() x <br />
Dx () ______________________________<br />
find the following:<br />
3 2<br />
2x 7x 13x 18<br />
x 1<br />
can be expressed as<br />
must approach the graph of y Q( x) as<br />
R( x) 120x 240<br />
The degree of the numerator is how much greater than the degree of the denominator? ___________<br />
What is the degree of the quotient polynomial? ___________<br />
Your answers to the previous two questions should be the same. Why should they be the same?
) Graph the rational function and the quotient function using your graphing calculator with the settings below. Sketch<br />
the graphs of both in the box provided.<br />
USE WINDOW<br />
x:[-8,8] scale:1<br />
y:[-500,500] scale:100<br />
c) What do the graphs in part b) suggest to you about the rational function and its corresponding quotient function?<br />
Explain.<br />
4. Given the rational function<br />
a) Use the above to determine the asymptote of the graph of<br />
5 3<br />
2x 58x 200x 4 3 2<br />
144<br />
y 2x 2x 56x 56x 144 .<br />
x1 x1<br />
(Note that the division has already been done for you)<br />
5 3<br />
2x 58x 200x<br />
y <br />
x 1<br />
as x .<br />
b) Verify your answer by graphing the rational function and your asymptote in the box provided.<br />
USE WINDOW<br />
x:[-6,6] scale:1<br />
y:[-200,400] scale:50<br />
5. Find the equation of the asymptote as x for each of the following rational functions. Graph each rational<br />
function and asymptote in a suitable window to verify your results.<br />
a)<br />
y <br />
4 3 2<br />
x x x x<br />
<br />
x 2<br />
2<br />
b)<br />
y <br />
2<br />
x x<br />
x 2<br />
c)<br />
y <br />
3 2<br />
x x x<br />
2 4 9<br />
2x3