3+1 formalism and bases of numerical relativity - LUTh ...
3+1 formalism and bases of numerical relativity - LUTh ...
3+1 formalism and bases of numerical relativity - LUTh ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
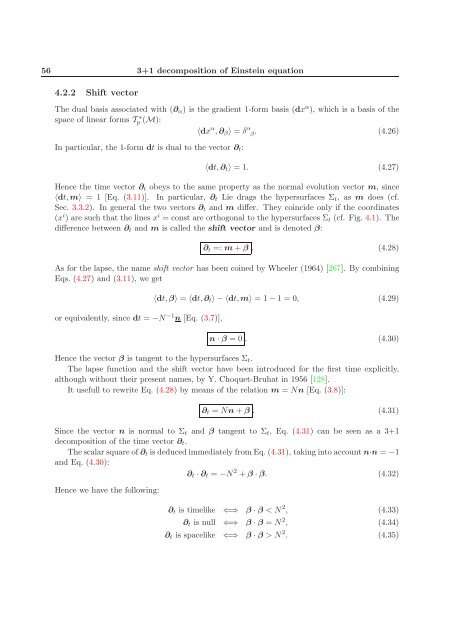
56 <strong>3+1</strong> decomposition <strong>of</strong> Einstein equation<br />
4.2.2 Shift vector<br />
The dual basis associated with (∂α) is the gradient 1-form basis (dxα ), which is a basis <strong>of</strong> the<br />
space <strong>of</strong> linear forms T ∗<br />
p (M):<br />
〈dx α ,∂β〉 = δ α β . (4.26)<br />
In particular, the 1-form dt is dual to the vector ∂t:<br />
〈dt,∂t〉 = 1. (4.27)<br />
Hence the time vector ∂t obeys to the same property as the normal evolution vector m, since<br />
〈dt,m〉 = 1 [Eq. (3.11)]. In particular, ∂t Lie drags the hypersurfaces Σt, as m does (cf.<br />
Sec. 3.3.2). In general the two vectors ∂t <strong>and</strong> m differ. They coincide only if the coordinates<br />
(x i ) are such that the lines x i = const are orthogonal to the hypersurfaces Σt (cf. Fig. 4.1). The<br />
difference between ∂t <strong>and</strong> m is called the shift vector <strong>and</strong> is denoted β:<br />
∂t =: m + β . (4.28)<br />
As for the lapse, the name shift vector has been coined by Wheeler (1964) [267]. By combining<br />
Eqs. (4.27) <strong>and</strong> (3.11), we get<br />
or equivalently, since dt = −N −1 n [Eq. (3.7)],<br />
〈dt,β〉 = 〈dt,∂t〉 − 〈dt,m〉 = 1 − 1 = 0, (4.29)<br />
n · β = 0 . (4.30)<br />
Hence the vector β is tangent to the hypersurfaces Σt.<br />
The lapse function <strong>and</strong> the shift vector have been introduced for the first time explicitly,<br />
although without their present names, by Y. Choquet-Bruhat in 1956 [128].<br />
It usefull to rewrite Eq. (4.28) by means <strong>of</strong> the relation m = Nn [Eq. (3.8)]:<br />
∂t = Nn + β . (4.31)<br />
Since the vector n is normal to Σt <strong>and</strong> β tangent to Σt, Eq. (4.31) can be seen as a <strong>3+1</strong><br />
decomposition <strong>of</strong> the time vector ∂t.<br />
The scalar square <strong>of</strong> ∂t is deduced immediately from Eq. (4.31), taking into account n·n = −1<br />
<strong>and</strong> Eq. (4.30):<br />
∂t · ∂t = −N 2 + β · β. (4.32)<br />
Hence we have the following:<br />
∂t is timelike ⇐⇒ β · β < N 2 , (4.33)<br />
∂t is null ⇐⇒ β · β = N 2 , (4.34)<br />
∂t is spacelike ⇐⇒ β · β > N 2 . (4.35)