3+1 formalism and bases of numerical relativity - LUTh ...

156 Choice of foliation and spatial coordinates
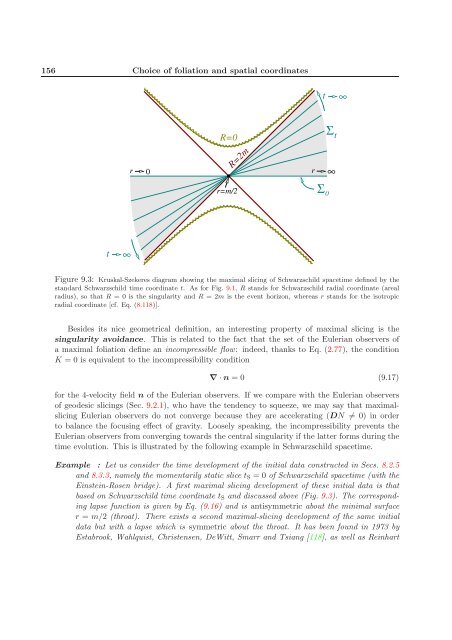
Figure 9.3: Kruskal-Szekeres diagram showing the maximal slicing of Schwarzschild spacetime defined by the
standard Schwarzschild time coordinate t. As for Fig. 9.1, R stands for Schwarzschild radial coordinate (areal
radius), so that R = 0 is the singularity and R = 2m is the event horizon, whereas r stands for the isotropic
radial coordinate [cf. Eq. (8.118)].
Besides its nice geometrical definition, an interesting property of maximal slicing is the
singularity avoidance. This is related to the fact that the set of the Eulerian observers of
a maximal foliation define an incompressible flow: indeed, thanks to Eq. (2.77), the condition
K = 0 is equivalent to the incompressibility condition
∇ · n = 0 (9.17)
for the 4-velocity field n of the Eulerian observers. If we compare with the Eulerian observers
of geodesic slicings (Sec. 9.2.1), who have the tendency to squeeze, we may say that maximalslicing
Eulerian observers do not converge because they are accelerating (DN = 0) in order
to balance the focusing effect of gravity. Loosely speaking, the incompressibility prevents the
Eulerian observers from converging towards the central singularity if the latter forms during the
time evolution. This is illustrated by the following example in Schwarzschild spacetime.
Example : Let us consider the time development of the initial data constructed in Secs. 8.2.5
and 8.3.3, namely the momentarily static slice tS = 0 of Schwarzschild spacetime (with the
Einstein-Rosen bridge). A first maximal slicing development of these initial data is that
based on Schwarzschild time coordinate tS and discussed above (Fig. 9.3). The corresponding
lapse function is given by Eq. (9.16) and is antisymmetric about the minimal surface
r = m/2 (throat). There exists a second maximal-slicing development of the same initial
data but with a lapse which is symmetric about the throat. It has been found in 1973 by
Estabrook, Wahlquist, Christensen, DeWitt, Smarr and Tsiang [118], as well as Reinhart