3+1 formalism and bases of numerical relativity - LUTh ...

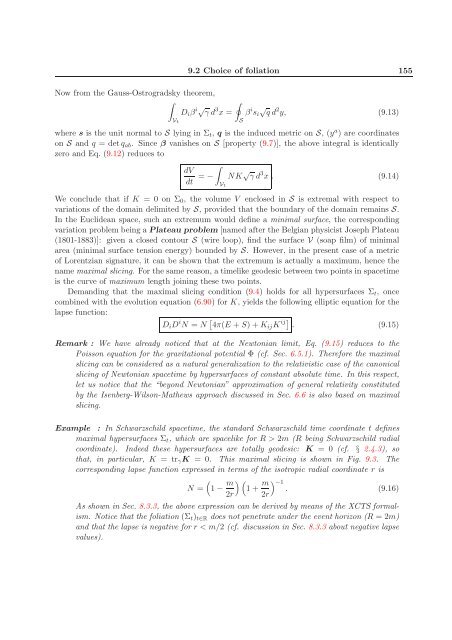
Now from the Gauss-Ostrogradsky theorem,
Diβ i√ γ d 3
x =
Vt
9.2 Choice of foliation 155
S
β i √ 2
si q d y, (9.13)
where s is the unit normal to S lying in Σt, q is the induced metric on S, (ya ) are coordinates
on S and q = detqab. Since β vanishes on S [property (9.7)], the above integral is identically
zero and Eq. (9.12) reduces to
dV
dt
= −
Vt
NK √ γ d 3 x . (9.14)
We conclude that if K = 0 on Σ0, the volume V enclosed in S is extremal with respect to
variations of the domain delimited by S, provided that the boundary of the domain remains S.
In the Euclidean space, such an extremum would define a minimal surface, the corresponding
variation problem being a Plateau problem [named after the Belgian physicist Joseph Plateau
(1801-1883)]: given a closed contour S (wire loop), find the surface V (soap film) of minimal
area (minimal surface tension energy) bounded by S. However, in the present case of a metric
of Lorentzian signature, it can be shown that the extremum is actually a maximum, hence the
name maximal slicing. For the same reason, a timelike geodesic between two points in spacetime
is the curve of maximum length joining these two points.
Demanding that the maximal slicing condition (9.4) holds for all hypersurfaces Σt, once
combined with the evolution equation (6.90) for K, yields the following elliptic equation for the
lapse function:
DiD i N = N 4π(E + S) + KijK ij . (9.15)
Remark : We have already noticed that at the Newtonian limit, Eq. (9.15) reduces to the
Poisson equation for the gravitational potential Φ (cf. Sec. 6.5.1). Therefore the maximal
slicing can be considered as a natural generalization to the relativistic case of the canonical
slicing of Newtonian spacetime by hypersurfaces of constant absolute time. In this respect,
let us notice that the “beyond Newtonian” approximation of general relativity constituted
by the Isenberg-Wilson-Mathews approach discussed in Sec. 6.6 is also based on maximal
slicing.
Example : In Schwarzschild spacetime, the standard Schwarzschild time coordinate t defines
maximal hypersurfaces Σt, which are spacelike for R > 2m (R being Schwarzschild radial
coordinate). Indeed these hypersurfaces are totally geodesic: K = 0 (cf. § 2.4.3), so
that, in particular, K = trγK = 0. This maximal slicing is shown in Fig. 9.3. The
corresponding lapse function expressed in terms of the isotropic radial coordinate r is
N = 1 − m
1 +
2r
m
−1 . (9.16)
2r
As shown in Sec. 8.3.3, the above expression can be derived by means of the XCTS formalism.
Notice that the foliation (Σt)t∈R does not penetrate under the event horizon (R = 2m)
and that the lapse is negative for r < m/2 (cf. discussion in Sec. 8.3.3 about negative lapse
values).