Gravitational Waves from Inspiralling Compact Binaries in ... - LUTH

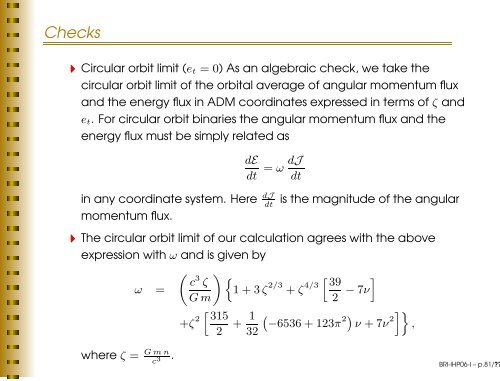
Checks
Circular orbit limit (et = 0) As an algebraic check, we take the
circular orbit limit of the orbital average of angular momentum flux
and the energy flux in ADM coordinates expressed in terms of ζ and
et. For circular orbit binaries the angular momentum flux and the
energy flux must be simply related as
dE
dt
in any coordinate system. Here dJ
dt
momentum flux.
= ω dJ
dt
is the magnitude of the angular
The circular orbit limit of our calculation agrees with the above
expression with ω and is given by
ω =
3
c ζ
1 + 3 ζ
G m
2/3 + ζ 4/3
39
− 7ν
2
+ζ 2
315 1 2
+ −6536 + 123π
2 32
ν + 7ν 2
where ζ =
G m n
c3 .
,
BRI-IHP06-I – p.81/??