FINDING N-TH ROOTS IN NILPOTENT GROUPS AND ...

FINDING N-TH ROOTS IN NILPOTENT GROUPS... 585
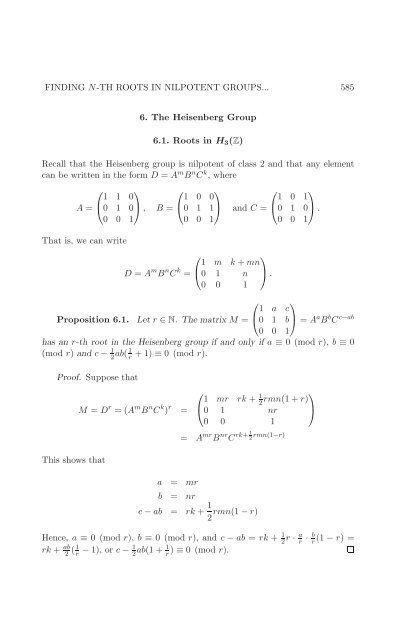
6. The Heisenberg Group
6.1. Roots in H3(Z)
Recall that the Heisenberg group is nilpotent of class 2 and that any element
can be written in the form D = A m B n C k , where
⎛ ⎞
1 1 0
⎛ ⎞
1 0 0
⎛ ⎞
1 0 1
A = ⎝0
1 0⎠,
B = ⎝0
1 1⎠
and C = ⎝0
1 0⎠
.
0 0 1 0 0 1
0 0 1
That is, we can write
D = A m B n C k =
⎛ ⎞
1 m k + mn
⎝0
1 n ⎠.
0 0 1
⎛ ⎞
1 a c
Proposition 6.1. Let r ∈ N. The matrix M = ⎝0
1 b⎠
= A
0 0 1
aBbC c−ab
has an r-th root in the Heisenberg group if and only if a ≡ 0 (mod r), b ≡ 0
+ 1) ≡ 0 (mod r).
(mod r) and c − 1
2ab(1 r
Proof. Suppose that
This shows that
M = D r = (A m B n C k ) r =
a = mr
⎛
1 mr rk +
⎝
1 ⎞
2rmn(1 + r)
0 1 nr ⎠
0 0 1
= A mr B nr C rk+1
2 rmn(1−r)
b = nr
c − ab = rk + 1
rmn(1 − r)
2
Hence, a ≡ 0 (mod r), b ≡ 0 (mod r), and c − ab = rk + 1
2
rk + ab
2 (1
r
− 1), or c − 1
2
1 ab(1 + r ) ≡ 0 (mod r).
r · a
r
b · r (1 − r) =