Hinton - The Fourth Dimension.pdf

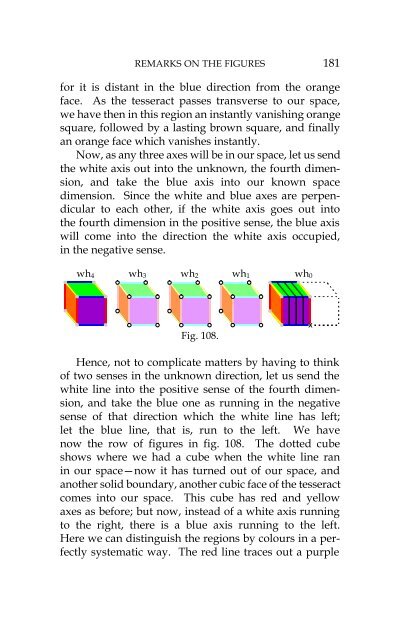
REMARKS ON THE FIGURES 181
for it is distant in the blue direction from the orange
face. As the tesseract passes transverse to our space,
we have then in this region an instantly vanishing orange
square, followed by a lasting brown square, and finally
an orange face which vanishes instantly.
Now, as any three axes will be in our space, let us send
the white axis out into the unknown, the fourth dimension,
and take the blue axis into our known space
dimension. Since the white and blue axes are perpendicular
to each other, if the white axis goes out into
the fourth dimension in the positive sense, the blue axis
will come into the direction the white axis occupied,
in the negative sense.
wh4 wh3 wh2 wh1 wh0
Fig. 108.
Hence, not to complicate matters by having to think
of two senses in the unknown direction, let us send the
white line into the positive sense of the fourth dimension,
and take the blue one as running in the negative
sense of that direction which the white line has left;
let the blue line, that is, run to the left. We have
now the row of figures in fig. 108. The dotted cube
shows where we had a cube when the white line ran
in our space—now it has turned out of our space, and
another solid boundary, another cubic face of the tesseract
comes into our space. This cube has red and yellow
axes as before; but now, instead of a white axis running
to the right, there is a blue axis running to the left.
Here we can distinguish the regions by colours in a perfectly
systematic way. The red line traces out a purple
x