6. Theorem of Ceva, Menelaus and Van Aubel.

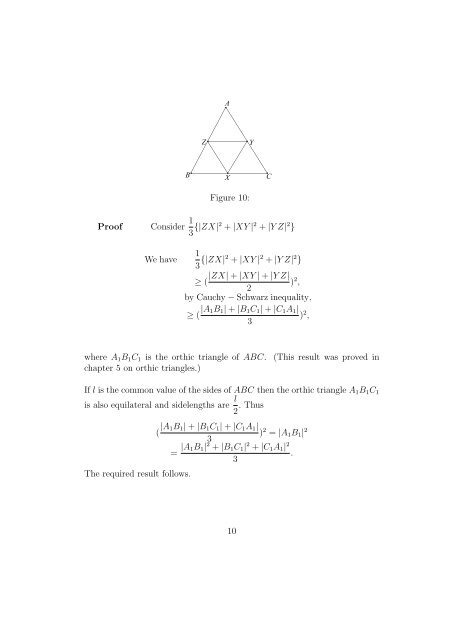
Figure 10:
Proof Consider 1
3 {|ZX|2 + |XY | 2 + |Y Z| 2 }
We have
1
3 {|ZX|2 + |XY | 2 + |Y Z| 2 }
|ZX| + |XY | + |Y Z|
≥ ( )
2
2 ,
by Cauchy − Schwarz inequality,
≥ ( |A1B1| + |B1C1| + |C1A1|
)
3
2 ,
where A1B1C1 is the orthic triangle of ABC. (This result was proved in
chapter 5 on orthic triangles.)
If l is the common value of the sides of ABC then the orthic triangle A1B1C1
is also equilateral and sidelengths are l
. Thus
2
The required result follows.
( |A1B1| + |B1C1| + |C1A1|
)
3
2 = |A1B1| 2
= |A1B1| 2 + |B1C1| 2 + |C1A1| 2
.
3
10