Etude des marchés d'assurance non-vie à l'aide d'équilibres de ...

tel-00703797, version 2 - 7 Jun 2012
3.5. Appendix
constrained equation reformulation was better. A method working for any general GNEP has
yet to be found and its convergence to be proved.
3.5 Appendix
3.5.1 Analysis
Nonsmooth analysis
Definition (locally Lipschitzian). G is locally Lipschitzian (on R n ) if ∀x ∈ R n , ∃U ∈ N (x), ∀y, z ∈
U, ∃kx > 0, ||G(y) − G(z)|| ≤ kx||y − z||.
From Clarke and Bessis (1999), the Rademacher theorem is
Theorem. Let f : R n ↦→ R be a locally Lipschitz function. Then f is almost everywhere
differentiable.
From (Clarke, 1990, Cor 2.2.4, Chap. 2), for a function f : R n ↦→ R locally Lipschitzian at
x, we have that the generalized gradient ∂f(y) is a singleton for all y ∈ B(x, ɛ) is equivalent
to f is C 1 on B(x, ɛ).
From (Clarke, 1990, Prop 2.6.2, Chap. 2), we have the following properties of the generalized
Jacobian.
Proposition. – ∂G(x) is a nonempty, convex, compact subset of R m×n , while ∂BG(x) is
nonempty and compact.
– ∂G is upper semicontinuous and closed at x and ∂BG is upper semicontinuous.
– ∂G(x) ⊂ ∂G1(x) × · · · × ∂Gm(x), where the right-hand side is a matrix set where the ith
row is the generalized gradient.
The term ∂G1(x) × · · · × ∂Gm(x) is sometimes denoted by ∂CG(x). But it is not Clarke’s
subdifferential, which seems to refer only to real-valued function, i.e. ∂G(x) = ∂CG(x).
From Theorem 2.3 of Qi and Sun (1993), we have the following equivalences
Proposition. – G is semismooth at x.
– ∀V ∈ ∂G(x + h), h → 0, V h − G ′ (x; h) = o(||h||).
– ∀x ∈ DG, G ′ (x + h; h) − G ′ (x; h) = o(||h||).
From Lemma 2.2 of Qi and Sun (1993) and Lemma 2.1 of Sun and Han (1997), we have
the following properties
Proposition. If G is semismooth at x, then d ↦→ G ′ (x; d) is a Lipschitz function; ∀h, ∃V ∈
G(x), V h = G ′ (x; h) and ∀h → 0, G(x + h) − G(x) − G ′ (x; h) = o(||h||) .
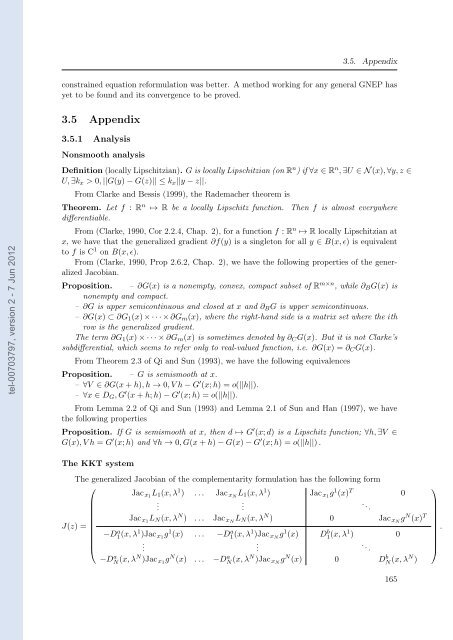
The KKT system
The generalized Jacobian of the complementarity formulation has the following form
⎛
⎜
J(z) = ⎜
⎝
Jac x1L1(x, λ1 ) . . . Jac xN L1(x, λ1 .
Jac x1
)
.
LN(x, λN ) . . . Jac xN LN(x, λN )
Jac x1g1 (x) T 0
. ..
0
Jac xN gN (x) T
−Da 1 (x, λ1 )Jac x1g1 (x) . . . −Da 1 (x, λ1 )Jac xN g1 .
(x)
.
Db 1 (x, λ1 )
. ..
0
−D a N (x, λN )Jac x1 gN (x) . . . −D a N (x, λN )Jac xN gN (x)
0 D b N (x, λN )
165
⎞
⎟ .
⎟
⎠