ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso

More documents

Recommendations

Info

68 4. Two-mode entanglement Eqs. (1.13,2.36,4.4) provide an explicit expression for any Sp as a function of µ and ∆. Such an expression can be exploited to study the behavior of ∆ as a function of the global purity µ, at fixed marginals and global Sp (from now on we will omit the explicit reference to fixed marginals). One has ∂µ ∂∆ Sp = − 2 R 2 ∂R ∂∆ Sp where we have defined the inverse participation ratio and the remaining quantities Np and Dp read Np(∆, R) = − Dp(∆, R) = = 2 R2 ∂Sp/∂∆| R ∂Sp/∂R| ∆ = 2 R2 Np(∆, R) , Dp(∆, R) (4.42) R ≡ 2 µ , (4.43) (R + 2 + 2 √ ∆ + R) p−1 − (R + 2 − 2 √ ∆ + R) p−1 √ ∆ − R (R − 2 + 2 √ ∆ − R) p−1 − (R − 2 − 2 √ ∆ − R) p−1 √ ∆ + R , ( √ ∆ + R + 1)(R + 2 + 2 √ ∆ + R) p−1 + ( √ ∆ + R − 1)(R + 2 − 2 √ ∆ + R) p−1 √ ∆ − R − ( √ ∆ − R + 1)(R − 2 − 2 √ ∆ − R) p−1 + ( √ ∆ − R − 1)(R − 2 + 2 √ ∆ − R) p−1 √ ∆ + R . (4.44) Now, it is easily shown that the ratio Np(∆, R)/Dp(∆, R) is increasing with increasing p and has a zero at p = 2 for any ∆, R; in particular, its absolute minimum (−1) is reached in the limit (∆ → 2, R → 2, p → 1). Thus the derivative Eq. (4.42) is negative for p < 2, null for p = 2 (in this case ∆ and S2 = 1 − µ are of course regarded as independent variables) and positive for p > 2. This implies that, for given marginals, keeping fixed any global Sp for p < 2 the minimum (maximum) value of ∆ corresponds to the maximum (minimum) value of the global purity µ. Instead, by keeping fixed any global Sp for p > 2 the minimum of ∆ is always attained at the minimum of the global purity µ. In other words, for fixed marginal entropies and global SV , the quantity ∆ decreases with increasing global purity, while for fixed marginal properties and global Sp (p > 2), ∆ increases with increasing µ. This observation allows to determine rather straightforwardly the states with extremal ∆. They are extremally entangled states because, for fixed global and marginal entropies, the logarithmic negativity of a state is determined only by the one remaining independent global symplectic invariant, represented by ∆ in our choice of parametrization. If, for the moment being, we neglect the fixed local purities, then the states with maximal ∆ are the states with minimal (maximal) µ for a given global Sp with p < 2 (p > 2) (see Sec. 2.3.2 and Fig. 2.1). As found in Sec. 2.3.2, such states are minimum-uncertainty two-mode states with mixedness concentrated in one quadrature. We have shown in Sec. 4.3.3.1 that they correspond to Gaussian least entangled mixed states (GLEMS) whose standard form is given by Eq. (4.39). As can be seen from Eq. (4.39), these states are consistent with any legitimate physical value of the local invariants µ1,2. We therefore conclude
4.3. Entanglement versus Entropic measures 69 that all Gaussian states with maximal ∆ for any fixed triple of values of global and marginal entropies are GLEMS. Viceversa one can show that all Gaussian states with minimal ∆ for any fixed triple of values of global and marginal entropies are Gaussian maximally entangled mixed states (GMEMS). This fact is immediately evident in the symmetric case because the extremal surface in the Sp vs. SL diagrams is always represented by symmetric two-mode squeezed thermal states (symmetric GMEMS). These states are characterized by a degenerate symplectic spectrum and encompass only equal choices of the local invariants: µ1 = µ2. 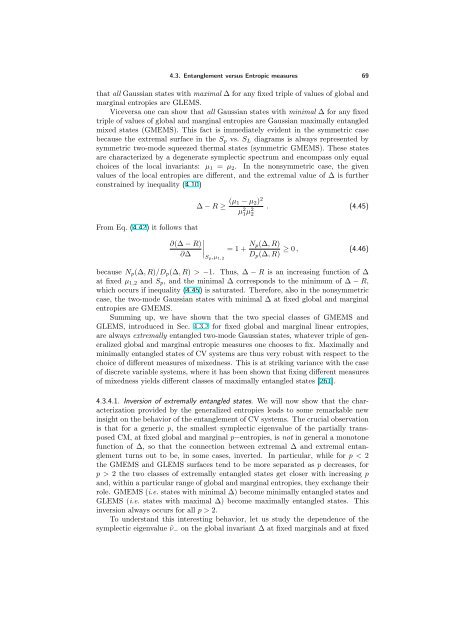In the nonsymmetric case, the given values of the local entropies are different, and the extremal value of ∆ is further constrained by inequality (4.10) From Eq. (4.42) it follows that ∂(∆ − R) ∂∆ ∆ − R ≥ (µ1 − µ2) 2 µ 2 1 µ2 2 Sp,µ1,2 . (4.45) = 1 + Np(∆, R) ≥ 0 , (4.46) Dp(∆, R) because Np(∆, R)/Dp(∆, R) > −1. Thus, ∆ − R is an increasing function of ∆ at fixed µ1,2 and Sp, and the minimal ∆ corresponds to the minimum of ∆ − R, which occurs if inequality (4.45) is saturated. Therefore, also in the nonsymmetric case, the two-mode Gaussian states with minimal ∆ at fixed global and marginal entropies are GMEMS. Summing up, we have shown that the two special classes of GMEMS and GLEMS, introduced in Sec. 4.3.3 for fixed global and marginal linear entropies, are always extremally entangled two-mode Gaussian states, whatever triple of generalized global and marginal entropic measures one chooses to fix. Maximally and minimally entangled states of CV systems are thus very robust with respect to the choice of different measures of mixedness. This is at striking variance with the case of discrete variable systems, where it has been shown that fixing different measures of mixedness yields different classes of maximally entangled states [261]. 4.3.4.1. Inversion of extremally entangled states. We will now show that the characterization provided by the generalized entropies leads to some remarkable new insight on the behavior of the entanglement of CV systems. The crucial observation is that for a generic p, the smallest symplectic eigenvalue of the partially transposed CM, at fixed global and marginal p−entropies, is not in general a monotone function of ∆, so that the connection between extremal ∆ and extremal entanglement turns out to be, in some cases, inverted. In particular, while for p < 2 the GMEMS and GLEMS surfaces tend to be more separated as p decreases, for p > 2 the two classes of extremally entangled states get closer with increasing p and, within a particular range of global and marginal entropies, they exchange their role. GMEMS (i.e. states with minimal ∆) become minimally entangled states and GLEMS (i.e. states with maximal ∆) become maximally entangled states. This inversion always occurs for all p > 2. To understand this interesting behavior, let us study the dependence of the symplectic eigenvalue ˜ν− on the global invariant ∆ at fixed marginals and at fixed
Page 1 and 2:
ENTANGLEMENT OF GAUSSIAN STATES Ger
Page 3:
Life’s Entanglement. CR Studio In
Page 7:
Abstract This Dissertation collects
Page 10 and 11:
x Contents 2.2.2.2. Symplectic repr
Page 12 and 13:
xii Contents 7.2.3. Tripartite enta
Page 14 and 15:
xiv Contents Chapter 13. Entangleme
Page 17 and 18:
Introduction About eighty years aft
Page 19 and 20:
Introduction 5 Gaussian states. Imp
Page 21:
Introduction 7 The companion Part V
Page 24 and 25:
10 1. Characterizing entanglement a
Page 26 and 27:
12 1. Characterizing entanglement w
Page 28 and 29:
14 1. Characterizing entanglement W
Page 30 and 31:
16 1. Characterizing entanglement F
Page 32 and 33: 18 1. Characterizing entanglement a
Page 34 and 35: 20 1. Characterizing entanglement i
Page 36 and 37: 22 1. Characterizing entanglement e
Page 38 and 39: 24 1. Characterizing entanglement n
Page 40 and 41: 26 1. Characterizing entanglement p
Page 43 and 44: CHAPTER 2 Gaussian states: structur
Page 45 and 46: 2.1. Introduction to continuous var
Page 47 and 48: 2.2. Mathematical description of Ga
Page 49 and 50: 2.2. Mathematical description of Ga
Page 51 and 52: 2.3. Degree of information encoded
Page 53 and 54: 2.3. Degree of information encoded
Page 55 and 56: 2.4. Standard forms of Gaussian cov
Page 63: Part II Bipartite entanglement of G
Page 66 and 67: 52 3. Characterizing entanglement o
Page 68 and 69: 54 3. Characterizing entanglement o
Page 71 and 72: CHAPTER 4 Two-mode entanglement Thi
Page 73 and 74: 4.2. Entanglement and symplectic ei
Page 75 and 76: 4.3. Entanglement versus Entropic m
Page 77 and 78: 1 0.75 0.5 SL1 0.25 0 (a) 4.3. Enta
Page 81: Μ Μ1Μ2 3 2 1 1 4.3. Entanglemen
Page 87 and 88: global generalized entropy S3 globa
Page 89 and 90: 4.4. Quantifying entanglement via p
Page 91 and 92: 4.4. Quantifying entanglement via p
Page 93 and 94: 4.5. Gaussian entanglement measures
Page 101 and 102: Ν p Σopt 1 0.8 0.6 0.4 0.2 0 4.5
Page 103 and 104: GEF GEF 4 3 2 1 0 4.6. Summary and
Page 105: 4.6. Summary and further remarks 91
Page 108 and 109: 94 5. Multimode entanglement under
Page 121: Part III Multipartite entanglement
Page 124 and 125: 110 6. Gaussian entanglement sharin
Page 132 and 133:
118 6. Gaussian entanglement sharin
Page 134 and 135:
120 6. Gaussian entanglement sharin
Page 136 and 137:
122 7. Tripartite entanglement in t
Page 138 and 139:
Page 140 and 141:
Page 142 and 143:
Page 144 and 145:
Page 146 and 147:
Page 148 and 149:
Page 150 and 151:
Page 152 and 153:
Page 154 and 155:
Page 156 and 157:
Page 158 and 159:
Page 160 and 161:
Page 162 and 163:
148 8. Unlimited promiscuity of mul
Page 164 and 165:
Page 166 and 167:
Page 168 and 169:
Page 171:
Part IV Quantum state engineering o
Page 174 and 175:
160 9. Two-mode Gaussian states in
Page 176 and 177:
Page 178 and 179:
Page 180 and 181:
Page 182 and 183:
Page 184 and 185:
Page 186 and 187:
Page 189 and 190:
CHAPTER 10 Tripartite and four-part
Page 191 and 192:
10.1. Optical production of three-m
Page 193 and 194:
(out) (in) TRITTER 10.1. Optical pr
Page 195 and 196:
10.2. How to produce and exploit un
Page 197 and 198:
CHAPTER 11 Efficient production of
Page 199 and 200:
11.2. Generic entanglement and stat
Page 201 and 202:
Page 203 and 204:
Page 205 and 206:
11.3. Economical state engineering
Page 207:
Part V Operational interpretation a
Page 210 and 211:
196 12. Multiparty quantum communic
Page 212 and 213:
Page 214 and 215:
Page 216 and 217:
Page 218 and 219:
Page 220 and 221:
Page 222 and 223:
Page 224 and 225:
Page 226 and 227:
Page 228 and 229:
Page 230 and 231:
Page 233 and 234:
CHAPTER 13 Entanglement in Gaussian
Page 235 and 236:
13.1. Gaussian valence bond states
Page 237 and 238:
Eq. (13.3) thus takes the form 13.1
Page 239 and 240:
13.2. Entanglement distribution in
Page 241 and 242:
s 20 17.5 15 12.5 10 7.5 5 2.5 13.2
Page 243 and 244:
13.3. Optical implementation of Gau
Page 245 and 246:
of the form Eq. (13.1), with 13.3.
Page 247 and 248:
13.4. Telecloning with Gaussian val
Page 249 and 250:
CHAPTER 14 Gaussian entanglement sh
Page 251 and 252:
14.1. Entanglement in non-inertial
Page 253 and 254:
14.2. Distributed Gaussian entangle
Page 255 and 256:
Page 257 and 258:
Page 259 and 260:
6 s 4 14.2. Distributed Gaussian en
Page 261 and 262:
2.5 0 0 5 10 IΣAR7.5 0 14.3. Distr
Page 263 and 264:
Page 265 and 266:
0 entanglement condition 14.3. Dist
Page 267 and 268:
Page 269 and 270:
Page 271 and 272:
4 3 a 2 1 14.4. Discussion and outl
Page 273:
Part VI Closing remarks Entanglemen
Page 276 and 277:
262 Conclusion and Outlook Gaussian
Page 278 and 279:
264 Conclusion and Outlook compass
Page 280 and 281:
266 A. Standard forms of pure Gauss
Page 282 and 283:
Page 284 and 285:
Page 286 and 287:
272 List of Publications [GA11] G.
Page 288 and 289:
274 Bibliography [23] C. H. Bennett
Page 290 and 291:
276 Bibliography [79] J. Eisert, C.
Page 292 and 293:
278 Bibliography [140] J. Laurat, T
Page 294 and 295:
280 Bibliography [198] T. Roscilde,
Page 296:
282 Bibliography [258] J. Von Neuma
show all

ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?