ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso

68 4. Two-mode entanglement
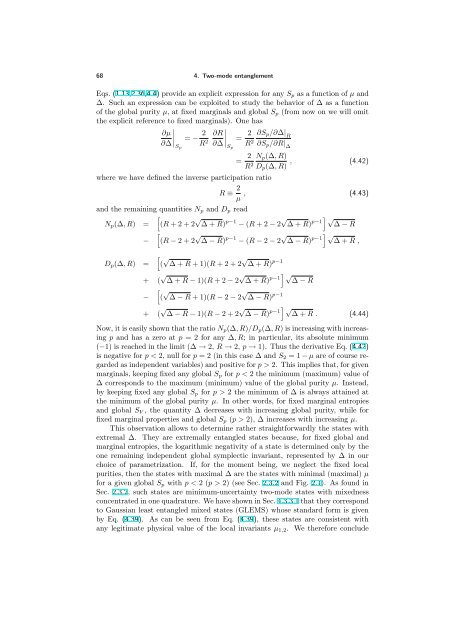
Eqs. (1.13,2.36,4.4) provide an explicit expression for any Sp as a function of µ and
∆. Such an expression can be exploited to study the behavior of ∆ as a function
of the global purity µ, at fixed marginals and global Sp (from now on we will omit
the explicit reference to fixed marginals). One has
∂µ
∂∆
Sp
= − 2
R 2
∂R
∂∆
Sp
where we have defined the inverse participation ratio
and the remaining quantities Np and Dp read
Np(∆, R) =
−
Dp(∆, R) =
= 2
R2 ∂Sp/∂∆| R
∂Sp/∂R| ∆
= 2
R2 Np(∆, R)
,
Dp(∆, R)
(4.42)
R ≡ 2
µ , (4.43)
(R + 2 + 2 √ ∆ + R) p−1 − (R + 2 − 2 √ ∆ + R) p−1 √
∆ − R
(R − 2 + 2 √ ∆ − R) p−1 − (R − 2 − 2 √ ∆ − R) p−1 √
∆ + R ,
( √ ∆ + R + 1)(R + 2 + 2 √ ∆ + R) p−1
+ ( √ ∆ + R − 1)(R + 2 − 2 √ ∆ + R) p−1 √
∆ − R
− ( √ ∆ − R + 1)(R − 2 − 2 √ ∆ − R) p−1
+ ( √ ∆ − R − 1)(R − 2 + 2 √ ∆ − R) p−1 √ ∆ + R . (4.44)
Now, it is easily shown that the ratio Np(∆, R)/Dp(∆, R) is increasing with increasing
p and has a zero at p = 2 for any ∆, R; in particular, its absolute minimum
(−1) is reached in the limit (∆ → 2, R → 2, p → 1). Thus the derivative Eq. (4.42)
is negative for p < 2, null for p = 2 (in this case ∆ and S2 = 1 − µ are of course regarded
as independent variables) and positive for p > 2. This implies that, for given
marginals, keeping fixed any global Sp for p < 2 the minimum (maximum) value of
∆ corresponds to the maximum (minimum) value of the global purity µ. Instead,
by keeping fixed any global Sp for p > 2 the minimum of ∆ is always attained at
the minimum of the global purity µ. In other words, for fixed marginal entropies
and global SV , the quantity ∆ decreases with increasing global purity, while for
fixed marginal properties and global Sp (p > 2), ∆ increases with increasing µ.
This observation allows to determine rather straightforwardly the states with
extremal ∆. They are extremally entangled states because, for fixed global and
marginal entropies, the logarithmic negativity of a state is determined only by the
one remaining independent global symplectic invariant, represented by ∆ in our
choice of parametrization. If, for the moment being, we neglect the fixed local
purities, then the states with maximal ∆ are the states with minimal (maximal) µ
for a given global Sp with p < 2 (p > 2) (see Sec. 2.3.2 and Fig. 2.1). As found in
Sec. 2.3.2, such states are minimum-uncertainty two-mode states with mixedness
concentrated in one quadrature. We have shown in Sec. 4.3.3.1 that they correspond
to Gaussian least entangled mixed states (GLEMS) whose standard form is given
by Eq. (4.39). As can be seen from Eq. (4.39), these states are consistent with
any legitimate physical value of the local invariants µ1,2. We therefore conclude