ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso

1
0.75
0.5
SL1
0.25
0
(a)
4.3. Entanglement versus Entropic measures 63
0.25
0.5
SL2 SL2
1
0.5
0
1
0.75
2
1.5
E
1
0.8
0.6
0.4
SL1
0.2
0
(b)
0.2
0.8
0.6
0.4
0.2
0
1
0.8
0.6
0.4
SL2 SL2
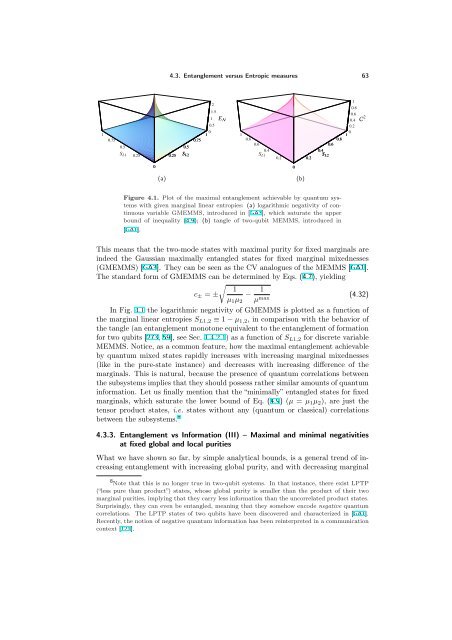
Figure 4.1. Plot of the maximal entanglement achievable by quantum systems
with given marginal linear entropies: (a) logarithmic negativity of continuous
variable GMEMMS, introduced in [GA3], which saturate the upper
bound of inequality (4.9); (b) tangle of two-qubit MEMMS, introduced in
[GA1].
This means that the two-mode states with maximal purity for fixed marginals are
indeed the Gaussian maximally entangled states for fixed marginal mixednesses
(GMEMMS) [GA3]. They can be seen as the CV analogues of the MEMMS [GA1].
The standard form of GMEMMS can be determined by Eqs. (4.7), yielding
1
c± = ± −
µ1µ2
1
µ max
(4.32)
In Fig. 4.1 the logarithmic negativity of GMEMMS is plotted as a function of
the marginal linear entropies SL1,2 ≡ 1 − µ1,2, in comparison with the behavior of
the tangle (an entanglement monotone equivalent to the entanglement of formation
for two qubits [273, 59], see Sec. 1.4.2.1) as a function of SL1,2 for discrete variable
MEMMS. Notice, as a common feature, how the maximal entanglement achievable
by quantum mixed states rapidly increases with increasing marginal mixednesses
(like in the pure-state instance) and decreases with increasing difference of the
marginals. This is natural, because the presence of quantum correlations between
the subsystems implies that they should possess rather similar amounts of quantum
information. Let us finally mention that the “minimally” entangled states for fixed
marginals, which saturate the lower bound of Eq. (4.9) (µ = µ1µ2), are just the
tensor product states, i.e. states without any (quantum or classical) correlations
between the subsystems. 8
4.3.3. Entanglement vs Information (III) – Maximal and minimal negativities
at fixed global and local purities
What we have shown so far, by simple analytical bounds, is a general trend of increasing
entanglement with increasing global purity, and with decreasing marginal
8 Note that this is no longer true in two-qubit systems. In that instance, there exist LPTP
(“less pure than product”) states, whose global purity is smaller than the product of their two
marginal purities, implying that they carry less information than the uncorrelated product states.
Surprisingly, they can even be entangled, meaning that they somehow encode negative quantum
correlations. The LPTP states of two qubits have been discovered and characterized in [GA1].
Recently, the notion of negative quantum information has been reinterpreted in a communication
context [121].
1
2