ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso
ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso
160 9. Two-mode Gaussian states in the lab generally mixed, Gaussian resources [GA9], which will be established in Chapter 12. Before turning to the operational interpretation of entanglement, we judge of interest to discuss, at this point of the Dissertation, the issue of providing efficient recipes to engineer, in the lab, the various classes of Gaussian states that we have singled out in the previous Parts for their remarkable entanglement properties. These optimal production schemes (among which we mention the one for all pure Gaussian states exhibiting generic entanglement [GA14], presented in Chapter 11) are of inherent usefulness to experimentalists who need to prepare entangled Gaussian states with minimal resources. Unless explicitly stated, we will always consider as preferred realistic setting for Gaussian state engineering that of quantum optics [65]. In this Chapter, we thus begin by first completing the analysis of Chapter 4 on the two special classes of two-mode “extremally” entangled Gaussian states that have arisen both in the negativity versus purity analysis, and in the comparison between Gaussian entanglement measures and negativities, namely GMEMS and GLEMS [GA3]. We discuss how both families of Gaussian states can be obtained experimentally, adding concreteness to the plethora of results previously presented on their entanglement characterization. After that, we move more deeply into the description of the experiment concerning production and optimization of entanglement in two-mode Gaussian states by optical means, reported in [GA8]. State engineering of Gaussian states of more than two modes will be addressed in the next two Chapters. 9.1. Schemes to realize extremally entangled states in experimental settings We discuss here how to obtain the two-mode states introduced in Sec. 4.3.3.1 in a practical setting. 9.1.1. GMEMS state engineering As we have seen, GMEMS are two-mode squeezed thermal states, whose general CM is described by Eqs. (2.54) and (4.29). A realistic instance giving rise to such states is provided by the dissipative evolution of an initially pure two-mode squeezed vacuum with CM Eq. (2.22). The latter may be created e.g. by mixing two independently squeezed beams (one in momentum and one in position, with equal squeezing parameter r) at a 50:50 beam-splitter B1,2(1/2), Eq. (2.26), as shown in Fig. 9.1. 25 Let us denote by σr the CM of a two mode squeezed vacuum with squeezing parameter r, Eq. (2.22), derived from Eqs. (4.29) with ν∓ = 1. The interaction of this initial state with a thermal noise results in the following dynamical map describing the time evolution of the CM σ(t) [210] σ(t) = e −Γt σr + (1 − e −Γt )σn1,n2 , (9.1) where Γ is the coupling to the noisy reservoir (equal to the inverse of the damping time) and σn1,n2 = ⊕ 2 i=1 ni2 is the CM of the thermal noise (see also Sec. 7.4.1.1). 25 See also Sec. 2.2.2. A more detailed discussion concerning the production of two-mode squeezed states is deferred to Sec. 9.2.
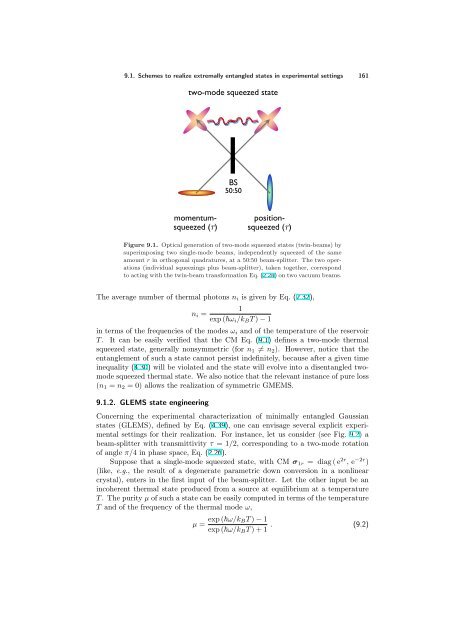
9.1. Schemes to realize extremally entangled states in experimental settings 161 two-mode squeezed state momentumsqueezed (r) BS 50:50 positionsqueezed (r) Figure 9.1. Optical generation of two-mode squeezed states (twin-beams) by superimposing two single-mode beams, independently squeezed of the same amount r in orthogonal quadratures, at a 50:50 beam-splitter. The two operations (individual squeezings plus beam-splitter), taken together, correspond to acting with the twin-beam transformation Eq. (2.28) on two vacuum beams. The average number of thermal photons ni is given by Eq. (2.32), ni = 1 exp (ωi/kBT ) − 1 in terms of the frequencies of the modes ωi and of the temperature of the reservoir T . It can be easily verified that the CM Eq. (9.1) defines a two-mode thermal squeezed state, generally nonsymmetric (for n1 = n2). However, notice that the entanglement of such a state cannot persist indefinitely, because after a given time inequality (4.30) will be violated and the state will evolve into a disentangled twomode squeezed thermal state. We also notice that the relevant instance of pure loss (n1 = n2 = 0) allows the realization of symmetric GMEMS. 9.1.2. GLEMS state engineering Concerning the experimental characterization of minimally entangled Gaussian states (GLEMS), defined by Eq. (4.39), one can envisage several explicit experimental settings for their realization. For instance, let us consider (see Fig. 9.2) a beam-splitter with transmittivity τ = 1/2, corresponding to a two-mode rotation of angle π/4 in phase space, Eq. (2.26). Suppose that a single-mode squeezed state, with CM σ1r = diag ( e 2r , e −2r ) (like, e.g., the result of a degenerate parametric down conversion in a nonlinear crystal), enters in the first input of the beam-splitter. Let the other input be an incoherent thermal state produced from a source at equilibrium at a temperature T . The purity µ of such a state can be easily computed in terms of the temperature T and of the frequency of the thermal mode ω, µ = exp (ω/kBT ) − 1 . (9.2) exp (ω/kBT ) + 1
- Page 124 and 125: 110 6. Gaussian entanglement sharin
- Page 126 and 127: 112 6. Gaussian entanglement sharin
- Page 128 and 129: 114 6. Gaussian entanglement sharin
- Page 130 and 131: 116 6. Gaussian entanglement sharin
- Page 132 and 133: 118 6. Gaussian entanglement sharin
- Page 134 and 135: 120 6. Gaussian entanglement sharin
- Page 136 and 137: 122 7. Tripartite entanglement in t
- Page 138 and 139: 124 7. Tripartite entanglement in t
- Page 140 and 141: 126 7. Tripartite entanglement in t
- Page 142 and 143: 128 7. Tripartite entanglement in t
- Page 144 and 145: 130 7. Tripartite entanglement in t
- Page 146 and 147: 132 7. Tripartite entanglement in t
- Page 148 and 149: 134 7. Tripartite entanglement in t
- Page 150 and 151: 136 7. Tripartite entanglement in t
- Page 152 and 153: 138 7. Tripartite entanglement in t
- Page 154 and 155: 140 7. Tripartite entanglement in t
- Page 156 and 157: 142 7. Tripartite entanglement in t
- Page 158 and 159: 144 7. Tripartite entanglement in t
- Page 160 and 161: 146 7. Tripartite entanglement in t
- Page 162 and 163: 148 8. Unlimited promiscuity of mul
- Page 164 and 165: 150 8. Unlimited promiscuity of mul
- Page 166 and 167: 152 8. Unlimited promiscuity of mul
- Page 168 and 169: 154 8. Unlimited promiscuity of mul
- Page 171: Part IV Quantum state engineering o
- Page 176 and 177: 162 9. Two-mode Gaussian states in
- Page 178 and 179: 164 9. Two-mode Gaussian states in
- Page 180 and 181: 166 9. Two-mode Gaussian states in
- Page 182 and 183: 168 9. Two-mode Gaussian states in
- Page 184 and 185: 170 9. Two-mode Gaussian states in
- Page 186 and 187: 172 9. Two-mode Gaussian states in
- Page 189 and 190: CHAPTER 10 Tripartite and four-part
- Page 191 and 192: 10.1. Optical production of three-m
- Page 193 and 194: (out) (in) TRITTER 10.1. Optical pr
- Page 195 and 196: 10.2. How to produce and exploit un
- Page 197 and 198: CHAPTER 11 Efficient production of
- Page 199 and 200: 11.2. Generic entanglement and stat
- Page 201 and 202: 11.2. Generic entanglement and stat
- Page 203 and 204: 11.2. Generic entanglement and stat
- Page 205 and 206: 11.3. Economical state engineering
- Page 207: Part V Operational interpretation a
- Page 210 and 211: 196 12. Multiparty quantum communic
- Page 212 and 213: 198 12. Multiparty quantum communic
- Page 214 and 215: 200 12. Multiparty quantum communic
- Page 216 and 217: 202 12. Multiparty quantum communic
- Page 218 and 219: 204 12. Multiparty quantum communic
- Page 220 and 221: 206 12. Multiparty quantum communic
- Page 222 and 223: 208 12. Multiparty quantum communic
9.1. Schemes to realize extremally entangled states in experimental settings 161<br />
two-mode squeezed state<br />
momentumsqueezed<br />
(r)<br />
BS<br />
50:50<br />
positionsqueezed<br />
(r)<br />
Figure 9.1. Optical generation of two-mode squeezed states (twin-beams) by<br />
superimposing two single-mode beams, independently squeezed of the same<br />
amount r in orthogonal quadratures, at a 50:50 beam-splitter. The two operations<br />
(individual squeezings plus beam-splitter), taken together, correspond<br />
to acting with the twin-beam transformation Eq. (2.28) on two vacuum beams.<br />
The average number of thermal photons ni is given by Eq. (2.32),<br />
ni =<br />
1<br />
exp (ωi/kBT ) − 1<br />
in terms of the frequencies of the modes ωi and of the temperature of the reservoir<br />
T . It can be easily verified that the CM Eq. (9.1) defines a two-mode thermal<br />
squeezed state, generally nonsymmetric (for n1 = n2). However, notice that the<br />
entanglement of such a state cannot persist indefinitely, because after a given time<br />
inequality (4.30) will be violated and the state will evolve into a disentangled twomode<br />
squeezed thermal state. We also notice that the relevant instance of pure loss<br />
(n1 = n2 = 0) allows the realization of symmetric GMEMS.<br />
9.1.2. GLEMS state engineering<br />
Concerning the experimental characterization of minimally entangled Gaussian<br />
states (GLEMS), defined by Eq. (4.39), one can envisage several explicit experimental<br />
settings for their realization. For instance, let us consider (see Fig. 9.2) a<br />
beam-splitter with transmittivity τ = 1/2, corresponding to a two-mode rotation<br />
of angle π/4 in phase space, Eq. (2.26).<br />
Suppose that a single-mode squeezed state, with CM σ1r = diag ( e 2r , e −2r )<br />
(like, e.g., the result of a degenerate parametric down conversion in a nonlinear<br />
crystal), enters in the first input of the beam-splitter. Let the other input be an<br />
incoherent thermal state produced from a source at equilibrium at a temperature<br />
T . The purity µ of such a state can be easily computed in terms of the temperature<br />
T and of the frequency of the thermal mode ω,<br />
µ = exp (ω/kBT ) − 1<br />
. (9.2)<br />
exp (ω/kBT ) + 1