ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso
ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso
ENTANGLEMENT OF GAUSSIAN STATES Gerardo Adesso
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
7.2. Distributed entanglement and genuine tripartite quantum correlations 131<br />
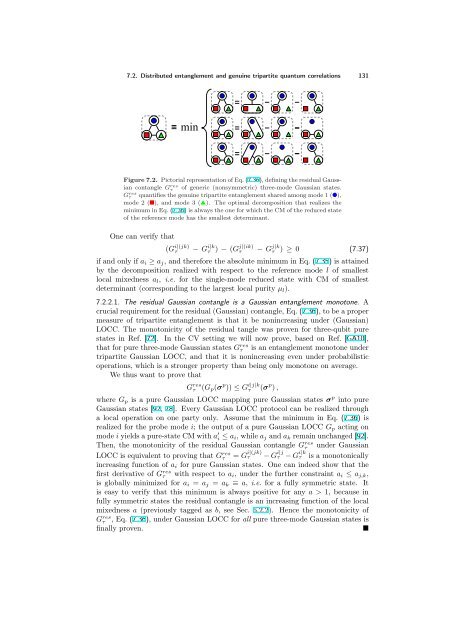
min<br />
Figure 7.2. Pictorial representation of Eq. (7.36), defining the residual Gaussian<br />
contangle Gres τ of generic (nonsymmetric) three-mode Gaussian states.<br />
Gres τ quantifies the genuine tripartite entanglement shared among mode 1 (●),<br />
mode 2 (■), and mode 3 (▲). The optimal decomposition that realizes the<br />
minimum in Eq. (7.36) is always the one for which the CM of the reduced state<br />
of the reference mode has the smallest determinant.<br />
One can verify that<br />
(G i|(jk)<br />
τ<br />
− G i|k<br />
τ ) − (G j|(ik)<br />
τ − G j|k<br />
τ ) ≥ 0 (7.37)<br />
if and only if ai ≥ aj, and therefore the absolute minimum in Eq. (7.35) is attained<br />
by the decomposition realized with respect to the reference mode l of smallest<br />
local mixedness al, i.e. for the single-mode reduced state with CM of smallest<br />
determinant (corresponding to the largest local purity µl).<br />
7.2.2.1. The residual Gaussian contangle is a Gaussian entanglement monotone. A<br />
crucial requirement for the residual (Gaussian) contangle, Eq. (7.36), to be a proper<br />
measure of tripartite entanglement is that it be nonincreasing under (Gaussian)<br />
LOCC. The monotonicity of the residual tangle was proven for three-qubit pure<br />
states in Ref. [72]. In the CV setting we will now prove, based on Ref. [GA10],<br />
that for pure three-mode Gaussian states Gres τ is an entanglement monotone under<br />
tripartite Gaussian LOCC, and that it is nonincreasing even under probabilistic<br />
operations, which is a stronger property than being only monotone on average.<br />
We thus want to prove that<br />
G res<br />
τ (Gp(σ p )) ≤ G i|j|k<br />
τ (σ p ) ,<br />
where Gp is a pure Gaussian LOCC mapping pure Gaussian states σp into pure<br />
Gaussian states [92, 78]. Every Gaussian LOCC protocol can be realized through<br />
a local operation on one party only. Assume that the minimum in Eq. (7.36) is<br />
realized for the probe mode i; the output of a pure Gaussian LOCC Gp acting on<br />
mode i yields a pure-state CM with a ′ i ≤ ai, while aj and ak remain unchanged [92].<br />
Then, the monotonicity of the residual Gaussian contangle Gres τ under Gaussian<br />
LOCC is equivalent to proving that Gres τ = G i|(jk)<br />
τ − G i|j<br />
τ − G i|k<br />
τ is a monotonically<br />
increasing function of ai for pure Gaussian states. One can indeed show that the<br />
first derivative of Gres τ with respect to ai, under the further constraint ai ≤ aj,k,<br />
is globally minimized for ai = aj = ak ≡ a, i.e. for a fully symmetric state. It<br />
is easy to verify that this minimum is always positive for any a > 1, because in<br />
fully symmetric states the residual contangle is an increasing function of the local<br />
mixedness a (previously tagged as b, see Sec. 6.2.2). Hence the monotonicity of<br />
Gres τ , Eq. (7.36), under Gaussian LOCC for all pure three-mode Gaussian states is<br />
finally proven.