Non-dispersive wave packets in periodically driven quantum systems

More documents

Recommendations

Info

506 A. Buchleitner et al. / Physics Reports 368 (2002) 409–547 |ψ (z)| 2 |ψ (z)| 2 0.06 0.04 0.02 0.00 0.01 0.00 0 500 1000 z 1500 2000 Fig. 43. Single Floquet state of the gravitational bouncer at phases !t = 0 (top) and !t = =2 (bottom), anchored to the 11:1 resonance island chain in classical phase space. Driving frequency ! =11 0:6027, n0 = 20 000, and =0:025. In the upper plot, among 11 individual wave packets which constitute the eigenstate, ve pairs (with partners moving in opposite directions) interfere at distances z¿0 from the mirror, whereas the 11th wave packet bounces o the wall and interferes with itself. At later times (bottom), the 11 wave packets are well separated in space. Observe the excellent agreement for small , with an almost exponential decrease of the splitting with √ , as expected from Eq. (256). However, for larger values of (nontheless still in the regime of predominantly regular classical motion) the splitting saturates and then starts to uctuate in an apparently random way. While the phenomenon has not been completely clari ed so far, we are inclined to attribute it to tiny avoided crossings with Floquet states localized is some other resonance islands (for a discussion of related phenomena see [160,161]). Comparison of the two panels of Fig. 42 additionally indicates that the region of values where avoided crossings become important increases in the semiclassical limit, and that Eq. (256) remains valid for small only. This is easily understood: in the semiclassical limit n0 →∞, the tunneling splitting decreases exponentially, while the density of states increases. Finally, Fig. 43 shows a Floquet eigenstate anchored to the s = 11 resonance island chain. It may be thought of as a linear combination of 11 non-dispersive wave packets which, at a given time, may interfere with each other, or, at another time (bottom panel), are spatially well separated. For a Helium atom bouncing o an atom mirror in the earth’s gravitational eld (alike the setting in [158]), the z values for such a state reach 5 mm. This non-dispersive wave packet is thus a macroscopic object composed of 11 individual components keeping a well-de ned phase coherence. 5.3. The s =2 resonance in atomic hydrogen under linearly polarized driving Let us now return to the hydrogen atom driven by LP microwaves. The highly non-linear character of the Coulomb interaction favours non-dispersive wave packets anchored to the s:1 resonance island, since the Fourier components Vs of the coupling between the atom and the microwave decay slowly 30 30 The very same behavior characterizes the gravitational bouncer discussed in the previous section. For the bouncer the slow inverse square dependence of Vs on s is due to a hard collision with the oscillating surface. For the Coulomb problem, the singularity at the origin is even stronger.
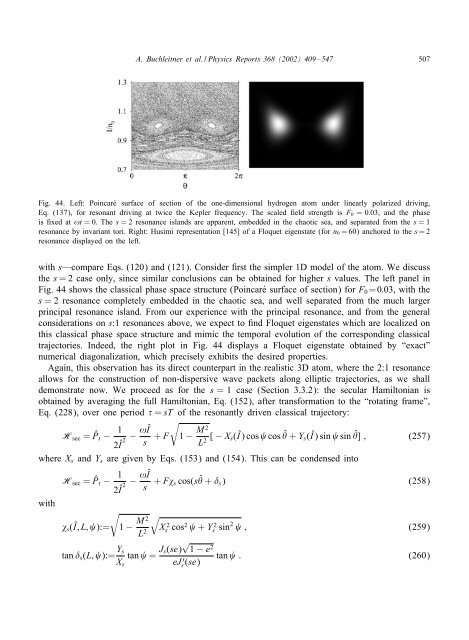
A. Buchleitner et al. / Physics Reports 368 (2002) 409–547 507 Fig. 44. Left: Poincare surface of section of the one-dimensional hydrogen atom under linearly polarized driving, Eq. (137), for resonant driving at twice the Kepler frequency. The scaled eld strength is F0 =0:03, and the phase is xed at !t =0: The s = 2 resonance islands are apparent, embedded in the chaotic sea, and separated from the s =1 resonance by invariant tori. Right: Husimi representation [145] of a Floquet eigenstate (for n0 = 60) anchored to the s =2 resonance displayed on the left. with s—compare Eqs. (120) and (121). Consider rst the simpler 1D model of the atom. We discuss the s = 2 case only, since similar conclusions can be obtained for higher s values. The left panel in Fig. 44 shows the classical phase space structure (Poincare surface of section) for F0 =0:03, with the s = 2 resonance completely embedded in the chaotic sea, and well separated from the much larger principal resonance island. From our experience with the principal resonance, and from the general considerations on s:1 resonances above, we expect to nd Floquet eigenstates which are localized on this classical phase space structure and mimic the temporal evolution of the corresponding classical trajectories. Indeed, the right plot in Fig. 44 displays a Floquet eigenstate obtained by “exact” numerical diagonalization, which precisely exhibits the desired properties. Again, this observation has its direct counterpart in the realistic 3D atom, where the 2:1 resonance allows for the construction of non-dispersive wave packets along elliptic trajectories, as we shall demonstrate now. We proceed as for the s = 1 case (Section 3.3.2): the secular Hamiltonian is obtained by averaging the full Hamiltonian, Eq. (152), after transformation to the “rotating frame”, Eq. (228), over one period = sT of the resonantly driven classical trajectory: Hsec = ˆPt − 1 !Î − + F 1 − 2 2Î s M 2 L 2 [ − Xs(Î) cos cos ˆ + Ys(Î) sin sin ˆ ] ; (257) where Xs and Ys are given by Eqs. (153) and (154). This can be condensed into with Hsec = ˆPt − 1 !Î − 2 2Î s + F s cos(s ˆ + s) (258) s(Î; L; ):= 1 − tan s(L; ):= Ys Xs M 2 L2 X 2 s cos2 + Y 2 s sin 2 ; (259) tan = Js(se) √ 1 − e 2 eJ ′ s(se) tan : (260)
Page 1 and 2:
Abstract Physics Reports 368 (2002)
Page 3 and 4:
A. Buchleitner et al. / Physics Rep
Page 5 and 6:
Page 7 and 8:
Page 9 and 10:
Page 11 and 12:
Page 13 and 14:
Page 15 and 16:
Page 17 and 18:
Page 19 and 20:
Page 21 and 22:
Page 23 and 24:
Page 25 and 26:
Page 27 and 28:
Page 29 and 30:
Page 31 and 32:
Page 33 and 34:
Page 35 and 36:
Page 37 and 38:
Page 39 and 40:
Page 41 and 42:
Page 43 and 44:
→ ; → ; A. Buchleitner et al. /
Page 45 and 46:
Page 47 and 48: A. Buchleitner et al. / Physics Rep
Page 97: |ψ (z)| 2 |ψ (z)| 2 0.3 0.2 0.1 0
Page 113 and 114: γ /∆ σ/∆ 10 -1 10 -2 10 -3 0.
Page 139: A. Buchleitner et al. / Physics Rep
show all

Non-dispersive wave packets in periodically driven quantum systems

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?