Non-dispersive wave packets in periodically driven quantum systems

More documents

Recommendations

Info

416 A. Buchleitner et al. / Physics Reports 368 (2002) 409–547 one obtains 9W (˜r; ˜p; t) = {H; W (˜r; ˜p; t)} ; (22) 9t where {:;:} denotes the classical Poisson bracket [3]: 3 {f; g} = i=1:::f 9f 9ri 9g 9pi − 9f 9pi 9g 9ri : (23) Eq. (22) is nothing but the classical Liouville equation [3] which describes the classical evolution of a phase space density. Hence, in the “semiclassical limit” ˝ → 0, the Wigner density evolves classically. Corrections of higher power in ˝ can be calculated systematically. For example, the next order is ˝3H 3W=24 in Eq. (20), and generates terms which contain third-order derivatives (in either position and=or momentum) of the Hamiltonian. Therefore, for a Hamiltonian of maximal degree two in position and=or momentum, all higher-order terms in Eq. (20) vanish and the Wigner distribution follows the classical evolution for an arbitrary initial phase space density, and for arbitrarily long times. The harmonic oscillator is an example of such a system [2,16], in agreement with our discussion of Eq. (15) above. Now, once again, why does a wave packet spread? At rst sight, it could be thought that this is due to the higher-order terms in Eq. (20), and thus of quantum origin. This is not true and spreading of a wave packet has a purely classical origin, as illustrated by the following example. Let us consider a one-dimensional, free particle (i.e., no potential), initially described by a Gaussian wave function with average position z0, average momentum p0 ¿ 0, and spatial width : 1 (z; t =0)= √ exp i 1=4 p0z 2 (z − z0) − ˝ 2 2 : (24) The corresponding Wigner distribution is a Gaussian in phase space: W (z; p; t =0)= 1 ˝ exp 2 2 (z − z0) (p − p0) − − 2 2 ˝2 : (25) As the Hamiltonian is quadratic in the momentum, without potential, this distribution evolves precisely alike the equivalent classical phase space density. Hence, the part of the wave packet with p¿p0 will evolve with a larger velocity than the part with p¡p0. Even if both parts are initially localized close to z0, the contribution of di erent velocity classes implies that their distance will increase without bound at long times. The wave packet will therefore spread, because the various classical trajectories have di erent velocities. Spreading is thus a completely classical phenomenon. This can be seen quantitatively by calculating the exact quantum evolution. One obtains W (z; p; t)= 1 ˝ exp − (z − z0 − pt=m) 2 2 − 2 (p − p0) 2 3 We choose here the most common de nition of the Poisson bracket. Note, however, that some authors [14,15] use the opposite sign. ˝ 2 (26)
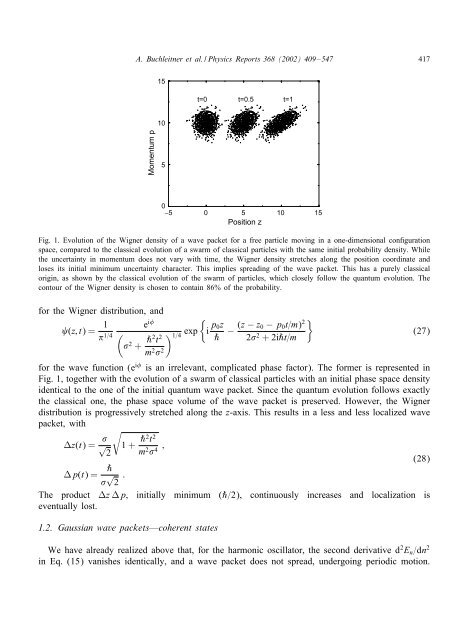
A. Buchleitner et al. / Physics Reports 368 (2002) 409–547 417 Momentum p 15 10 5 t=0 t=0.5 t=1 0 −5 0 5 Position z 10 15 Fig. 1. Evolution of the Wigner density of a wave packet for a free particle moving in a one-dimensional con guration space, compared to the classical evolution of a swarm of classical particles with the same initial probability density. While the uncertainty in momentum does not vary with time, the Wigner density stretches along the position coordinate and loses its initial minimum uncertainty character. This implies spreading of the wave packet. This has a purely classical origin, as shown by the classical evolution of the swarm of particles, which closely follow the quantum evolution. The contour of the Wigner density is chosen to contain 86% of the probability. for the Wigner distribution, and (z; t)= 1 1=4 ei ˝ 2 + 2t2 exp i 1=4 m2 2 p0z ˝ − (z − z0 − p0t=m) 2 2 2 +2i˝t=m for the wave function (ei is an irrelevant, complicated phase factor). The former is represented in Fig. 1, together with the evolution of a swarm of classical particles with an initial phase space density identical to the one of the initial quantum wave packet. Since the quantum evolution follows exactly the classical one, the phase space volume of the wave packet is preserved. However, the Wigner distribution is progressively stretched along the z-axis. This results in a less and less localized wave packet, with z(t)=√ 2 p(t)= ˝ √ 2 : 1+ ˝2t2 ; m2 4 The product z p, initially minimum (˝=2), continuously increases and localization is eventually lost. 1.2. Gaussian wave packets—coherent states We have already realized above that, for the harmonic oscillator, the second derivative d 2 En=dn 2 in Eq. (15) vanishes identically, and a wave packet does not spread, undergoing periodic motion. (27) (28)
Page 1 and 2: Abstract Physics Reports 368 (2002)
Page 3 and 4: A. Buchleitner et al. / Physics Rep
Page 7: A. Buchleitner et al. / Physics Rep
Page 43 and 44: → ; → ; A. Buchleitner et al. /
Page 59 and 60:
A. Buchleitner et al. / Physics Rep
Page 61 and 62:
Page 63 and 64:
Page 65 and 66:
Page 67 and 68:
Page 69 and 70:
Page 71 and 72:
Page 73 and 74:
Page 75 and 76:
Page 77 and 78:
Page 79 and 80:
Page 81 and 82:
Page 83 and 84:
Page 85 and 86:
Page 87 and 88:
Page 89 and 90:
Page 91 and 92:
Page 93 and 94:
Page 95 and 96:
Page 97 and 98:
|ψ (z)| 2 |ψ (z)| 2 0.3 0.2 0.1 0
Page 99 and 100:
Page 101 and 102:
Page 103 and 104:
Page 105 and 106:
Page 107 and 108:
Page 109 and 110:
Page 111 and 112:
Page 113 and 114:
γ /∆ σ/∆ 10 -1 10 -2 10 -3 0.
Page 115 and 116:
Page 117 and 118:
Page 119 and 120:
Page 121 and 122:
Page 123 and 124:
Page 125 and 126:
Page 127 and 128:
Page 129 and 130:
Page 131 and 132:
Page 133 and 134:
Page 135 and 136:
Page 137 and 138:
Page 139:
show all

Non-dispersive wave packets in periodically driven quantum systems

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?