Non-dispersive wave packets in periodically driven quantum systems

More documents

Recommendations

Info

422 A. Buchleitner et al. / Physics Reports 368 (2002) 409–547 ∆z ∆p (hbar unit) 100 10 1 Heisenberg limit Revival 0 10 20 Time (Kepler periods) Fig. 4. Time evolution of the uncertainty product z p (in units of ˝) of the wave packet shown in Fig. 3. Starting out from minimum uncertainty, z p ˝=2 (the Heisenberg limit, Eq. (1)), the wave packet exhibits some transient spreading on the time-scale of a Kepler period Trecurrence, thus re ecting the classical motion (compare top left of Fig. 3), collapses on a time-scale of few Kepler cycles (manifest in the damping of the oscillations of z p during the rst ve classical periods), shows a fractional revival around t 10 × Trecurrence, and a full revival at t 20 × Trecurrence. Note that, nontheless, even at the full revival the contrast of the oscillations of the uncertainty product is reduced as compared to the initial stage of the evolution, as a consequence of higher-order corrections which are neglected in Eq. (15). Fig. 5. Time evolution of a wave packet launched along a circular Kepler trajectory, with the same Gaussian weights cn, Eq. (11), as employed for the one-dimensional example displayed in Fig. 3, i.e., centered around the principal quantum number n0 = 60. Since the relative phases accumulated during the time evolution only depend on n0—see Eq. (13)—we observe precisely the same behavior as in the one-dimensional case: classical propagation at short times (top), followed by spreading and collapse (middle), and revival (bottom). The snapshots of the wave function are taken at times (in units of Trecurrence) t = 0 (top left), t =0:5 (top right), t = 1 (middle left), t =12:5 (middle right), and t =19:45 (bottom). The cube size is 10 000 Bohr radii, centered on the nucleus (marked with a cross). The radius of the circular wave-packet trajectory equals approx. 3600 Bohr radii. Surprisingly, it is classical mechanics which provides us with a possible solution. Indeed, as discussed above, a quantum wave packet spreads exactly as the corresponding swarm of classical particles. Hence, dispersion can be overcome if all classical trajectories behave similarly in
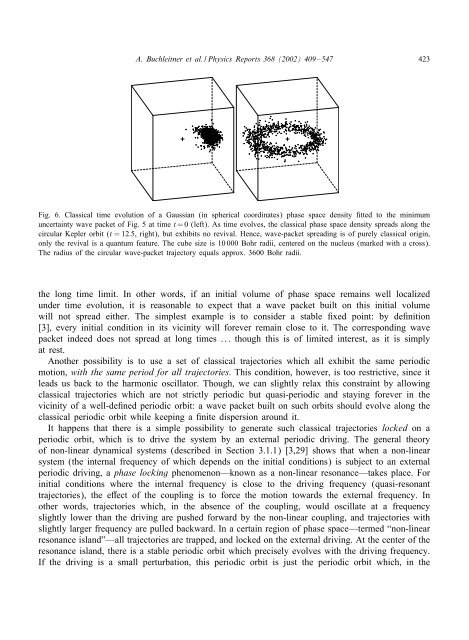
A. Buchleitner et al. / Physics Reports 368 (2002) 409–547 423 Fig. 6. Classical time evolution of a Gaussian (in spherical coordinates) phase space density tted to the minimum uncertainty wave packet of Fig. 5 at time t = 0 (left). As time evolves, the classical phase space density spreads along the circular Kepler orbit (t =12:5, right), but exhibits no revival. Hence, wave-packet spreading is of purely classical origin, only the revival is a quantum feature. The cube size is 10 000 Bohr radii, centered on the nucleus (marked with a cross). The radius of the circular wave-packet trajectory equals approx. 3600 Bohr radii. the long time limit. In other words, if an initial volume of phase space remains well localized under time evolution, it is reasonable to expect that a wave packet built on this initial volume will not spread either. The simplest example is to consider a stable xed point: by de nition [3], every initial condition in its vicinity will forever remain close to it. The corresponding wave packet indeed does not spread at long times ::: though this is of limited interest, as it is simply at rest. Another possibility is to use a set of classical trajectories which all exhibit the same periodic motion, with the same period for all trajectories. This condition, however, is too restrictive, since it leads us back to the harmonic oscillator. Though, we can slightly relax this constraint by allowing classical trajectories which are not strictly periodic but quasi-periodic and staying forever in the vicinity of a well-de ned periodic orbit: a wave packet built on such orbits should evolve along the classical periodic orbit while keeping a nite dispersion around it. It happens that there is a simple possibility to generate such classical trajectories locked on a periodic orbit, which is to drive the system by an external periodic driving. The general theory of non-linear dynamical systems (described in Section 3.1.1) [3,29] shows that when a non-linear system (the internal frequency of which depends on the initial conditions) is subject to an external periodic driving, a phase locking phenomenon—known as a non-linear resonance—takes place. For initial conditions where the internal frequency is close to the driving frequency (quasi-resonant trajectories), the e ect of the coupling is to force the motion towards the external frequency. In other words, trajectories which, in the absence of the coupling, would oscillate at a frequency slightly lower than the driving are pushed forward by the non-linear coupling, and trajectories with slightly larger frequency are pulled backward. In a certain region of phase space—termed “non-linear resonance island”—all trajectories are trapped, and locked on the external driving. At the center of the resonance island, there is a stable periodic orbit which precisely evolves with the driving frequency. If the driving is a small perturbation, this periodic orbit is just the periodic orbit which, in the
Page 1 and 2: Abstract Physics Reports 368 (2002)
Page 3 and 4: A. Buchleitner et al. / Physics Rep
Page 13: A. Buchleitner et al. / Physics Rep
Page 43 and 44: → ; → ; A. Buchleitner et al. /
Page 65 and 66:
A. Buchleitner et al. / Physics Rep
Page 67 and 68:
Page 69 and 70:
Page 71 and 72:
Page 73 and 74:
Page 75 and 76:
Page 77 and 78:
Page 79 and 80:
Page 81 and 82:
Page 83 and 84:
Page 85 and 86:
Page 87 and 88:
Page 89 and 90:
Page 91 and 92:
Page 93 and 94:
Page 95 and 96:
Page 97 and 98:
|ψ (z)| 2 |ψ (z)| 2 0.3 0.2 0.1 0
Page 99 and 100:
Page 101 and 102:
Page 103 and 104:
Page 105 and 106:
Page 107 and 108:
Page 109 and 110:
Page 111 and 112:
Page 113 and 114:
γ /∆ σ/∆ 10 -1 10 -2 10 -3 0.
Page 115 and 116:
Page 117 and 118:
Page 119 and 120:
Page 121 and 122:
Page 123 and 124:
Page 125 and 126:
Page 127 and 128:
Page 129 and 130:
Page 131 and 132:
Page 133 and 134:
Page 135 and 136:
Page 137 and 138:
Page 139:
show all

Non-dispersive wave packets in periodically driven quantum systems

Create successful ePaper yourself

Delete template?

Save as template?