Non-dispersive wave packets in periodically driven quantum systems

More documents

Recommendations

Info

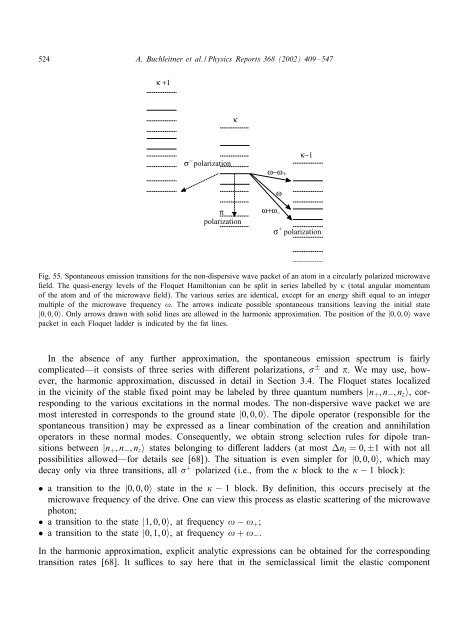
524 A. Buchleitner et al. / Physics Reports 368 (2002) 409–547 κ +1 σ − polarization κ π polarization ω−ω + ω ω+ω − κ−1 σ + polarization Fig. 55. Spontaneous emission transitions for the non-dispersive wave packet of an atom in a circularly polarized microwave eld. The quasi-energy levels of the Floquet Hamiltonian can be split in series labelled by (total angular momentum of the atom and of the microwave eld). The various series are identical, except for an energy shift equal to an integer multiple of the microwave frequency !. The arrows indicate possible spontaneous transitions leaving the initial state |0; 0; 0〉. Only arrows drawn with solid lines are allowed in the harmonic approximation. The position of the |0; 0; 0〉 wave packet in each Floquet ladder is indicated by the fat lines. In the absence of any further approximation, the spontaneous emission spectrum is fairly complicated—it consists of three series with di erent polarizations, ± and . We may use, however, the harmonic approximation, discussed in detail in Section 3.4. The Floquet states localized in the vicinity of the stable xed point may be labeled by three quantum numbers |n+;n−;nz〉, corresponding to the various excitations in the normal modes. The non-dispersive wave packet we are most interested in corresponds to the ground state |0; 0; 0〉. The dipole operator (responsible for the spontaneous transition) may be expressed as a linear combination of the creation and annihilation operators in these normal modes. Consequently, we obtain strong selection rules for dipole transitions between |n+;n−;nz〉 states belonging to di erent ladders (at most ni =0; ±1 with not all possibilities allowed—for details see [68]). The situation is even simpler for |0; 0; 0〉, which may decay only via three transitions, all + polarized (i.e., from the block to the − 1 block): • a transition to the |0; 0; 0〉 state in the − 1 block. By de nition, this occurs precisely at the microwave frequency of the drive. One can view this process as elastic scattering of the microwave photon; • a transition to the state |1; 0; 0〉, at frequency ! − !+; • a transition to the state |0; 1; 0〉, at frequency ! + !−. In the harmonic approximation, explicit analytic expressions can be obtained for the corresponding transition rates [68]. It su ces to say here that in the semiclassical limit the elastic component
A. Buchleitner et al. / Physics Reports 368 (2002) 409–547 525 becomes dominant, since its intensity scales as ! 5=3 , while the intensities of the other two components are proportional to ! 2 , i.e., are typically weaker by a factor n0 = ! −1=3 . This implies that the non-dispersive wave packet decays exclusively (in the harmonic approximation) to its immediate neighbor states, emitting a photon with frequency in the microwave range, comparable to the driving frequency. Direct decay to the atomic |n =1;L= M =0〉 ground state or to weakly excited states of the system is forbidden by the selection rules of the dipole operator. This is easily understood: the CP non-dispersive ground state wave packet |0; 0; 0〉 is built essentially from states with large angular momentum (of the order of n0), and as it can lose only one unit of angular momentum per spontaneous emission event, it can decay only to similar states. When the harmonic approximation breaks down, additional lines may appear, but, for the same reason, in the microwave range only. Another important observation is that the inelastic component at ! + !− is by far stronger than the one at ! − !+. This is entirely due to the cubic power of the transition frequency entering the expression for the rate (271). Note the sign di erence, due to the sign di erence between ± modes in the harmonic Hamiltonian, Eq. (197). In the semiclassical limit ! = n −3 0 → 0, the decay is dominated by the elastic component, and the total decay rate is [68] = 2 3 ! 5=3 q −2=3 3 ; (272) when, multiplied by the energy ! of the spontaneous photon, gives the energy loss due to spontaneous emission: dE dt = 2 3 ! 8=3q−2=3 = 3 2 3 ! 4 |xeq| 2 ; 3 (273) where we used Eq. (186). This is nothing but the result obtained from classical electrodynamics [190] for a point charge moving on a circular orbit of radius |xeq| with frequency !. Since the charge loses energy, it cannot survive on a circular orbit and would eventually fall onto the nucleus following a spiral trajectory. This model stimulated Bohr’s original formulation of quantum mechanics. Let us notice that the non-dispersive wave packet is the rst physical realization of the Bohr model. There is no net loss of energy since, in our case, the electron is driven by the microwave eld and an emission at frequency ! occurs in fact as an elastic scattering of a microwave photon. Thus the non-dispersive wave packet is a cure of the long-lasting Bohr paradox. Fig. 56 shows the square of the dipole matrix elements connecting the non-dispersive wave packet, for n0 = 60 (i.e., microwave frequency ! =1=603 ) and scaled microwave eld F0 =0:04446, to other Floquet states with lower energy. These are the results of an exact numerical diagonalization of the full Floquet Hamiltonian. They are presented as a stick spectrum because the widths of the important lines are very narrow on the scale of the gure, which is given as a function of the energy di erence between the initial and the nal state, that is the frequency of the scattered photon. Thus, this gure shows the lines that could be observed when recording the photoabsorption of a weak microwave probe eld. As expected, there is a dominant line at the frequency ! of the microwave, and two other lines at frequencies !−!− and !+!+ with comparable intensities, while all other lines are at least 10 times weaker. This means that the harmonic approximation works here very well; its predictions, indicated by the crosses in the gure, are in good quantitative agreement with the exact result (apart from tiny shifts recognizable in the gure, which correspond to the mismatch between the exact
Page 1 and 2:
Abstract Physics Reports 368 (2002)
Page 3 and 4:
A. Buchleitner et al. / Physics Rep
Page 5 and 6:
Page 7 and 8:
Page 9 and 10:
Page 11 and 12:
Page 13 and 14:
Page 15 and 16:
Page 17 and 18:
Page 19 and 20:
Page 21 and 22:
Page 23 and 24:
Page 25 and 26:
Page 27 and 28:
Page 29 and 30:
Page 31 and 32:
Page 33 and 34:
Page 35 and 36:
Page 37 and 38:
Page 39 and 40:
Page 41 and 42:
Page 43 and 44:
→ ; → ; A. Buchleitner et al. /
Page 45 and 46:
Page 47 and 48:
Page 49 and 50:
Page 51 and 52:
Page 53 and 54:
Page 55 and 56:
Page 57 and 58:
Page 59 and 60:
Page 61 and 62:
Page 63 and 64:
Page 65 and 66: A. Buchleitner et al. / Physics Rep
Page 97 and 98: |ψ (z)| 2 |ψ (z)| 2 0.3 0.2 0.1 0
Page 113 and 114: γ /∆ σ/∆ 10 -1 10 -2 10 -3 0.
Page 115: A. Buchleitner et al. / Physics Rep
Page 139: A. Buchleitner et al. / Physics Rep
show all

Non-dispersive wave packets in periodically driven quantum systems

Create successful ePaper yourself

Delete template?

Save as template?