Math 411: Honours Complex Variables - University of Alberta

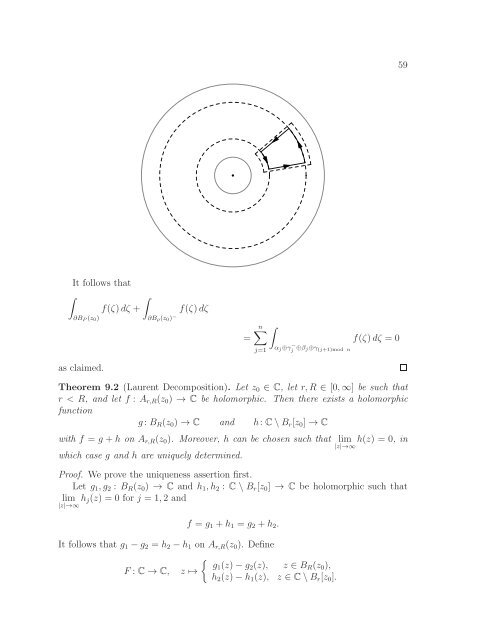
It follows that
� �
f(ζ)dζ +
∂BP(z0)
as claimed.
∂Bρ(z0) −
f(ζ)dζ
=
n�
�
j=1
αj⊕γ −
j ⊕βj⊕γ (j+1)mod n
f(ζ)dζ = 0
Theorem 9.2 (Laurent Decomposition). Let z0 ∈ C, let r,R ∈ [0,∞] be such that
r < R, and let f : Ar,R(z0) → C be holomorphic. Then there exists a holomorphic
function
g: BR(z0) → C and h: C\Br[z0] → C
with f = g +h on Ar,R(z0). Moreover, h can be chosen such that lim h(z) = 0, in
|z|→∞
which case g and h are uniquely determined.
Proof. We prove the uniqueness assertion first.
Let g1,g2 : BR(z0) → C and h1,h2 : C \ Br[z0] → C be holomorphic such that
lim hj(z) = 0 for j = 1,2 and
|z|→∞
f = g1 +h1 = g2 +h2.
It follows that g1 −g2 = h2 −h1 on Ar,R(z0). Define
F : C → C, z ↦→
� g1(z)−g2(z), z ∈ BR(z0),
h2(z)−h1(z), z ∈ C\Br[z0].
59